Exam 14: Multiple Integrals
Exam 1: Preliminaries143 Questions
Exam 2: Limits and Continuity125 Questions
Exam 3: Differentiation150 Questions
Exam 4: Applications of the Derivative143 Questions
Exam 5: Integration154 Questions
Exam 6: Applications of the Definite Integral113 Questions
Exam 7: Integration Techniques95 Questions
Exam 8: First-Order Differential Equations72 Questions
Exam 9: Infinite Series111 Questions
Exam 10: Parametric Equations and Polar Coordinates129 Questions
Exam 11: Vectors and the Geometry of Space107 Questions
Exam 12: Vector-Valued Functions103 Questions
Exam 13: Functions of Several Variables and Partial Differentiation112 Questions
Exam 14: Multiple Integrals92 Questions
Exam 15: Vector Calculus67 Questions
Exam 16: Second Order Differential Equations38 Questions
Select questions type
Set up the triple integral 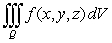 in cylindrical coordinates where Q is the solid above
in cylindrical coordinates where Q is the solid above 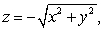 below
below 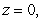 and inside
and inside 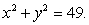
Free
(Multiple Choice)
4.9/5  (36)
(36)
Correct Answer:
C
Use polar coordinates to evaluate 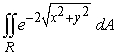 where R is the disk
where R is the disk 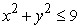 .
. 
Free
(Multiple Choice)
4.7/5  (40)
(40)
Correct Answer:
A
Set up and evaluate the integral 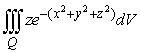 where Q is the region inside a sphere centered at (0,0,0) and with a radius of 7.
where Q is the region inside a sphere centered at (0,0,0) and with a radius of 7.
(Multiple Choice)
4.7/5  (34)
(34)
Calculate the mass of an object with density 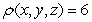 and bounded by
and bounded by 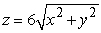 and
and 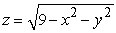
(Multiple Choice)
4.9/5  (45)
(45)
Compute the volume of the solid bounded by 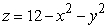 ,
,  and the coordinate planes.
and the coordinate planes.
(Multiple Choice)
4.8/5  (30)
(30)
Find the center of mass of the solid with density 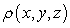 and the given shape.
and the given shape. 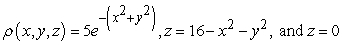 ,
,
(Multiple Choice)
4.8/5  (25)
(25)
Use a transformation to evaluate the double integral over the region R which is bounded by 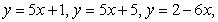 and
and 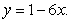

(Multiple Choice)
4.8/5  (36)
(36)
Using an appropriate coordinate system, evaluate the integral  where Q is the region inside the cylinder
where Q is the region inside the cylinder 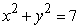 and between the planes
and between the planes  and
and  .
.
(Multiple Choice)
4.8/5  (32)
(32)
Convert the equation  in spherical coordinates to an equation in rectangular coordinates.
in spherical coordinates to an equation in rectangular coordinates.
(Multiple Choice)
4.8/5  (43)
(43)
Approximate the double integral.  , where R is bounded by x = 0, x = 3, y = 0, and y = x + 2
, where R is bounded by x = 0, x = 3, y = 0, and y = x + 2
(Multiple Choice)
4.9/5  (37)
(37)
Use an appropriate coordinate system to compute the volume of the solid below  and above
and above 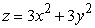 .
.
(Multiple Choice)
4.9/5  (28)
(28)
Which of the following could represent the triple integral 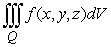 in cylindrical coordinates where Q is the region bounded below by
in cylindrical coordinates where Q is the region bounded below by  and above by
and above by  ?
?
(Multiple Choice)
4.9/5  (33)
(33)
Showing 1 - 20 of 92
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)