Exam 7: Goal Programming and Multiple Objective Optimization
Exam 1: Introduction to Modeling and Decision Analysis78 Questions
Exam 2: Introduction to Optimization and Linear Programming76 Questions
Exam 3: Modeling and Solving Lp Problems in a Spreadsheet93 Questions
Exam 4: Sensitivity Analysis and the Simplex Method76 Questions
Exam 5: Network Modeling92 Questions
Exam 6: Integer Linear Programming102 Questions
Exam 7: Goal Programming and Multiple Objective Optimization81 Questions
Exam 8: Nonlinear Programming Evolutionary Optimization87 Questions
Exam 9: Regression Analysis93 Questions
Exam 10: Data Mining125 Questions
Exam 11: Time Series Forecasting125 Questions
Exam 12: Introduction to Simulation Using Analytic Solver Platform85 Questions
Exam 13: Queuing Theory97 Questions
Exam 14: Decision Analysis125 Questions
Exam 15: Project Management Online82 Questions
Select questions type
What is the meaning of the ti term in this objective function for a goal programming problem? 
(Multiple Choice)
4.8/5  (38)
(38)
A company makes 2 products A and B from 2 resources. The products have the following resource requirements and produce the accompanying profits. The available quantity of resources is also shown in the table. 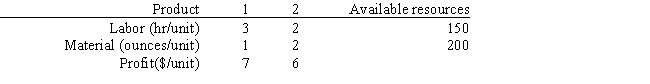 Management has developed the following set of goals
Management has developed the following set of goals  Based on this GP formulation of the problem and the associated optimal integer solution what values should go in cells B2:F16 of the following Excel spreadsheet?
Based on this GP formulation of the problem and the associated optimal integer solution what values should go in cells B2:F16 of the following Excel spreadsheet? 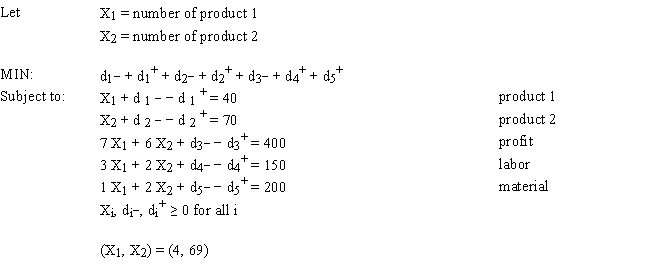
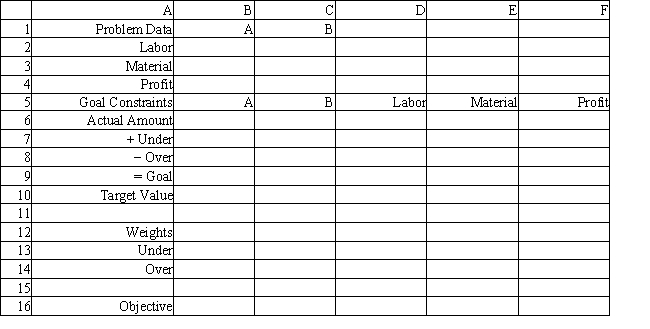
(Essay)
4.7/5  (23)
(23)
Suppose that profit and human variables are assigned the weight of zero. Then the "triple bottom line" approach reduces to:
(Multiple Choice)
4.9/5  (40)
(40)
A manager wants to ensure that he does not exceed his budget by more than $1000 in a goal programming problem. If the budget constraint is the third constraint in the goal programming problem which of the following formulas will best ensure that the manager's objective is met?
(Multiple Choice)
4.7/5  (31)
(31)
Suppose that X1 equals 4. What are the values for d1+ and d1− in the following constraint?
X1 + d1−− d1+ = 8
(Multiple Choice)
4.8/5  (28)
(28)
A company wants to purchase large and small delivery trucks. The company wants to purchase about 10 large and 15 small trucks. Each large truck costs $30,000 and has a 10 ton capacity. Each small truck costs $20,000 and has a 7 ton capacity. The company wants to have about 200 tons of capacity and spend about $600,000.
Formulate a goal programming model of this problem.
(Essay)
4.8/5  (39)
(39)
Exhibit 7.2
The following questions are based on the problem below.
An investor has $150,000 to invest in investments A and B. Investment A requires a $10,000 minimum investment, pays a return of 12% and has a risk factor of .50. Investment B requires a $15,000 minimum investment, pays a return of 10% and has a risk factor of .20. The investor wants to maximize the return while minimizing the risk of the portfolio. The following multi-objective linear programming (MOLP) has been solved in Excel. 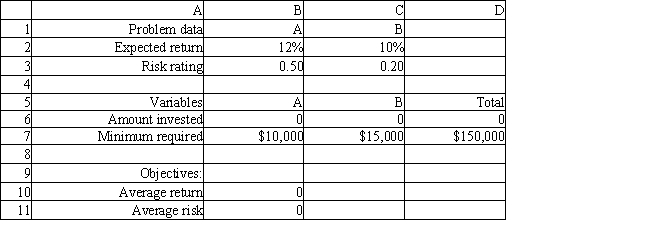 -Refer to Exhibit 7.2. Which cell(s) is(are) the target cells in this model?
-Refer to Exhibit 7.2. Which cell(s) is(are) the target cells in this model?
(Multiple Choice)
4.7/5  (38)
(38)
A company makes 2 products A and B from 2 resources, labor and material. The products have the following resource requirements and produce the accompanying profits. The available quantity of resources is also shown in the table. 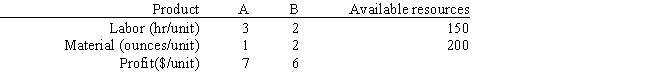 Management has developed the following set of goals
Management has developed the following set of goals  Formulate a goal programming model of this problem.
Formulate a goal programming model of this problem.
(Essay)
4.8/5  (47)
(47)
Exhibit 7.3
The following questions are based on the problem below.
An investor has $150,000 to invest in investments A and B. Investment A requires a $10,000 minimum investment, pays a return of 12% and has a risk factor of .50. Investment B requires a $15,000 minimum investment, pays a return of 10% and has a risk factor of .20. The investor wants to maximize the return while minimizing the risk of the portfolio. The following minimax formulation of the problem has been solved in Excel.  -Refer to Exhibit 7.3. Which value should the investor change, and in what direction, if he wants to reduce the risk of the portfolio?
-Refer to Exhibit 7.3. Which value should the investor change, and in what direction, if he wants to reduce the risk of the portfolio?
(Multiple Choice)
4.9/5  (42)
(42)
Trade-offs in goal programming can be made by modifying the weights to satisfy the priorities of a decision maker
(True/False)
4.8/5  (33)
(33)
One major advantage of goal programming (GP) is that the technique:
(Multiple Choice)
5.0/5  (27)
(27)
An investor wants to invest $50,000 in two mutual funds, A and B. The rates of return, risks and minimum investment requirements for each fund are: 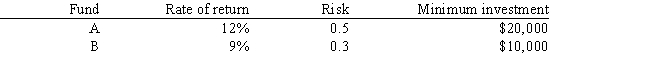 Note that a low Risk rating means a less risky investment. The investor can invest to maximize the expected rate of return or minimize risk. Any money beyond the minimum investment requirements can be invested in either fund.
The following is the MOLP formulation for this problem:
Note that a low Risk rating means a less risky investment. The investor can invest to maximize the expected rate of return or minimize risk. Any money beyond the minimum investment requirements can be invested in either fund.
The following is the MOLP formulation for this problem:  The solution for the second LP is (X1, X2) = (20,000, 30,000).
What formulas should go in cells B2:D11 of the spreadsheet? NOTE: Formulas are not required in all of these cells.
The solution for the second LP is (X1, X2) = (20,000, 30,000).
What formulas should go in cells B2:D11 of the spreadsheet? NOTE: Formulas are not required in all of these cells. 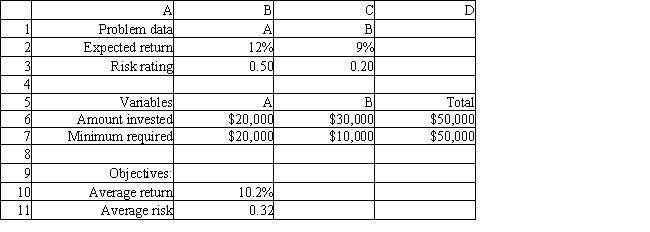
(Essay)
4.9/5  (26)
(26)
Exhibit 7.4
The following questions are based on the problem below.
Robert Gardner runs a small, local-only delivery service. His fleet consists of three smaller panel trucks. He recently accepted a contract to deliver 12 shipping boxes of goods for delivery to 12 different customers. The box weights are: 210, 160, 320, 90, 110, 70, 410, 260, 170, 240, 80 and 180 for boxes 1 through 12, respectively. Since each truck differs each truck has different load capacities as given below: 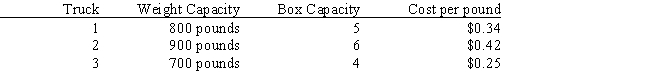 Robert would like each truck equally loaded, both in terms of number of boxes and in terms of total weight, while minimizing his shipping costs. Assume a cost of $50 per item for trucks carrying extra boxes and $0.10 per pound cost for trucks carrying less weight.
The following integer goal programming formulation applies to his problem.
Y1 = weight loaded in truck 1; Y2 = weight loaded in truck 2; Y3 = weight loaded in truck 3;
Xi,j = 0 if truck i not loaded with box j; 1 if truck i loaded with box j.
Robert would like each truck equally loaded, both in terms of number of boxes and in terms of total weight, while minimizing his shipping costs. Assume a cost of $50 per item for trucks carrying extra boxes and $0.10 per pound cost for trucks carrying less weight.
The following integer goal programming formulation applies to his problem.
Y1 = weight loaded in truck 1; Y2 = weight loaded in truck 2; Y3 = weight loaded in truck 3;
Xi,j = 0 if truck i not loaded with box j; 1 if truck i loaded with box j.  Given the following spreadsheet solution of this integer goal programming formulation, answer the following questions.
Given the following spreadsheet solution of this integer goal programming formulation, answer the following questions. 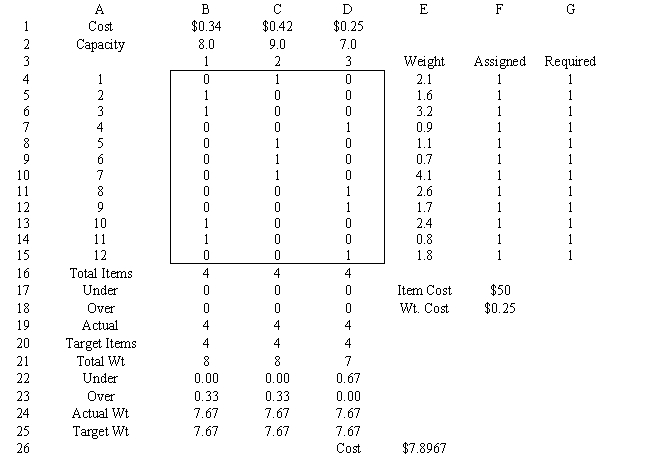 -Refer to Exhibit 7.4. Given the solution indicated in the spreadsheet, which trucks, if any, are under an equal weight amount, and which trucks are over an equal weight amount?
-Refer to Exhibit 7.4. Given the solution indicated in the spreadsheet, which trucks, if any, are under an equal weight amount, and which trucks are over an equal weight amount?
(Essay)
4.8/5  (30)
(30)
Exhibit 7.4
The following questions are based on the problem below.
Robert Gardner runs a small, local-only delivery service. His fleet consists of three smaller panel trucks. He recently accepted a contract to deliver 12 shipping boxes of goods for delivery to 12 different customers. The box weights are: 210, 160, 320, 90, 110, 70, 410, 260, 170, 240, 80 and 180 for boxes 1 through 12, respectively. Since each truck differs each truck has different load capacities as given below: 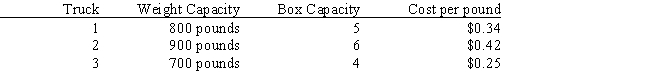 Robert would like each truck equally loaded, both in terms of number of boxes and in terms of total weight, while minimizing his shipping costs. Assume a cost of $50 per item for trucks carrying extra boxes and $0.10 per pound cost for trucks carrying less weight.
The following integer goal programming formulation applies to his problem.
Y1 = weight loaded in truck 1; Y2 = weight loaded in truck 2; Y3 = weight loaded in truck 3;
Xi,j = 0 if truck i not loaded with box j; 1 if truck i loaded with box j.
Robert would like each truck equally loaded, both in terms of number of boxes and in terms of total weight, while minimizing his shipping costs. Assume a cost of $50 per item for trucks carrying extra boxes and $0.10 per pound cost for trucks carrying less weight.
The following integer goal programming formulation applies to his problem.
Y1 = weight loaded in truck 1; Y2 = weight loaded in truck 2; Y3 = weight loaded in truck 3;
Xi,j = 0 if truck i not loaded with box j; 1 if truck i loaded with box j.  Given the following spreadsheet solution of this integer goal programming formulation, answer the following questions.
Given the following spreadsheet solution of this integer goal programming formulation, answer the following questions.  -Refer to Exhibit 7.4. The spreadsheet model has scaled all the weights from pounds into 100s pounds. How does this scaling effect the solution obtained using the Analytic Solver Platform (ASP)?
-Refer to Exhibit 7.4. The spreadsheet model has scaled all the weights from pounds into 100s pounds. How does this scaling effect the solution obtained using the Analytic Solver Platform (ASP)?
(Essay)
4.8/5  (37)
(37)
An investor wants to invest $50,000 in two mutual funds, A and B. The rates of return, risks and minimum investment requirements for each fund are: 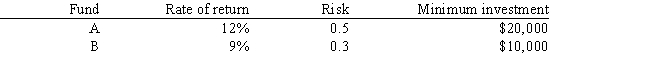 Note that a low Risk rating means a less risky investment. The investor wants to maximize the expected rate of return while minimizing his risk. Any money beyond the minimum investment requirements can be invested in either fund. The investor has found that the maximum possible expected rate of return is 11.4% and the minimum possible risk is 0.32.
Formulate a goal programming model with a MINIMAX objective function.
Note that a low Risk rating means a less risky investment. The investor wants to maximize the expected rate of return while minimizing his risk. Any money beyond the minimum investment requirements can be invested in either fund. The investor has found that the maximum possible expected rate of return is 11.4% and the minimum possible risk is 0.32.
Formulate a goal programming model with a MINIMAX objective function.
(Essay)
4.8/5  (27)
(27)
In the goal programming problem, the weights, wi, attached to deviational variables must decay exponentially
(True/False)
4.9/5  (39)
(39)
Showing 21 - 40 of 81
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)