Exam 13: Multiple Regression
Exam 1: Data and Statistics84 Questions
Exam 2: Descriptive Statistics: Tabular and Graphical Presentations116 Questions
Exam 3: Descriptive Statistics: Numerical Measures130 Questions
Exam 4: Introduction to Probability127 Questions
Exam 5: Discrete Probability Distributions146 Questions
Exam 6: Continuous Probability Distributions138 Questions
Exam 7: Sampling and Sampling Distributions123 Questions
Exam 8: Interval Estimation111 Questions
Exam 9: Hypothesis Tests117 Questions
Exam 10: Comparisons Involving Means, Experimental Design, and Analysis of Variance184 Questions
Exam 11: Comparisons Involving Proportions and a Test of Independence117 Questions
Exam 12: Simple Linear Regression107 Questions
Exam 13: Multiple Regression111 Questions
Exam 14: Statistical Methods for Quality Control72 Questions
Exam 15: Time Series Analysis and Forecastng75 Questions
Select questions type
Exhibit 13-3
In a regression model involving 30 observations, the following estimated regression equation was obtained: 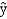 = 17 + 4x1 - 3x2 + 8x3 + 8x4
For this model SSR = 700 and SSE = 100.
-Refer to Exhibit 13-3. The conclusion is that the
= 17 + 4x1 - 3x2 + 8x3 + 8x4
For this model SSR = 700 and SSE = 100.
-Refer to Exhibit 13-3. The conclusion is that the
(Multiple Choice)
4.9/5  (38)
(38)
The following regression model has been proposed to predict monthly sales at a shoe store. 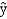 = 40 - 3x1 + 12x2 + 10x3
where
x1 = competitor's previous month's sales (in $1,000s)
x2 = Stores previous month's sales (in $1,000s)
= 40 - 3x1 + 12x2 + 10x3
where
x1 = competitor's previous month's sales (in $1,000s)
x2 = Stores previous month's sales (in $1,000s) 
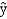 = sales (in $1000s)
a.Predict sales (in dollars) for the shoe store if the competitor's previous month's sales were $9,000, the store's previous month's sales were $30,000, and no radio advertisements were run.
b.Predict sales (in dollars) for the shoe store if the competitor's previous month's sales were $9,000, the store's previous month's sales were $30,000, and 10 radio advertisements were run.
= sales (in $1000s)
a.Predict sales (in dollars) for the shoe store if the competitor's previous month's sales were $9,000, the store's previous month's sales were $30,000, and no radio advertisements were run.
b.Predict sales (in dollars) for the shoe store if the competitor's previous month's sales were $9,000, the store's previous month's sales were $30,000, and 10 radio advertisements were run.
(Essay)
4.8/5  (29)
(29)
Below you are given a partial ANOVA table relating the price of a company's stock (y in dollars), the Dow Jones industrial average (x1), and the stock price of the company's major competitor (x2 in dollars).
 a.What has been the sample size for this regression analysis?
b.At = 0.05 level of significance, test to determine if the model is significant. That is, determine if there exists a significant relationship between the independent variables and the dependent variable.
c.Determine the multiple coefficient of determination.
a.What has been the sample size for this regression analysis?
b.At = 0.05 level of significance, test to determine if the model is significant. That is, determine if there exists a significant relationship between the independent variables and the dependent variable.
c.Determine the multiple coefficient of determination.
(Essay)
4.8/5  (37)
(37)
Exhibit 13-4
a.
y = 0 + 1x1 + 2x2 +
b.
E(y) = 0 + 1x1 + 2x2
c.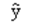 = bo + b1 x1 + b2 x2
d.
E(y) = 0 + 1x1 + 2x2
-Refer to Exhibit 13-4. Which equation gives the estimated regression line?
= bo + b1 x1 + b2 x2
d.
E(y) = 0 + 1x1 + 2x2
-Refer to Exhibit 13-4. Which equation gives the estimated regression line?
(Multiple Choice)
4.7/5  (34)
(34)
The correct relationship between SST, SSR, and SSE is given by
(Multiple Choice)
4.8/5  (39)
(39)
In a residual plot that does not suggest we should challenge the assumptions of our regression model, we would expect to see
(Multiple Choice)
4.8/5  (28)
(28)
A student used multiple regression analysis to study how family spending (y) is influenced by income (x1), family size (x2), and additions to savings (x3). The variables y, x1, and x3 are measured in thousands of dollars. The following results were obtained.
 Coefficient of determination = 0.946
a.Write out the estimated regression equation for the relationship between the variables.
b.What can you say about the strength of this relationship?
c.Carry out a test of whether y is significantly related to the independent variables. Use a .05 level of significance.
d.Carry out a test to see if x3 and y are significantly related. Use a .05 level of significance.
e.Why would a coefficient of determination very close to 1.0 be expected here?
Coefficient of determination = 0.946
a.Write out the estimated regression equation for the relationship between the variables.
b.What can you say about the strength of this relationship?
c.Carry out a test of whether y is significantly related to the independent variables. Use a .05 level of significance.
d.Carry out a test to see if x3 and y are significantly related. Use a .05 level of significance.
e.Why would a coefficient of determination very close to 1.0 be expected here?
(Essay)
4.9/5  (28)
(28)
A measure of goodness of fit for the estimated regression equation is the
(Multiple Choice)
4.9/5  (34)
(34)
A regression was performed on a sample of 16 observations. The estimated equation is 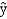 = 23.5 - 14.28x1 + 6.72x2 + 15.68x3. The standard errors for the coefficients are Sb1 = 4.2, Sb2 = 5.6, and Sb3 = 2.8. For this model, SST = 3809.6 and SSR = 3285.4.
a.Compute the appropriate t ratios.
b.Test for the significance of 1, 2, and 3 at the 5% level of significance.
c.Do you think that any of the variables should be dropped from the model? Explain.
d.Compute R2 and Ra2. Interpret R2.
e.Test the significance of the relationship among the variables at the 5% level of significance.
= 23.5 - 14.28x1 + 6.72x2 + 15.68x3. The standard errors for the coefficients are Sb1 = 4.2, Sb2 = 5.6, and Sb3 = 2.8. For this model, SST = 3809.6 and SSR = 3285.4.
a.Compute the appropriate t ratios.
b.Test for the significance of 1, 2, and 3 at the 5% level of significance.
c.Do you think that any of the variables should be dropped from the model? Explain.
d.Compute R2 and Ra2. Interpret R2.
e.Test the significance of the relationship among the variables at the 5% level of significance.
(Essay)
4.7/5  (29)
(29)
Exhibit 13-3
In a regression model involving 30 observations, the following estimated regression equation was obtained: 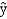 = 17 + 4x1 - 3x2 + 8x3 + 8x4
For this model SSR = 700 and SSE = 100.
-Refer to Exhibit 13-3. The computed F statistic for testing the significance of the above model is
= 17 + 4x1 - 3x2 + 8x3 + 8x4
For this model SSR = 700 and SSE = 100.
-Refer to Exhibit 13-3. The computed F statistic for testing the significance of the above model is
(Multiple Choice)
4.9/5  (32)
(32)
In order to test for the significance of a regression model involving 14 independent variables and 255 observations, the numerator and denominator degrees of freedom (respectively) for the critical value of F are
(Multiple Choice)
4.7/5  (38)
(38)
A variable that cannot be measured in terms of how much or how many but instead is assigned values to represent categories is called
(Multiple Choice)
4.8/5  (36)
(36)
Exhibit 13-2
A regression model between sales (y in $1,000), unit price (x1 in dollars) and television advertisement (x2 in dollars) resulted in the following function: 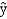 = 7 - 3x1 + 5x2
For this model SSR = 3500, SSE = 1500, and the sample size is 18.
-Refer to Exhibit 13-2. The coefficient of x2 indicates that if television advertising is increased by $1 (holding the unit price constant), sales are expected to
= 7 - 3x1 + 5x2
For this model SSR = 3500, SSE = 1500, and the sample size is 18.
-Refer to Exhibit 13-2. The coefficient of x2 indicates that if television advertising is increased by $1 (holding the unit price constant), sales are expected to
(Multiple Choice)
4.7/5  (36)
(36)
Exhibit 13-2
A regression model between sales (y in $1,000), unit price (x1 in dollars) and television advertisement (x2 in dollars) resulted in the following function: 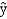 = 7 - 3x1 + 5x2
For this model SSR = 3500, SSE = 1500, and the sample size is 18.
-Refer to Exhibit 13-2. If we want to test for the significance of the regression model, the critical value of F at 95% confidence is
= 7 - 3x1 + 5x2
For this model SSR = 3500, SSE = 1500, and the sample size is 18.
-Refer to Exhibit 13-2. If we want to test for the significance of the regression model, the critical value of F at 95% confidence is
(Multiple Choice)
4.9/5  (43)
(43)
A regression model involving 8 independent variables for a sample of 69 periods resulted in the following sum of squares.
SSE = 306
SST = 1800
a.Compute the coefficient of determination.
b.At = 0.05, test to determine whether or not the model is significant.
(Essay)
4.8/5  (34)
(34)
The following regression model has been proposed to predict sales at a fast food outlet. 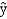 = 18 - 2x1 + 7x2 + 15x3
where
x1 = the number of competitors within 1 mile
x2 = the population within 1 mile (in 1,000s)
x3 = 1 if drive-up windows are present, 0 otherwise
= 18 - 2x1 + 7x2 + 15x3
where
x1 = the number of competitors within 1 mile
x2 = the population within 1 mile (in 1,000s)
x3 = 1 if drive-up windows are present, 0 otherwise 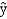 = sales (in $1,000s)
a.What is the interpretation of 15 (the coefficient of x3) in the regression equation?
b.Predict sales for a store with 2 competitors, a population of 10,000 within one mile, and one drive-up window (give the answer in dollars).
c.Predict sales for the store with 2 competitors, a population of 10,000 within one mile, and no drive-up window (give the answer in dollars).
= sales (in $1,000s)
a.What is the interpretation of 15 (the coefficient of x3) in the regression equation?
b.Predict sales for a store with 2 competitors, a population of 10,000 within one mile, and one drive-up window (give the answer in dollars).
c.Predict sales for the store with 2 competitors, a population of 10,000 within one mile, and no drive-up window (give the answer in dollars).
(Essay)
4.8/5  (36)
(36)
In order to determine whether or not the number of automobiles sold per day (y) is related to price (x1 in $1,000), and the number of advertising spots (x2), data were gathered for 7 days. Part of the Excel output is shown below.
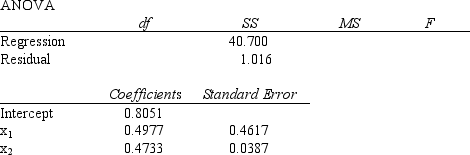 a.Determine the least squares regression function relating y to x1 and x2.
b.If the company charges $20,000 for each car and uses 10 advertising spots, how many cars would you expect them to sell in a day?
c.At = 0.05, test to determine if the fitted equation developed in Part a represents a significant relationship between the independent variables and the dependent variable.
d.At = 0.05, test to see if 1 is significantly different from zero.
e.Determine the multiple coefficient of determination.
a.Determine the least squares regression function relating y to x1 and x2.
b.If the company charges $20,000 for each car and uses 10 advertising spots, how many cars would you expect them to sell in a day?
c.At = 0.05, test to determine if the fitted equation developed in Part a represents a significant relationship between the independent variables and the dependent variable.
d.At = 0.05, test to see if 1 is significantly different from zero.
e.Determine the multiple coefficient of determination.
(Essay)
4.9/5  (27)
(27)
The following is part of the results of a regression analysis involving sales (y in millions of dollars), advertising expenditures (x1 in thousands of dollars), and number of salespeople (x2) for a corporation. The regression was performed on a sample of 10 observations.
 a.Write the regression equation.
b.Interpret the coefficients of the estimated regression equation found in Part (a).
c.At =0.05, test for the significance of the coefficient of advertising.
d.At =0.05, test for the significance of the coefficient of number of salespeople.
e.If the company uses $50,000 in advertisement and has 800 salespersons, what are the expected sales? Give your answer in dollars.
a.Write the regression equation.
b.Interpret the coefficients of the estimated regression equation found in Part (a).
c.At =0.05, test for the significance of the coefficient of advertising.
d.At =0.05, test for the significance of the coefficient of number of salespeople.
e.If the company uses $50,000 in advertisement and has 800 salespersons, what are the expected sales? Give your answer in dollars.
(Essay)
4.8/5  (34)
(34)
Showing 21 - 40 of 111
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)