Exam 2: Linear Programming: Model Formulation and Graphical Solution

Consider the following linear programming problem: Max Z =    $3x + $9y Subject to:    20x + 32y ? 1600       4x + 2y ? 240       y ? 40       x, y ? 0 Solve for the quantities of x and y which will maximize Z. What is the value of the slack variable associated with constraint 2?

The following is a graph of a linear programming problem. The feasible solution space is shaded, and the optimal solution is at the point labeled Z*. 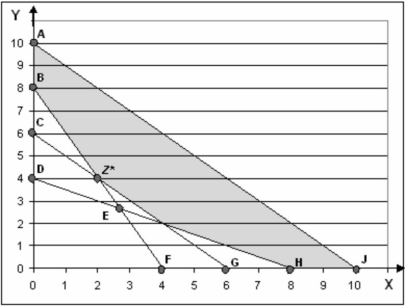-The constraint AJ

The region that satisfies all of the constraints in a graphical linear programming problem is called the

In linear programming models , objective functions can only be maximized.

In a linear programming problem, a valid objective function can be represented as:

Linear programming is a model consisting of linear relationships representing a firm's decisions given an objective and resource constraints.

Multiple optimal solutions occur when constraints are parallel to each other.

Which of the following statements is not true?

A manufacturer using linear programming to decide the best product mix to maximize profit typically has a(n) ________ constraint included in the model.

The feasible solution area contains infinite solutions to the linear program.

A hot dog manufacturer wishes to minimize the cost in dollars of producing a low-cost niched product while meeting the dietary guidelines for protein and sodium. Once the model has been run, the surplus variable in the sodium constraint has a value of 1300 milligrams. The best interpretation of this outcome is:

Consider the following linear program: MAX z = 5x + 3y S .t.    x - y ? 6    X ? 1 The optimal solution

There is exactly one optimal solution point to a linear program.

Typically, finding a corner point for the feasible region involves solving a set of three simultaneous equations.

Consider the following minimization problem: Min z = x1 + 2x2 s.t.   x1 + x2 ? 300     2x1 + x2 ? 400     2x1 + 5x2 ? 750     x1, x2 ? 0 What is the optimal solution?

Non-negativity constraints

In a linear programming problem, the binding constraints for the optimal solution are: 5x1 + 3x2 ≤ 30 2x1 + 5x2 ≤ 20 As long as the slope of the objective function stays between ________ and ________, the current optimal solution point will remain optimal.

Given this set of constraints, for what objective function is the point x=5, y=3 in the feasible region? s.t 3x + 6y ≤ 30 10x + 10y ≤ 60 10x + 15y ≤ 90

The theoretical limit on the number of constraints that can be handled by a linear programming problem is:

A ________ is a linear relationship representing a restriction on decision making.