Exam 14: Introduction to Multiple Regression
Exam 1: Instruction and Data Collection47 Questions
Exam 2: Presenting Data in Tables and Charts277 Questions
Exam 3: Numerical Descriptive Measures139 Questions
Exam 4: Basic Probability137 Questions
Exam 5: Some Important Discrete Probability Distributions188 Questions
Exam 6: The Normal Distribution and Other Continuous Distributions164 Questions
Exam 7: Sampling and Sampling Distributions187 Questions
Exam 8: Confidence Interval Estimation173 Questions
Exam 9: Fundamentals of Hypothesis Testing: One-Sample Tests146 Questions
Exam 10: Two-Sample Tests190 Questions
Exam 11: Analysis of Variance127 Questions
Exam 12: Chi-Square Tests and Nonparametric Tests174 Questions
Exam 13: Simple Linear Regression198 Questions
Exam 14: Introduction to Multiple Regression215 Questions
Exam 15: Multiple Regression Model Building101 Questions
Exam 16: Time-Series Analysis and Index Numbers133 Questions
Exam 17: Statistical Applications in Quality Management132 Questions
Exam 18: Data Analysis Overview52 Questions
Select questions type
TABLE 14-5
A microeconomist wants to determine how corporate sales are influenced by capital and wage spending by companies. She proceeds to randomly select 26 large corporations and record information in millions of dollars. The Microsoft Excel output below shows results of this multiple regression.
SUMMARY OUTPUT
Regression Statistics
 ANOVA
ANOVA

 -Referring to Table 14-5, what is the p-value for testing whether Capital has a negative influence on corporate sales?
-Referring to Table 14-5, what is the p-value for testing whether Capital has a negative influence on corporate sales?
(Multiple Choice)
4.8/5  (29)
(29)
TABLE 14-15
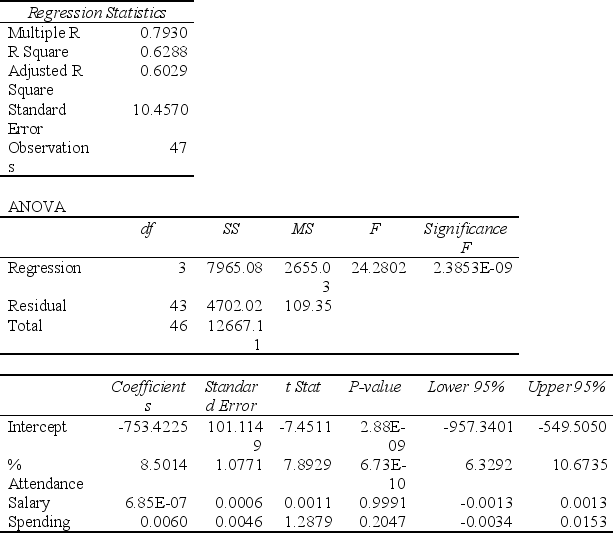
The superintendent of a school district wanted to predict the percentage of students passing a sixth-grade proficiency test. She obtained the data on percentage of students passing the proficiency test (% Passing), daily average of the percentage of students attending class (% Attendance), average teacher salary in dollars (Salaries), and instructional spending per pupil in dollars (Spending) of 47 schools in the state.
Following is the multiple regression output with Y = % Passing as the dependent variable, X1= % Attendance, X2= Salaries and X3= Spending:
 Note:
Note:
 -Referring to Table 14-15, which of the following is the correct null hypothesis to test whether instructional spending per pupil has any effect on percentage of students passing the proficiency test?
-Referring to Table 14-15, which of the following is the correct null hypothesis to test whether instructional spending per pupil has any effect on percentage of students passing the proficiency test?
(Multiple Choice)
4.9/5  (33)
(33)
TABLE 14-2
A professor of industrial relations believes that an individual's wage rate at a factory (Y) depends on his performance rating (X1) and the number of economics courses the employee successfully completed in college (X2). The professor randomly selects 6 workers and collects the following information:
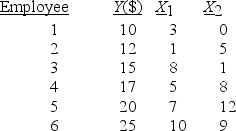 -Referring to Table 14-2, for these data, what is the estimated coefficient for performance rating, b1?
-Referring to Table 14-2, for these data, what is the estimated coefficient for performance rating, b1?
(Multiple Choice)
4.8/5  (32)
(32)
TABLE 14-15
The superintendent of a school district wanted to predict the percentage of students passing a sixth-grade proficiency test. She obtained the data on percentage of students passing the proficiency test (% Passing), daily average of the percentage of students attending class (% Attendance), average teacher salary in dollars (Salaries), and instructional spending per pupil in dollars (Spending) of 47 schools in the state.
Following is the multiple regression output with Y = % Passing as the dependent variable, X1= % Attendance, X2= Salaries and X3= Spending:
 Note:
Note:
 -Referring to Table 14-15, what is the value of the test statistic when testing whether daily average of the percentage of students attending class has any effect on percentage of students passing the proficiency test?
-Referring to Table 14-15, what is the value of the test statistic when testing whether daily average of the percentage of students attending class has any effect on percentage of students passing the proficiency test?
(Short Answer)
4.8/5  (36)
(36)
TABLE 14-3
An economist is interested to see how consumption for an economy (in $ billions) is influenced by gross domestic product ($ billions) and aggregate price (consumer price index). The Microsoft Excel output of this regression is partially reproduced below.
SUMMARY OUTPUT
Regression Statistics
 ANOVA
ANOVA

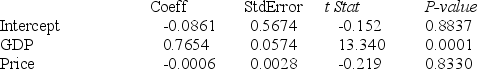 -Referring to Table 14-3, to test whether aggregate price index has a negative impact on consumption, the p-value is ________.
-Referring to Table 14-3, to test whether aggregate price index has a negative impact on consumption, the p-value is ________.
(Multiple Choice)
4.8/5  (39)
(39)
TABLE 14-5
A microeconomist wants to determine how corporate sales are influenced by capital and wage spending by companies. She proceeds to randomly select 26 large corporations and record information in millions of dollars. The Microsoft Excel output below shows results of this multiple regression.
SUMMARY OUTPUT
Regression Statistics
 ANOVA
ANOVA

 -Referring to Table 14-5, what is the p-value for Capital?
-Referring to Table 14-5, what is the p-value for Capital?
(Multiple Choice)
4.8/5  (35)
(35)
You have just run a regression in which the value of coefficient of multiple determination is 0.57. To determine if this indicates that the independent variables explain a significant portion of the variation in the dependent variable, you would perform an F-test.
(True/False)
4.7/5  (34)
(34)
TABLE 14-15
The superintendent of a school district wanted to predict the percentage of students passing a sixth-grade proficiency test. She obtained the data on percentage of students passing the proficiency test (% Passing), daily average of the percentage of students attending class (% Attendance), average teacher salary in dollars (Salaries), and instructional spending per pupil in dollars (Spending) of 47 schools in the state.
Following is the multiple regression output with Y = % Passing as the dependent variable, X1= % Attendance, X2= Salaries and X3= Spending:
 Note:
Note:
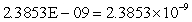 -Referring to Table 14-15, there is sufficient evidence that the percentage of students passing the proficiency test depends on at least one of the explanatory variables.
-Referring to Table 14-15, there is sufficient evidence that the percentage of students passing the proficiency test depends on at least one of the explanatory variables.
(True/False)
4.7/5  (31)
(31)
TABLE 14-15
The superintendent of a school district wanted to predict the percentage of students passing a sixth-grade proficiency test. She obtained the data on percentage of students passing the proficiency test (% Passing), daily average of the percentage of students attending class (% Attendance), average teacher salary in dollars (Salaries), and instructional spending per pupil in dollars (Spending) of 47 schools in the state.
Following is the multiple regression output with Y = % Passing as the dependent variable, X1= % Attendance, X2= Salaries and X3= Spending:
 Note:
Note:
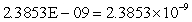 -Referring to Table 14-15, the alternative hypothesis H1 : At least one of βj ≠ 0 for j = 1, 2, 3 implies that percentage of students passing the proficiency test is related to all of the explanatory variables.
-Referring to Table 14-15, the alternative hypothesis H1 : At least one of βj ≠ 0 for j = 1, 2, 3 implies that percentage of students passing the proficiency test is related to all of the explanatory variables.
(True/False)
4.9/5  (29)
(29)
TABLE 14-15
The superintendent of a school district wanted to predict the percentage of students passing a sixth-grade proficiency test. She obtained the data on percentage of students passing the proficiency test (% Passing), daily average of the percentage of students attending class (% Attendance), average teacher salary in dollars (Salaries), and instructional spending per pupil in dollars (Spending) of 47 schools in the state.
Following is the multiple regression output with Y = % Passing as the dependent variable, X1= % Attendance, X2= Salaries and X3= Spending:
 Note:
Note:
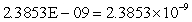 -Referring to Table 14-15, the alternative hypothesis H1 : At least one of βj ≠ 0 for j = 1, 2, 3 implies that percentage of students passing the proficiency test is affected by at least one of the explanatory variables.
-Referring to Table 14-15, the alternative hypothesis H1 : At least one of βj ≠ 0 for j = 1, 2, 3 implies that percentage of students passing the proficiency test is affected by at least one of the explanatory variables.
(True/False)
4.8/5  (34)
(34)
TABLE 14-7
The department head of the accounting department wanted to see if she could predict the GPA of students using the number of course units (credits) and total SAT scores of each. She takes a sample of students and generates the following Microsoft Excel output:
SUMMARY OUTPUT
Regression Statistics
 ANOVA
ANOVA

 -Referring to Table 14-7, the department head wants to test H0 : β1 = β2 = 0. The appropriate alternative hypothesis is ________.
-Referring to Table 14-7, the department head wants to test H0 : β1 = β2 = 0. The appropriate alternative hypothesis is ________.
(Short Answer)
4.8/5  (32)
(32)
TABLE 14-8
A financial analyst wanted to examine the relationship between salary (in $1,000) and 4 variables: age (X1 = Age), experience in the field (X2 = Exper), number of degrees (X3 = Degrees), and number of previous jobs in the field (X4 = Prevjobs). He took a sample of 20 employees and obtained the following Microsoft Excel output:
SUMMARY OUTPUT
Regression Statistics
 ANOVA
ANOVA

 -Referring to Table 14-8, the analyst wants to use a t test to test for the significance of the coefficient of X3. The value of the test statistic is ________.
-Referring to Table 14-8, the analyst wants to use a t test to test for the significance of the coefficient of X3. The value of the test statistic is ________.
(Short Answer)
4.8/5  (34)
(34)
TABLE 14-5
A microeconomist wants to determine how corporate sales are influenced by capital and wage spending by companies. She proceeds to randomly select 26 large corporations and record information in millions of dollars. The Microsoft Excel output below shows results of this multiple regression.
SUMMARY OUTPUT
Regression Statistics
 ANOVA
ANOVA

 -Referring to Table 14-5, one company in the sample had sales of $21.439 billion (Sales = 21,439). This company spent $300 million on capital and $700 million on wages. What is the residual (in millions of dollars) for this data point?
-Referring to Table 14-5, one company in the sample had sales of $21.439 billion (Sales = 21,439). This company spent $300 million on capital and $700 million on wages. What is the residual (in millions of dollars) for this data point?
(Multiple Choice)
4.9/5  (28)
(28)
TABLE 14-3
An economist is interested to see how consumption for an economy (in $ billions) is influenced by gross domestic product ($ billions) and aggregate price (consumer price index). The Microsoft Excel output of this regression is partially reproduced below.
SUMMARY OUTPUT
Regression Statistics
 ANOVA
ANOVA

 -Referring to Table 14-3, one economy in the sample had an aggregate consumption level of $4 billion, a GDP of $6 billion, and an aggregate price level of 200. What is the residual for this data point?
-Referring to Table 14-3, one economy in the sample had an aggregate consumption level of $4 billion, a GDP of $6 billion, and an aggregate price level of 200. What is the residual for this data point?
(Multiple Choice)
4.9/5  (38)
(38)
TABLE 14-2
A professor of industrial relations believes that an individual's wage rate at a factory (Y) depends on his performance rating (X1) and the number of economics courses the employee successfully completed in college (X2). The professor randomly selects 6 workers and collects the following information:
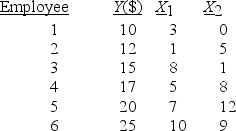 -Referring to Table 14-2, for these data, what is the estimated coefficient for the number of economics courses taken, b2?
-Referring to Table 14-2, for these data, what is the estimated coefficient for the number of economics courses taken, b2?
(Multiple Choice)
4.9/5  (41)
(41)
TABLE 14-9
You decide to predict gasoline prices in different cities and towns in the United States for your term project. Your dependent variable is price of gasoline per gallon and your explanatory variables are per capita income, the number of firms that manufacture automobile parts in and around the city, the number of new business starts in the last year, population density of the city, percentage of local taxes on gasoline, and the number of people using public transportation. You collected data of 32 cities and obtained a regression sum of squares SSR= 122.8821. Your computed value of standard error of the estimate is 1.9549.
-Referring to Table 14-9, the value of adjusted r2 is
(Multiple Choice)
4.9/5  (35)
(35)
TABLE 14-15
The superintendent of a school district wanted to predict the percentage of students passing a sixth-grade proficiency test. She obtained the data on percentage of students passing the proficiency test (% Passing), daily average of the percentage of students attending class (% Attendance), average teacher salary in dollars (Salaries), and instructional spending per pupil in dollars (Spending) of 47 schools in the state.
Following is the multiple regression output with Y = % Passing as the dependent variable, X1= % Attendance, X2= Salaries and X3= Spending:
 Note:
Note:
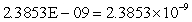 -Referring to Table 14-15, what are the lower and upper limits of the 95% confidence interval estimate for the effect of a one dollar increase in average teacher salary on the mean percentage of students passing the proficiency test?
-Referring to Table 14-15, what are the lower and upper limits of the 95% confidence interval estimate for the effect of a one dollar increase in average teacher salary on the mean percentage of students passing the proficiency test?
(Short Answer)
4.8/5  (40)
(40)
In calculating the standard error of the estimate, , there are n - k - 1 degrees of freedom, where n is the sample size and k represents the number of independent variables in the model.
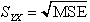
(True/False)
4.7/5  (43)
(43)
TABLE 14-15
The superintendent of a school district wanted to predict the percentage of students passing a sixth-grade proficiency test. She obtained the data on percentage of students passing the proficiency test (% Passing), daily average of the percentage of students attending class (% Attendance), average teacher salary in dollars (Salaries), and instructional spending per pupil in dollars (Spending) of 47 schools in the state.
Following is the multiple regression output with Y = % Passing as the dependent variable, X1= % Attendance, X2= Salaries and X3= Spending:
 Note:
Note:
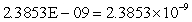 -Referring to Table 14-15, we can conclude that average teacher salary has no impact on the mean percentage of students passing the proficiency test at a 5% level of significance using the 95% confidence interval estimate for β2.
-Referring to Table 14-15, we can conclude that average teacher salary has no impact on the mean percentage of students passing the proficiency test at a 5% level of significance using the 95% confidence interval estimate for β2.
(True/False)
4.8/5  (36)
(36)
TABLE 14-1
A manager of a product sales group believes the number of sales made by an employee (Y) depends on how many years that employee has been with the company (X1) and how he/she scored on a business aptitude test (X2). A random sample of 8 employees provides the following:
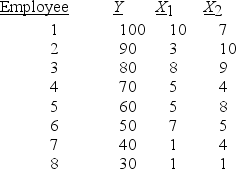 -Referring to Table 14-1, for these data, what is the estimated coefficient for the variable representing years an employee has been with the company, b1?
-Referring to Table 14-1, for these data, what is the estimated coefficient for the variable representing years an employee has been with the company, b1?
(Multiple Choice)
4.8/5  (39)
(39)
Showing 181 - 200 of 215
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)