Exam 15: Topics in Vector Calculus
Exam 1: Limits and Continuity186 Questions
Exam 2: The Derivative198 Questions
Exam 3: Topics in Deifferentiation171 Questions
Exam 4: The Derivative in Graphing and Applications656 Questions
Exam 5: Integration323 Questions
Exam 6: Applications of the Definite Integral in Geometry, Science and Engineering314 Questions
Exam 7: Principle of Integral Evaluation269 Questions
Exam 8: Mathematical Modeling With Differential Equations77 Questions
Exam 9: Infinte Series288 Questions
Exam 10: Parametric and Polar Curves; Conic Sections199 Questions
Exam 11: Three-Dimensional Space; Vectors173 Questions
Exam 12: Vector-Valued Functions147 Questions
Exam 13: Partial Derivatives194 Questions
Exam 14: Multiple Integrals117 Questions
Exam 15: Topics in Vector Calculus149 Questions
Select questions type
Use Stokes' Theorem to evaluate C 2(x + y)dx + 2(2x - 3)dy + 2(y + z)dz over the boundary of the triangle with vertices (2, 0, 0), (0, 3, 0), and (0, 0, 6) traversed in a counterclockwise manner.
(Multiple Choice)
4.8/5  (32)
(32)
Evaluate 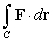 where F(x, y, z) = 12z i + 12x j + 12y k and C is the helix r(t) = sin t i + 3 sin t j + sin2t k for
where F(x, y, z) = 12z i + 12x j + 12y k and C is the helix r(t) = sin t i + 3 sin t j + sin2t k for  .
.
(Short Answer)
4.7/5  (40)
(40)
Use Green's Theorem to evaluate 4(3x2 + y)dx + 8xy3dy where C is the rectangle bounded by x = -1, x = 3, y = 0, and y = 2.
(Short Answer)
4.7/5  (36)
(36)
Use the divergence theorem to evaluate 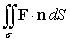 where F(x, y, z) = 11yz i + 11xy j + 11xz k, n is the outer unit normal to , and is the surface enclosed by the cylinder x2 + z2 = 1 and the planes y = -1 and y = 1.
where F(x, y, z) = 11yz i + 11xy j + 11xz k, n is the outer unit normal to , and is the surface enclosed by the cylinder x2 + z2 = 1 and the planes y = -1 and y = 1.
(Short Answer)
4.9/5  (30)
(30)
Evaluate the surface integral 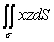 where
where 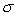 is the part of the plane
is the part of the plane 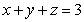 in the first octant.
in the first octant.
(Multiple Choice)
4.8/5  (38)
(38)
If F(x, y, z) = 7y j + 7z k, the magnitude of the flux through the portion of the surface that lies in front of the xz-plane, where is defined by y = 1 - x2 - z2, is
(Multiple Choice)
4.7/5  (25)
(25)
Sketch the vector field, F(x, y) = -5x j, by drawing some typical non-intersecting vectors. The vectors need not be drawn to the same scale as the coordinate axes, but they should be in the correct proportions relative to each other.
(Essay)
4.8/5  (32)
(32)
Evaluate the surface integral 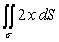 where is that portion of the plane x + 2y + 3z = 6 which lies in the first octant.
where is that portion of the plane x + 2y + 3z = 6 which lies in the first octant.
(Essay)
4.8/5  (39)
(39)
Find the surface area of (x - 7)2 + (y + 1)2 + (z - 4)2 = 4 that lies below z = 6.
(Multiple Choice)
4.8/5  (23)
(23)
Evaluate the surface integral 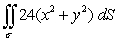 where is that portion of the cylinder x2 + z2 = 1 that lies above the xy-plane enclosed by 0 y 5.
where is that portion of the cylinder x2 + z2 = 1 that lies above the xy-plane enclosed by 0 y 5.
(Essay)
4.7/5  (38)
(38)
Find the outward flux of F(x, y, z) = 4x i + 4y j + 4(z - 2)k across the rectangular box with vertices (0, 0, 0), (0, 0, 1), (0, 1, 0), (0, 1, 1), (3, 0, 0), (3, 1, 0), (3, 0, 1), and (3, 1, 1).
(Multiple Choice)
4.8/5  (39)
(39)
Use Stokes' Theorem to evaluate C -21 dx + 21x dy + 7z dz over the circle x2 + y2 = 1, z = 1 traversed counterclockwise.
(Essay)
4.9/5  (34)
(34)
Find the surface area of (x - 1)2 + (y + 1)2 + (z - 4)2 = 4 that lies below z = 4.
(Multiple Choice)
4.8/5  (39)
(39)
Evaluate the surface integral 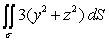 where is the portion of the cone
where is the portion of the cone 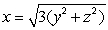 for 0 x 3.
for 0 x 3.
(Essay)
4.9/5  (31)
(31)
Evaluate 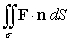 where F(x, y, z) = 2x i + 2y j + 4z k and is that portion of the surface z = 4 - x2 - y2 above the xy-plane oriented by upward unit normals.
where F(x, y, z) = 2x i + 2y j + 4z k and is that portion of the surface z = 4 - x2 - y2 above the xy-plane oriented by upward unit normals.
(Essay)
4.8/5  (36)
(36)
Evaluate the surface integral 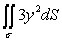 where is that portion of the cylinder y2 + z2 = 1 that lies above the xy-plane enclosed by 0 x 5 and -1 y 1.
where is that portion of the cylinder y2 + z2 = 1 that lies above the xy-plane enclosed by 0 x 5 and -1 y 1.
(Multiple Choice)
5.0/5  (32)
(32)
Showing 61 - 80 of 149
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)