Exam 4: Exponential and Logarithmic Functions

Solve the equation. Write the solution set with the exact values given in terms of natural or common logarithms. Also give approximate solutions to 4 decimal places, if necessary. - $3 ^ { 3 x - 3 } = 2 ^ { 5 x - 4 }$

Use the definition of a one-to-one function to determine if the function is one-to-one. - $f ( x ) = | x - 4 |$

Approximate $f ( x ) = \ln x$ for the given value of x. Round to four decimal places. -f (460)

Write the logarithmic expression as a single logarithm with coefficient 1, and simplify as much as possible. - $5 \log _ { b } y + 3 \log _ { b } z$

Choose the one alternative that best completes the statement or answers the question. Solve for the indicated variable. - $N = N _ { 0 } e ^ { - 0.0361 t }$ for $t$ $\ln \frac { N } { N _ { 0 } }$

Solve the equation. Write the solution set with the exact values given in terms of natural or common logarithms. Also give approximate solutions to 4 decimal places, if necessary. - $4 e ^ { 5 m - 5 } - 2 = 14$

Write the word or phrase that best completes each statement or answers the question. Provide the missing information. -The change-of-base formula indicates that logb x can be written as a ratio of logarithms with base a as: $\log _ { b } x = \frac { \square } { \square }$

Determine if the statement is true or false. - $\ln 10 = \frac { 1 } { \log e }$

Simplify the expression. - $\log 0.00001$

Solve the problem. - $\$ 14,000$ is invested at $6 \%$ interest compounded quarterly and grows to $\$ 16,738.65$ . For how long was the money invested? Round to the nearest year.

Solve the problem. -The population of a country on January 1, 2000, is $20.7$ million and on January 1, 2010, it has risen to $22.6$ million. Write a function of the form $P ( t ) = P _ { 0 } e ^ { r t }$ to model the population $P ( t )$ (in millions) $t$ years after January 1, 2000. Then use the model to predict the population of the country on January $1,2,017$ . round to the nearest hundred thousand.

Solve the equation. - $\log _ { 2 } ( 72 x ) - \log _ { 2 } ( x + 96 ) = 3$

Solve the equation. - $e ^ { 2 x } - 14 e ^ { x } + 9 = 0$

Write the logarithmic expression as a single logarithm with coefficient 1, and simplify as much as possible. - $\log _ { 4 } 112 - \log _ { 4 } 7$

Solve the problem. -Given that $\log 5 \approx 0.6990$ and $\log 9 \approx 0.9542$ , use the properties of logarithms to approximate the following. Do not use a calculator. $\log \frac { 5 } { 81 }$

Solve the problem. -If $\$ 18,000$ is put aside in a money market account with interest reinvested monthly at $2.3 \%$ , find the time required for the account to earn $\$ 2,000$ . Round to the nearest month. Use the model $A = P$ $\left( 1 + \frac { r } { n } \right) ^ { n t }$ where $A$ represents the future value of $P$ dollars invested at an interest rate $r$ compounded $n$ times per year for $t$ years.

Solve the problem. -After a new product is launched the cumulative sales $S ( t )$ (in $\$ 1000 ) t$ weeks after launch is given by: $S ( t ) = \frac { 64 } { 1 + 8 e ^ { - 0.47 t } }$ What is the limiting value in sales?

Graph the function and write the domain and range in interval notation. - $f ( x ) = \left( \frac { 2 } { 5 } \right) ^ { x }$

Solve the problem. -The function $P ( t ) = 8 ( 1.0134 ) ^ { t }$ represents the population (in millions) of a country $t$ years after January 1, 2000. Write an equivalent function using base $e$ ; that is, write a function of the $P ( t ) = P _ { 0 } e ^ { r t }$ . Round $r$ to 5 decimal places. Also, determine the population for the year 2000 .

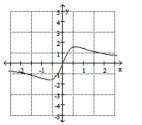
Determine if the relation defines y as a one-to-one function of x. -