Exam 4: Exponential and Logarithmic Functions

Choose the one alternative that best completes the statement or answers the question. Evaluate the function at the given value of x. Round to 4 decimal places if necessary. - $f ( x ) = \left( \frac { 1 } { 4 } \right) ^ { x } ; \quad f ( - 5 )$

Solve the logarithmic equation. - $\log _ { 9 } t ^ { 3 } = \log _ { 9 } t ^ { 2 } - 2$

Choose the one alternative that best completes the statement or answers the question. Write the equation in exponential form. - $\log _ { 4 } 16 = 2$

A function defined by $y = f ( x ) \text { (is/is not) }$ ) (is/is not) a one-to-one function if no horizontal line intersects the graph of f in more than one point.

Solve the equation. - $\log _ { 5 } ( 5 p + 3 ) + \log _ { 5 } p = \log _ { 5 } 14$

Solve the equation. Write the solution set with the exact solutions. Also give approximate solutions to 4 decimal places if necessary. - $\log _ { 5 } x - \log _ { 5 } ( 2 x + 6 ) = \frac { 1 } { 2 } \log _ { 5 } 4$

Solve the problem. -The formula $L = 10 \log \left( \frac { I } { I _ { 0 } } \right)$ gives the loudness of sound $L$ (in $\mathrm { dB }$ ) based on the intensity of the sound (in $\mathrm { W } / \mathrm { m } ^ { 2 }$ ). The value $I _ { 0 } = 10 ^ { - 12 } \mathrm {~W} / \mathrm { m } ^ { 2 }$ is the minimal threshold for hearing for mid-frequency sounds. Hearing impairment is often measured according to the minimal sound level (in $\mathrm { dB }$ ) detected by an individual for sounds of various frequencies. If the minimum loudness of sound detected by an individual is $60 \mathrm {~dB}$ , determine the corresponding intensity of sound.

Graph the points and from visual inspection, select the model that would best fit the data. Choose from y=mx+b (linear) y=a (exponential) y=a+bx (logarithmic) y= (logistic) Then use a graphing utility to find a function that fits the data. (Hint: For a logistic model, go to STAT, CALC, Logistic.) - x y 4 4.4 5 5.2 9 10.5 14 25.1 20 71.3 24 143.1

Simplify the expression. - $4 \log _ { 4 } \left( x ^ { 5 } - 7 \right)$

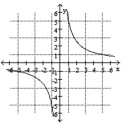
Determine if the relation defines y as a one-to-one function of x. -

Find an equation for the inverse function. - $f ( x ) = 8 ^ { x } - 3$

Find an equation for the inverse function. - $f ( x ) = \ln ( x + 3 )$

Solve the problem. -Given that the domain of a one-to-one function $f$ is $[ - 8 , - 1 )$ and the range of $f$ is $( 8 , \infty )$ , state the domain and range of $f ^ { - 1 }$ .

Graph the function. - $y = \log _ { 1 / 3 } x$

Write the word or phrase that best completes each statement or answers the question. Provide the missing information. - $\text { As } x \rightarrow \infty \text {, the value of } \left( 1 + \frac { 1 } { x } \right) ^ { x } \text { approaches }$ ــــــــــــــ.

Use transformations of the graph $y = e ^ { x }$ to graph the function. Write the domain and range in interval notation. - $f ( x ) = e ^ { x + 2 }$

Write the word or phrase that best completes each statement or answers the question. Provide the missing information. - $\log _ { b } b =$ ـــــــــــــــــــ because $b ^ { \square } = b$ .

Write the word or phrase that best completes each statement or answers the question. Provide the missing information. -The domain of an exponential function $f ( x ) = b ^ { x }$ is .

Graph the points and from visual inspection, select the model that would best fit the data. Choose from y=mx+b (linear) y=a (exponential) y=a+bx (logarithmic) y= (logistic) Then use a graphing utility to find a function that fits the data. (Hint: For a logistic model, go to STAT, CALC, Logistic.) - x y 10 41.2 20 47.5 30 51.2 40 53.8 50 55.9 60 57.5

Write the word or phrase that best completes each statement or answers the question. Provide the missing information. - $\text { Given a logistic growth function } y = \frac { c } { 1 + a e ^ { - b t } } \text {, the limiting value of } y \text { is }$ ــــــــــــــ