Exam 1: Review of Basic Concepts

Determine what signs on values of x and y would make the statement true. Assume that x and y are not 0. -Find the magnitude of the difference between a windchill factor of -75 and a windchill factor of 156) -25.

Answer the question. - $( 3 m - 8 w ) ( 3 m + 8 w )$

Evaluate the expression. - $( 7 + ( - 4 ) ) [ 7 + ( 6 + 9 ) ]$

Tell whether the statement is true or false. -Let $A = \{ 6,7,8,9,10,11 \} , B = \{ 8,10,12 \} , C = \{ 6,7,9,11 \} , D = \{ 6,11 \}$ , and $U = \{ 6,7,8,9,10,11,12 \}$ . $\mathrm { B } \nsubseteq \mathrm { C }$

Factor by any method. - $y ^ { 2 } - 81$

Find the product or quotient. - $\frac { 3 x ^ { 2 } } { 2 } \cdot \frac { 10 } { x ^ { 3 } }$

Solve the problem. -The length of the diagonal of a box is given by $D = \sqrt { L ^ { 2 } + W ^ { 2 } + H ^ { 2 } }$ where $L , W$ , and $H$ are the length, width, and height of the box. Find the length of the diagonal, D, of a box that is $4 \mathrm { ft }$ long, $2 \mathrm { ft }$ high, and $4 \mathrm { ft }$ wide. Give the exact value.

Simplify the expression. - $\sqrt { ( 3 y + 5 ) ^ { 2 } }$

Simplify the expression. Assume all variables represent positive real numbers. - $\sqrt { 294 }$

Tell whether the statement is true or false. -Let $A = \{ 3,4,5,6,7,8 \} , B = \{ 5,7,9 \} , C = \{ 3,4,6,8 \} , D = \{ 3,8 \}$ , and $U = \{ 3,4,5,6,7,8,9 \}$ . $\mathrm { A } \subseteq \mathrm { U }$

Round to two decimal places unless otherwise indicated. If the average cost per unit C(x) to produce x units of plywood is given by C(x) , what is the -Suppose a cost-benefit model is given by $y = \frac { 1.5 x } { 100 - x }$ , where $y$ is the cost in thousands of dollars for removing x percent of a given pollutant. Find the cost of removing $25 \%$ to the nearest dollar.

Simplify the expression. Assume all variables represent nonzero real numbers. - $( - 3 x ) ^ { 2 }$

Simplify the expression. - $\sqrt { ( z - 5 ) ^ { 2 } }$

Solve the problem. - $( 6 a - 5 ) ^ { 2 }$

Use these sets to find the following. Identify any disjoint sets. -Let $U = \{ 4,5,6,7,8,9,10,11,12,13,14 \} , M = \{ 4,6,8,10 \} , N = \{ 5,7,9,11,13 \} , Q = \{ 4,6,8,10,12,14 \}$ , and $\mathrm { R } = \{ 4,5,6,7 \}$ . $( \mathrm { R } \cup \mathrm { N } ) \cap \mathrm { M } ^ { \prime }$

Solve the problem. - $\frac { 64 s t ^ { 6 } - 10 t ^ { 10 } + 256 s t ^ { 5 } } { 8 s t ^ { 5 } }$

Identify the property illustrated by the statement. Assume all variables represent real numbers. -2 + 7 = 7 + 2

Provide an appropriate response. -Consider the following figure, which is a square divided into two squares and two rectangles. 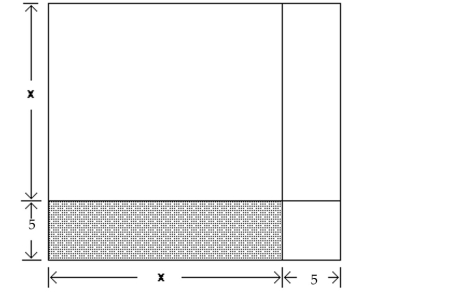The length of each side of the large square is $x + 5$ , which means that the area of the largest square is $( x + 5 ) ^ { 2 }$ . Use the formulas for the area of a square and the area of a rectangle to write the area of the largest square as a trinomial that represents the sum of the areas of the four figures that comprise it.

Factor the polynomial by substitution. - $a ^ { 2 } ( a + b ) ^ { 2 } - a b ( a + b ) ^ { 2 } - 30 b ^ { 2 } ( a + b ) ^ { 2 }$