Question 1

Solve the inequality.
-$30 \left( x ^ { 2 } - 1 \right) > 11 x$

Accepted Answer

A)  $\left( - \infty , - \frac { 5 } { 6 } \right)$ or $\left( \frac { 6 } { 5 } , \infty \right)$ 
B)  $\left( - \frac { 6 } { 5 } , \frac { 5 } { 6 } \right)$ 
C)  $\left( - \infty , - \frac { 6 } { 5 } \right)$ or $\left( \frac { 5 } { 6 } , \infty \right)$ 
D)  $\left( - \frac { 5 } { 6 } , \frac { 6 } { 5 } \right)$ 
A)  $\left( - \infty , - \frac { 5 } { 6 } \right)$ or $\left( \frac { 6 } { 5 } , \infty \right)$ 
B)  $\left( - \frac { 6 } { 5 } , \frac { 5 } { 6 } \right)$ 
C)  $\left( - \infty , - \frac { 6 } { 5 } \right)$ or $\left( \frac { 5 } { 6 } , \infty \right)$ 
D)  $\left( - \frac { 5 } { 6 } , \frac { 6 } { 5 } \right)$

Question 2

Without solving, determine the character of the solutions of the equation.
-$$x ^ { 2 } - 8 x + 16 = 0$$

Accepted Answer

A)  a repeated real solution 
B)  two complex solutions that are conjugates of each other 
C)  two unequal real solutions 
A)  a repeated real solution 
B)  two complex solutions that are conjugates of each other 
C)  two unequal real solutions

Question 3

Find the zeros of the quadratic function using the Square Root Method. List the x-intercepts of the graph of the function.
-$$h ( x ) = ( x + 4 ) ^ { 2 } - 16$$

Accepted Answer

A)  $x = - 8 , x = 0$ 
B)  $x = - 8$ 
C)  $x = - 4 , x = 4$ 
D)  $x = 0$ 
A)  $x = - 8 , x = 0$ 
B)  $x = - 8$ 
C)  $x = - 4 , x = 4$ 
D)  $x = 0$

Question 4

Solve the problem.
-As part of a physics experiment, Ming drops a baseball from the top of a 310-foot building. To the nearest tenth of a second, for how many seconds will the baseball fall? (Hint: Use the formula h = 16t2, which gives the
Distance h, in feet, that a free-falling object travels in t seconds.)

Accepted Answer

A)  19.4 sec 
B)  1.1 sec 
C)  4.4 sec 
D)  77.5 sec 
A)  19.4 sec 
B)  1.1 sec 
C)  4.4 sec 
D)  77.5 sec

Question 5

Line of best fit = -0.68x + 82.91
Choose the one alternative that best completes the statement or answers the question.
-Super Sally, a truly amazing individual, picks up a rock and throws it as hard as she can. The table below displays the relationship between the rock's horizontal distance, d (in feet) from Sally and the initial speed with which she throws. $$\begin{array} { l | c c c c c } 
\text { Initial speed( in ft/sec), v } & 10 & 15 & 20 & 25 & 30 \
\hline \text { Horizontal distance of the rock (in feet), d } & 9.9 & 14.8 & 19.1 & 24.5 & 28.2
\end{array}$$ Assume that the horizontal distance travelled varies linearly with the speed with which the rock is thrown. Using a
Graphing utility, find the line of best fit, and estimate, rounded to two decimal places, the horizontal distance of the rock
If the initial speed is 33 ft/sec.

Accepted Answer

A)  31.34 feet 
B)  26.67 feet 
C)  31.33 feet 
D)  34.76 feet 
A)  31.34 feet 
B)  26.67 feet 
C)  31.33 feet 
D)  34.76 feet

Question 6

Solve the problem.
-A coin is tossed upward from a balcony $390 \mathrm { ft }$ high with an initial velocity of $16 \mathrm { ft } / \mathrm { sec }$. During what interval of time will the coin be at a height of at least $70 \mathrm { ft }$ ?
$\left( h = - 16 t ^ { 2 } + v _ { O } t + h _ { O } \cdot \right)$

Accepted Answer

A)  $0 \leq t \leq 1$ 
B)  $0 \leq t \leq 5$ 
C)  $4 \leq \mathrm { t } \leq 5$ 
D)  $5 \leq t \leq 10$ 
A)  $0 \leq t \leq 1$ 
B)  $0 \leq t \leq 5$ 
C)  $4 \leq \mathrm { t } \leq 5$ 
D)  $5 \leq t \leq 10$

Question 7

Use a graphing utility to find the equation of the line of best fit. Round to two decimal places, if necessary.
-$\begin{array}{c|ccccc}
\mathrm{x} & 2 & 3 & 7 & 8 & 10 \
\hline \mathrm{y} & 2 & 4 & 4 & 6 & 6
\end{array}$

Accepted Answer

A)  $y = - 1.86 x + 1.79$ 
B)  $y = 1.79 x + 0.43$ 
C)  $y = 1.79 x - 1.86$ 
D)  $y = 0.43 x + 1.79$ 
A)  $y = - 1.86 x + 1.79$ 
B)  $y = 1.79 x + 0.43$ 
C)  $y = 1.79 x - 1.86$ 
D)  $y = 0.43 x + 1.79$

Question 8

Use a graphing utility to find the equation of the line of best fit. Round to two decimal places, if necessary.
-$\begin{array}{c|cccccc}
\mathrm{x} & 1 & 2 & 3 & 4 & 5 & 6 \
\hline \mathrm{y} & 17 & 20 & 19 & 22 & 21 & 24
\end{array}$

Accepted Answer

A)  $y = 1.17 x + 16.4$ 
B)  $y = 1.03 x + 16.4$ 
C)  $y = 1.03 x + 18.9$ 
D)  $y = 1.17 x + 18.9$ 
A)  $y = 1.17 x + 16.4$ 
B)  $y = 1.03 x + 16.4$ 
C)  $y = 1.03 x + 18.9$ 
D)  $y = 1.17 x + 18.9$

Question 9

Solve the inequality. Express your answer using interval notation. Graph the solution set.
-$$| 8 k - 9 | + 4 < 8$$

Accepted Answer

A)  $\left( - \infty , \frac { 5 } { 8 } \right)$
  
B)  $\left( \frac { 5 } { 8 } , \frac { 13 } { 8 } \right)$
  
C)  $\left( - \infty , \frac { 5 } { 8 } \right) \cup \left( \frac { 13 } { 8 } , \infty \right)$
  
D)  $\left( - \infty , \frac { 13 } { 8 } \right)$
  
A)  $\left( - \infty , \frac { 5 } { 8 } \right)$
  
B)  $\left( \frac { 5 } { 8 } , \frac { 13 } { 8 } \right)$
  
C)  $\left( - \infty , \frac { 5 } { 8 } \right) \cup \left( \frac { 13 } { 8 } , \infty \right)$
  
D)  $\left( - \infty , \frac { 13 } { 8 } \right)$

Question 10

Solve the problem.
-The owner of a video store has determined that the cost $\mathrm { C }$, in dollars, of operating the store is approximately given by $C ( x ) = 2 x ^ { 2 } - 32 x + 530$, where $x$ is the number of videos rented daily. Find the lowest cost to the nearest dollar.

Accepted Answer

A)  $\$ 658$ 
B)  $\$ 274$ 
C)  $\$ 402$ 
D)  $\$ 18$ 
A)  $\$ 658$ 
B)  $\$ 274$ 
C)  $\$ 402$ 
D)  $\$ 18$

Question 11

Solve the problem.
-If $f ( x ) = 6 x ^ { 2 } - 5 x$ and $g ( x ) = 2 x + 3$, solve for $f ( x ) = g ( x )$

Accepted Answer

A)  $\left\{ \frac { 1 } { 6 } , 1 \right\}$ 
B)  $\left\{ \frac { 3 } { 2 } , - \frac { 1 } { 3 } \right\}$ 
C)  $\left\{ - \frac { 1 } { 2 } , 1 \right\}$ 
D)  $\left\{ \frac { 1 } { 3 } , - \frac { 3 } { 2 } \right\}$ 
A)  $\left\{ \frac { 1 } { 6 } , 1 \right\}$ 
B)  $\left\{ \frac { 3 } { 2 } , - \frac { 1 } { 3 } \right\}$ 
C)  $\left\{ - \frac { 1 } { 2 } , 1 \right\}$ 
D)  $\left\{ \frac { 1 } { 3 } , - \frac { 3 } { 2 } \right\}$

Question 12

Solve the problem.
-The price $p$ (in dollars) and the quantity $x$ sold of a certain product obey the demand equation
$$p = - 10 x + 240 , \quad 0 \leq x \leq 24 \text {. }$$
What price should the company charge to maximize revenue?

Accepted Answer

A)  \$6 
B)  $\$ 18$ 
C)  $\$ 14.4$ 
D)  $\$ 12$ 
A)  \$6 
B)  $\$ 18$ 
C)  $\$ 14.4$ 
D)  $\$ 12$

Question 13

Graph the function using its vertex, axis of symmetry, and intercepts.
-$f(x)=-x^{2}-2 x+8$

Accepted Answer

A) 
vertex $ (-1,9) $
intercepts $ (2,0),(-4,0),(0,8) $
  
B) 
vertex $ (1,-9) $
intercepts $ (-2,0),(4,0),(0,-8) $
  
C) 
vertex $ (-1,-9) $
intercepts $ (2,0),(-4,0),(0,-8) $
  
D)  vertex $( 1,9 )$
intercepts $( - 2,0 ) , ( 4,0 ) , ( 0,8 )$
  
A) 
vertex $ (-1,9) $
intercepts $ (2,0),(-4,0),(0,8) $
  
B) 
vertex $ (1,-9) $
intercepts $ (-2,0),(4,0),(0,-8) $
  
C) 
vertex $ (-1,-9) $
intercepts $ (2,0),(-4,0),(0,-8) $
  
D)  vertex $( 1,9 )$
intercepts $( - 2,0 ) , ( 4,0 ) , ( 0,8 )$

Question 14

Solve the problem.
-If an object is dropped off of a tower, the velocity, $V$, of the object after $t$ seconds can be obtained by multiplying $t$ by 32 and adding 10 to the result. Express $\mathrm { V }$ as a linear function of $\mathrm { t }$.

Accepted Answer

A)  $V ( t ) = 32 t + 10$ 
B)  $V ( t ) = 42 t$ 
C)  $V ( t ) = \frac { t - 10 } { 32 }$ 
D)  $V ( t ) = 32 + 10 t$ 
A)  $V ( t ) = 32 t + 10$ 
B)  $V ( t ) = 42 t$ 
C)  $V ( t ) = \frac { t - 10 } { 32 }$ 
D)  $V ( t ) = 32 + 10 t$

Question 15

Solve the problem.
-A rock falls from a tower that is $208 \mathrm { ft }$ high. As it is falling, its height is given by the formula $\mathrm { h } = 208 - 16 \mathrm { t } ^ { 2 }$. How many seconds will it take for the rock to hit the ground $( h = 0 )$ ?

Accepted Answer

A)  $13.9 \mathrm {~s}$ 
B)  $2,704 \mathrm {~s}$ 
C)  $14.4 \mathrm {~s}$ 
D)  $3.6 \mathrm {~s}$ 
A)  $13.9 \mathrm {~s}$ 
B)  $2,704 \mathrm {~s}$ 
C)  $14.4 \mathrm {~s}$ 
D)  $3.6 \mathrm {~s}$

Question 16

Graph the function using its vertex, axis of symmetry, and intercepts.
-$$f ( x ) = - 3 x ^ { 2 } - 2 x - 10$$

Accepted Answer

A)  vertex $\left( - \frac { 1 } { 3 } , - \frac { 29 } { 3 } \right)$
 intercept $( 0 , - 10 )$
  
B)  vertex $\left( \frac { 1 } { 3 } 
, - \frac { 29 } { 3 } \right)$
intercept $( 0 , - 10 )$
  
C)  vertex $\left( \frac { 1 } { 3 } , \frac { 29 } { 3 } \right)$
intercept $( 0,10 )$
  
D)  vertex $\left( - \frac { 1 } { 3 } , \frac { 29 } { 3 } \right)$ 
intercept $( 0,10 )$
  
A)  vertex $\left( - \frac { 1 } { 3 } , - \frac { 29 } { 3 } \right)$
 intercept $( 0 , - 10 )$
  
B)  vertex $\left( \frac { 1 } { 3 } 
, - \frac { 29 } { 3 } \right)$
intercept $( 0 , - 10 )$
  
C)  vertex $\left( \frac { 1 } { 3 } , \frac { 29 } { 3 } \right)$
intercept $( 0,10 )$
  
D)  vertex $\left( - \frac { 1 } { 3 } , \frac { 29 } { 3 } \right)$ 
intercept $( 0,10 )$

Question 17

Determine the domain and the range of the function.
-$$f ( x ) = x ^ { 2 } - 2 x - 8$$

Accepted Answer

A)  domain: all real numbers
range: all real numbers 
B)  domain: $\{ x \mid x \geq - 1 \}$  
range: $\{ y \mid y \geq - 9 \}$ 
C)  domain: all real numbers
 range: $\{ y \mid y \geq - 9 \}$ 
D)  domain: all real numbers 
range: $\{ \mathrm { y } \mid \mathrm { y } \leq 9 \}$ 
A)  domain: all real numbers
range: all real numbers 
B)  domain: $\{ x \mid x \geq - 1 \}$  
range: $\{ y \mid y \geq - 9 \}$ 
C)  domain: all real numbers
 range: $\{ y \mid y \geq - 9 \}$ 
D)  domain: all real numbers 
range: $\{ \mathrm { y } \mid \mathrm { y } \leq 9 \}$

Question 18

Solve the inequality.
-$$\mathrm { x } ^ { 2 } + 6 \mathrm { x } \leq 0$$

Accepted Answer

A)  $[ - 6,0 ]$ 
B)  $[ 0,6 ]$ 
C)  $( - \infty , 0 ]$ or $[ 6 , \infty )$ 
D)  $( - \infty , - 6 ]$ or $[ 0 , \infty )$ 
A)  $[ - 6,0 ]$ 
B)  $[ 0,6 ]$ 
C)  $( - \infty , 0 ]$ or $[ 6 , \infty )$ 
D)  $( - \infty , - 6 ]$ or $[ 0 , \infty )$

Question 19

Determine the slope and y-intercept of the function.
-p(x) = -x - 3

Accepted Answer

A)  m =1; b = 3 
B)  m = -1; b = 3 
C)  m = 0; b = -3 
D)  m = -1; b =-3 
A)  m =1; b = 3 
B)  m = -1; b = 3 
C)  m = 0; b = -3 
D)  m = -1; b =-3

Question 20

Determine where the function is increasing and where it is decreasing.
-$$f ( x ) = x ^ { 2 } + 4 x + 4$$

Accepted Answer

A)  increasing on $( 2 , \infty )$
 decreasing on $( - \infty , 2 )$ 
B)  increasing on $( - 2 , \infty )$
decreasing on $( - \infty , - 2 )$ 
C)  increasing on $( - \infty , - 2 )$
decreasing on $( - 2 , \infty )$ 
D)  increasing on $( - \infty , 2 )$ 
decreasing on $( 2 , \infty )$ 
A)  increasing on $( 2 , \infty )$
 decreasing on $( - \infty , 2 )$ 
B)  increasing on $( - 2 , \infty )$
decreasing on $( - \infty , - 2 )$ 
C)  increasing on $( - \infty , - 2 )$
decreasing on $( - 2 , \infty )$ 
D)  increasing on $( - \infty , 2 )$ 
decreasing on $( 2 , \infty )$

Solve the inequality. - $30 \left( x ^ { 2 } - 1 \right) > 11 x$

Without solving, determine the character of the solutions of the equation. - $x ^ { 2 } - 8 x + 16 = 0$

Find the zeros of the quadratic function using the Square Root Method. List the x-intercepts of the graph of the function. - $h ( x ) = ( x + 4 ) ^ { 2 } - 16$

Use a graphing utility to find the equation of the line of best fit. Round to two decimal places, if necessary. - 2 3 7 8 10 2 4 4 6 6

Use a graphing utility to find the equation of the line of best fit. Round to two decimal places, if necessary. - 1 2 3 4 5 6 17 20 19 22 21 24

Solve the inequality. Express your answer using interval notation. Graph the solution set. - $| 8 k - 9 | + 4 < 8$

Solve the problem. -The owner of a video store has determined that the cost $\mathrm { C }$ , in dollars, of operating the store is approximately given by $C ( x ) = 2 x ^ { 2 } - 32 x + 530$ , where $x$ is the number of videos rented daily. Find the lowest cost to the nearest dollar.

Solve the problem. -If $f ( x ) = 6 x ^ { 2 } - 5 x$ and $g ( x ) = 2 x + 3$ , solve for $f ( x ) = g ( x )$

Solve the problem. -The price $p$ (in dollars) and the quantity $x$ sold of a certain product obey the demand equation $p = - 10 x + 240 , \quad 0 \leq x \leq 24 \text {. }$ What price should the company charge to maximize revenue?

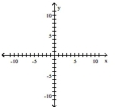
Graph the function using its vertex, axis of symmetry, and intercepts. - $f(x)=-x^{2}-2 x+8$ $Graph the function using its vertex, axis of symmetry, and intercepts. - f(x)=-x^{2}-2 x+8$

Solve the problem. -If an object is dropped off of a tower, the velocity, $V$ , of the object after $t$ seconds can be obtained by multiplying $t$ by 32 and adding 10 to the result. Express $\mathrm { V }$ as a linear function of $\mathrm { t }$ .

Solve the problem. -A rock falls from a tower that is $208 \mathrm { ft }$ high. As it is falling, its height is given by the formula $\mathrm { h } = 208 - 16 \mathrm { t } ^ { 2 }$ . How many seconds will it take for the rock to hit the ground $( h = 0 )$ ?

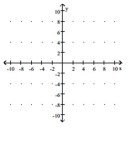
Graph the function using its vertex, axis of symmetry, and intercepts. - $f ( x ) = - 3 x ^ { 2 } - 2 x - 10$ $Graph the function using its vertex, axis of symmetry, and intercepts. - f ( x ) = - 3 x ^ { 2 } - 2 x - 10$

Determine the domain and the range of the function. - $f ( x ) = x ^ { 2 } - 2 x - 8$

Solve the inequality. - $\mathrm { x } ^ { 2 } + 6 \mathrm { x } \leq 0$

Determine the slope and y-intercept of the function. -p(x) = -x - 3

Determine where the function is increasing and where it is decreasing. - $f ( x ) = x ^ { 2 } + 4 x + 4$

Functions and Their Graphs

Polynomial and Rational Functions

Exponential and Logarithmic Functions

Trigonometric Functions

Analytic Trigonometry

Applications of Trigonometric Functions

Polar Coordinates; Vectors

Analytic Geometry

Systems of Equations and Inequalities

Sequences; Induction; the Binomial Theorem

Counting and Probability

A Preview of Calculus: the Limit, Derivative, and Integral of a Function

Foundations: a Prelude to Functions

Graphing Utilities

Filters

Exam 2: Linear and Quadratic Functions

Solve the inequality. - 30(x2−1)>11x30 \left( x ^ { 2 } - 1 \right) > 11 x30(x2−1)>11x

Without solving, determine the character of the solutions of the equation. - x2−8x+16=0x ^ { 2 } - 8 x + 16 = 0x2−8x+16=0

Find the zeros of the quadratic function using the Square Root Method. List the x-intercepts of the graph of the function. - h(x)=(x+4)2−16h ( x ) = ( x + 4 ) ^ { 2 } - 16h(x)=(x+4)2−16