Question 1

Determine, without graphing, whether the given quadratic function has a maximum value or a minimum value and then
find that value.
-$$f ( x ) = x ^ { 2 } + 2 x - 6$$

Accepted Answer

A)  minimum; - 7 
B)  maximum; - 1 
C)  maximum; - 7 
D)  minimum; - 1 
A)  minimum; - 7 
B)  maximum; - 1 
C)  maximum; - 7 
D)  minimum; - 1

Question 2

Determine, without graphing, whether the given quadratic function has a maximum value or a minimum value and then
find that value.
-$$f ( x ) = - x ^ { 2 } - 2 x - 8$$

Accepted Answer

A)  maximum; - 1 
B)  minimum; - 7 
C)  maximum; - 7 
D)  minimum; - 1 
A)  maximum; - 1 
B)  minimum; - 7 
C)  maximum; - 7 
D)  minimum; - 1

Question 3

Determine where the function is increasing and where it is decreasing.
-$$f ( x ) = x ^ { 2 } - 4 x + 3$$

Accepted Answer

A)  increasing on $( - 1 , \infty )$
decreasing on $( - \infty , - 1 )$ 
B)  increasing on $( - \infty , - 1 )$ 
decreasing on $( - 1 , \infty )$ 
C)  increasing on $( - \infty , 2 )$
decreasing on $( 2 , \infty )$ 
D)  increasing on $( 2 , \infty )$ 
decreasing on $( - \infty , 2 )$ 
A)  increasing on $( - 1 , \infty )$
decreasing on $( - \infty , - 1 )$ 
B)  increasing on $( - \infty , - 1 )$ 
decreasing on $( - 1 , \infty )$ 
C)  increasing on $( - \infty , 2 )$
decreasing on $( 2 , \infty )$ 
D)  increasing on $( 2 , \infty )$ 
decreasing on $( - \infty , 2 )$

Question 4

Write the word or phrase that best completes each statement or answers the question.
Solve the problem.
-The one-day temperatures for 12 world cities along with their latitudes are shown in the table below. Make a
scatter diagram for the data. Then find the line of best fit and graph it on the scatter diagram. $$\begin{array}{l}
\begin{array} { c | c | c } 
\text { City } & \text { Temperature (F) } & \text { Latitude } \
\hline \text { Oslo, Norway } & 30 ^ { \circ } & 59 ^ { \circ } \
\text { Seattle, WA } & 57 ^ { \circ } & 47 ^ { \circ } \
\text { Anchorage, AK } & 40 ^ { \circ } & 61 ^ { \circ } \
\text { Paris, France } & 61 ^ { \circ } & 48 ^ { \circ } \
\text { Vancouver, Canada } & 54 ^ { \circ } & 49 ^ { \circ } \
\text { London, England } & 48 ^ { \circ } & 51 ^ { \circ } \
\text { Tokyo, Japan } & 55 ^ { \circ } & 35 ^ { \circ } \
\text { Cairo, Egypt } & 82 ^ { \circ } & 30 ^ { \circ } \
\text { Mexico City, Mexico } & 84 ^ { \circ } & 19 ^ { \circ } \
\text { Miami, FL } & 81 ^ { \circ } & 25 ^ { \circ } \
\text { New Delhi, India } & 95 ^ { \circ } & 28 ^ { \circ } \
\text { Manila, Philippines } & 93 ^ { \circ } & 14 ^ { \circ }
\end{array}\
\text { Latitude (degrees) }
\end{array}$$  
$$\text { Temperature } ( \mathrm { F } ) ^ { \circ }$$

Accepted Answer

The answer of Write the word or phrase that best...

Question 5

Line of best fit = -0.68x + 82.91
Choose the one alternative that best completes the statement or answers the question.
-A marina owner wishes to estimate a linear function that relates boat length in feet and its draft (depth of boat below water line) in feet. He collects the following data. Let boat length represent the independent variable and
Draft represent the dependent variable. Use a graphing utility to draw a scatter diagram and to find the line of
Best fit. What is the draft for a boat 60 ft in length (to the nearest tenth)? $\begin{array}{l|l}
\text { Boat Length (ft) } & \text { Draft (ft) } \
\hline 25 & 2.5 \
25 & 2 \
30 & 3 \
30 & 3.5 \
45 & 6 \
45 & 7 \
50 & 7 \
50 & 8
\end{array}$

Accepted Answer

A)  $10.3$ 
B)  $9.7$ 
C)  $15.7$ 
D)  $10.5$ 
A)  $10.3$ 
B)  $9.7$ 
C)  $15.7$ 
D)  $10.5$

Question 6

Determine the domain and the range of the function.
-$$f ( x ) = x ^ { 2 } - 12 x + 36$$

Accepted Answer

A)  domain: all real numbers
range: $\{ y \mid y \geq 0 \}$ 
B)  domain: all real numbers 
range: $\{ y \mid y \geq 36 \}$ 
C)  domain: $\{ x \mid x \geq - 6 \}$
range: $\{ y \mid y \geq 0 \}$ 
D)  domain: $\{ x \mid x \geq 6 \}$
range: $\left\{ y ^ { \mid } y \geq 0 \right\}$ 
A)  domain: all real numbers
range: $\{ y \mid y \geq 0 \}$ 
B)  domain: all real numbers 
range: $\{ y \mid y \geq 36 \}$ 
C)  domain: $\{ x \mid x \geq - 6 \}$
range: $\{ y \mid y \geq 0 \}$ 
D)  domain: $\{ x \mid x \geq 6 \}$
range: $\left\{ y ^ { \mid } y \geq 0 \right\}$

Question 7

Use a graphing calculator to plot the data and find the quadratic function of best fit.
-The number of housing starts in one beachside community remained fairly level until 1992 and then began to increase. The following data shows the number of housing starts since 1992 (x = 1). Use a graphing calculator to
Plot a scatter diagram. What is the quadratic function of best fit? $\begin{array}{l|l}
\text { Year, x } & \text { Housing Starts, H } \
\hline 1 & 200 \
2 & 210 \
3 & 230 \
4 & 240 \
5 & 250 \
6 & 230 \
7 & 215 \
8 & 208
\end{array}$

Accepted Answer

A)  $\mathrm { H } ( \mathrm { x } ) = - 3.268 \mathrm { x } ^ { 2 } - 30.494 \mathrm { x } + 168.982$ 
B)  $\mathrm { H } ( \mathrm { x } ) = - 3.268 \mathrm { x } ^ { 2 } + 30.494 \mathrm { x } - 168.982$ 
C)  $\mathrm { H } ( \mathrm { x } ) = 3.268 \mathrm { x } ^ { 2 } + 30.494 \mathrm { x } + 168.982$ 
D)  $\mathrm { H } ( \mathrm { x } ) = - 3.268 \mathrm { x } ^ { 2 } + 30.494 \mathrm { x } + 168.982$ 
A)  $\mathrm { H } ( \mathrm { x } ) = - 3.268 \mathrm { x } ^ { 2 } - 30.494 \mathrm { x } + 168.982$ 
B)  $\mathrm { H } ( \mathrm { x } ) = - 3.268 \mathrm { x } ^ { 2 } + 30.494 \mathrm { x } - 168.982$ 
C)  $\mathrm { H } ( \mathrm { x } ) = 3.268 \mathrm { x } ^ { 2 } + 30.494 \mathrm { x } + 168.982$ 
D)  $\mathrm { H } ( \mathrm { x } ) = - 3.268 \mathrm { x } ^ { 2 } + 30.494 \mathrm { x } + 168.982$

Question 8

Solve the problem.
-The profit that the vendor makes per day by selling $x$ pretzels is given by the function $P ( x ) = - 0.002 x ^ { 2 } + 1.6 x - 350$. Find the number of pretzels that must be sold to maximize profit.

Accepted Answer

A)  $0.8$ pretzels 
B)  $- 30$ pretzels 
C)  800 pretzels 
D)  400 pretzels 
A)  $0.8$ pretzels 
B)  $- 30$ pretzels 
C)  800 pretzels 
D)  400 pretzels

Question 9

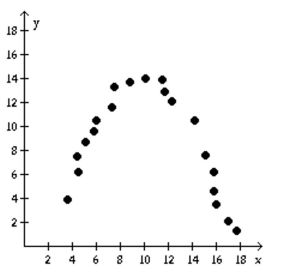
Determine if the type of relation is linear, nonlinear, or none.
-

Accepted Answer

A)  none 
B)  linear 
C)  nonlinear 
A)  none 
B)  linear 
C)  nonlinear

Question 10

Determine, without graphing, whether the given quadratic function has a maximum value or a minimum value and then
find that value.
-$$f ( x ) = 4 x ^ { 2 } + 12 x$$

Accepted Answer

A)  minimum; $- \frac { 3 } { 2 }$ 
B)  minimum; $- 9$ 
C)  maximum; $- \frac { 3 } { 2 }$ 
D)  maximum; $- 9$ 
A)  minimum; $- \frac { 3 } { 2 }$ 
B)  minimum; $- 9$ 
C)  maximum; $- \frac { 3 } { 2 }$ 
D)  maximum; $- 9$

Question 11

employees each year.
Choose the one alternative that best completes the statement or answers the question.
Use factoring to find the zeros of the quadratic function. List the x-intercepts of the graph of the function.
-$$G ( x ) = x ^ { 2 } - 5 x$$

Accepted Answer

A)  $x = - 5$ 
B)  $x = 0 , x = - 5$ 
C)  $x = 5$ 
D)  $x = 0 , x = 5$ 
A)  $x = - 5$ 
B)  $x = 0 , x = - 5$ 
C)  $x = 5$ 
D)  $x = 0 , x = 5$

Question 12

Determine where the function is increasing and where it is decreasing.
-$$f ( x ) = x ^ { 2 } + 4 x + 3$$

Accepted Answer

A)  increasing on $( - \infty , - 1 )$
 decreasing on $( - 1 , \infty )$ 
B)  increasing on $( - 1 , \infty )$
decreasing on $( - \infty , - 1 )$ 
C)  increasing on $( - 2 , \infty )$ 
D)  increasing on $( - \infty , - 2 )$

decreasing on $( - \infty , - 2 )$
decreasing on $( - 2 , \infty )$ 
A)  increasing on $( - \infty , - 1 )$
 decreasing on $( - 1 , \infty )$ 
B)  increasing on $( - 1 , \infty )$
decreasing on $( - \infty , - 1 )$ 
C)  increasing on $( - 2 , \infty )$ 
D)  increasing on $( - \infty , - 2 )$

decreasing on $( - \infty , - 2 )$
decreasing on $( - 2 , \infty )$

Question 13

Match the graph to one of the listed functions.
-

Accepted Answer

A)  $f ( x ) = x ^ { 2 } + 2 x - 1$ 
B)  $f ( x ) = x ^ { 2 } - 2 x$ 
C)  $f ( x ) = x ^ { 2 } + 2 x$ 
D)  $f ( x ) = x ^ { 2 } - 2 x - 1$ 
A)  $f ( x ) = x ^ { 2 } + 2 x - 1$ 
B)  $f ( x ) = x ^ { 2 } - 2 x$ 
C)  $f ( x ) = x ^ { 2 } + 2 x$ 
D)  $f ( x ) = x ^ { 2 } - 2 x - 1$

Question 14

Find the zeros of the quadratic function by completing the square. List the x-intercepts of the graph of the function.
-$$F ( x ) = x ^ { 2 } + 6 x - 40$$

Accepted Answer

A)  $x = \sqrt { - 40 } , x = \sqrt { 40 }$ 
B)  $x = - 30 , x = - 10$ 
C)  $x = - 4 , x = 10$ 
D)  $x = 4 , x = - 10$ 
A)  $x = \sqrt { - 40 } , x = \sqrt { 40 }$ 
B)  $x = - 30 , x = - 10$ 
C)  $x = - 4 , x = 10$ 
D)  $x = 4 , x = - 10$

Question 15

Find the zeros of the quadratic function by completing the square. List the x-intercepts of the graph of the function.
-$$f ( x ) = x ^ { 2 } - \frac { 2 } { 5 } x - \frac { 8 } { 25 }$$

Accepted Answer

A)  $x = - \frac { 4 } { 5 } , x = - \frac { 2 } { 5 }$ 
B)  $x = \frac { 4 } { 5 } , x = \frac { 2 } { 5 }$ 
C)  $x = - \frac { 4 } { 5 } , x = \frac { 2 } { 5 }$ 
D)  $x = \frac { 4 } { 5 } , x = - \frac { 2 } { 5 }$ 
A)  $x = - \frac { 4 } { 5 } , x = - \frac { 2 } { 5 }$ 
B)  $x = \frac { 4 } { 5 } , x = \frac { 2 } { 5 }$ 
C)  $x = - \frac { 4 } { 5 } , x = \frac { 2 } { 5 }$ 
D)  $x = \frac { 4 } { 5 } , x = - \frac { 2 } { 5 }$

Question 16

Use the slope and y-intercept to graph the linear function.
-$$\mathrm { F } ( \mathrm { x } ) = 2$$

Accepted Answer

A) 
  
B) 
  
C) 
  
D) 
  
A) 
  
B) 
  
C) 
  
D)

Question 17

employees each year.
Choose the one alternative that best completes the statement or answers the question.
Use factoring to find the zeros of the quadratic function. List the x-intercepts of the graph of the function.
-$$f ( x ) = x ^ { 2 } + 6 x - 40$$

Accepted Answer

A)  $x = - 10 , x = 1$ 
B)  $x = 10 , x = - 4$ 
C)  $x = 10 , x = 4$ 
D)  $x = - 10 , x = 4$ 
A)  $x = - 10 , x = 1$ 
B)  $x = 10 , x = - 4$ 
C)  $x = 10 , x = 4$ 
D)  $x = - 10 , x = 4$

Question 18

Solve the inequality. Express your answer using interval notation. Graph the solution set.
-$| 6 x | < 24$

Accepted Answer

A)  $( - \infty , - 4 )$ Or $( 4 , \infty )$
  
B)  $( - 4,4 )$
  
C) $( - 4,4 )$
  
D)  $( - \infty , 4 )$
  
A)  $( - \infty , - 4 )$ Or $( 4 , \infty )$
  
B)  $( - 4,4 )$
  
C) $( - 4,4 )$
  
D)  $( - \infty , 4 )$

Question 19

Solve the problem.
-A projectile is fired from a cliff 300 feet above the water at an inclination of 45° to the horizontal, with a muzzle velocity of 300 feet per second. The height h of the projectile above the water is given by $$h ( x ) = \frac { - 32 x ^ { 2 } } { ( 300 ) ^ { 2 } } + x + 300$$ where x is the horizontal distance of the projectile from the base of the cliff. How far from the base of the cliff is
The height of the projectile a maximum?

Accepted Answer

A)  1,003.13 ft 
B)  1,406.25 ft 
C)  703.13 ft 
D)  2,409.38 ft 
A)  1,003.13 ft 
B)  1,406.25 ft 
C)  703.13 ft 
D)  2,409.38 ft

Question 20

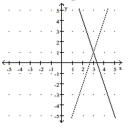
Solve the problem.
-Let $f ( x )$ be the function represented by the dashed line and $g ( x )$ be the function represented by the solid lin Solve the equation $f ( x ) = g ( x )$.

Accepted Answer

A)  x = -3 
B)  x = -1 
C)  x = 1 
D)  x = 3 
A)  x = -3 
B)  x = -1 
C)  x = 1 
D)  x = 3

Determine, without graphing, whether the given quadratic function has a maximum value or a minimum value and then find that value. - $f ( x ) = x ^ { 2 } + 2 x - 6$

Determine, without graphing, whether the given quadratic function has a maximum value or a minimum value and then find that value. - $f ( x ) = - x ^ { 2 } - 2 x - 8$

Determine where the function is increasing and where it is decreasing. - $f ( x ) = x ^ { 2 } - 4 x + 3$

Determine the domain and the range of the function. - $f ( x ) = x ^ { 2 } - 12 x + 36$

Solve the problem. -The profit that the vendor makes per day by selling $x$ pretzels is given by the function $P ( x ) = - 0.002 x ^ { 2 } + 1.6 x - 350$ . Find the number of pretzels that must be sold to maximize profit.

Determine if the type of relation is linear, nonlinear, or none. -

Determine, without graphing, whether the given quadratic function has a maximum value or a minimum value and then find that value. - $f ( x ) = 4 x ^ { 2 } + 12 x$

employees each year. Choose the one alternative that best completes the statement or answers the question. Use factoring to find the zeros of the quadratic function. List the x-intercepts of the graph of the function. - $G ( x ) = x ^ { 2 } - 5 x$

Determine where the function is increasing and where it is decreasing. - $f ( x ) = x ^ { 2 } + 4 x + 3$

Match the graph to one of the listed functions. -

Find the zeros of the quadratic function by completing the square. List the x-intercepts of the graph of the function. - $F ( x ) = x ^ { 2 } + 6 x - 40$

Find the zeros of the quadratic function by completing the square. List the x-intercepts of the graph of the function. - $f ( x ) = x ^ { 2 } - \frac { 2 } { 5 } x - \frac { 8 } { 25 }$

Use the slope and y-intercept to graph the linear function. - $\mathrm { F } ( \mathrm { x } ) = 2$ $Use the slope and y-intercept to graph the linear function. - \mathrm { F } ( \mathrm { x } ) = 2$

employees each year. Choose the one alternative that best completes the statement or answers the question. Use factoring to find the zeros of the quadratic function. List the x-intercepts of the graph of the function. - $f ( x ) = x ^ { 2 } + 6 x - 40$

Solve the inequality. Express your answer using interval notation. Graph the solution set. - $| 6 x | < 24$

Solve the problem. -Let $f ( x )$ be the function represented by the dashed line and $g ( x )$ be the function represented by the solid lin Solve the equation $f ( x ) = g ( x )$ .

Functions and Their Graphs

Polynomial and Rational Functions

Exponential and Logarithmic Functions

Trigonometric Functions

Analytic Trigonometry

Applications of Trigonometric Functions

Polar Coordinates; Vectors

Analytic Geometry

Systems of Equations and Inequalities

Sequences; Induction; the Binomial Theorem

Counting and Probability

A Preview of Calculus: the Limit, Derivative, and Integral of a Function

Foundations: a Prelude to Functions

Graphing Utilities

Filters

Exam 2: Linear and Quadratic Functions

Determine, without graphing, whether the given quadratic function has a maximum value or a minimum value and then find that value. - f(x)=x2+2x−6f ( x ) = x ^ { 2 } + 2 x - 6f(x)=x2+2x−6

Determine, without graphing, whether the given quadratic function has a maximum value or a minimum value and then find that value. - f(x)=−x2−2x−8f ( x ) = - x ^ { 2 } - 2 x - 8f(x)=−x2−2x−8

Determine where the function is increasing and where it is decreasing. - f(x)=x2−4x+3f ( x ) = x ^ { 2 } - 4 x + 3f(x)=x2−4x+3

Determine the domain and the range of the function. - f(x)=x2−12x+36f ( x ) = x ^ { 2 } - 12 x + 36f(x)=x2−12x+36

Solve the problem. -The profit that the vendor makes per day by selling xxx pretzels is given by the function P(x)=−0.002x2+1.6x−350P ( x ) = - 0.002 x ^ { 2 } + 1.6 x - 350P(x)=−0.002x2+1.6x−350 . Find the number of pretzels that must be sold to maximize profit.