Exam 9: Matrices and Determinants
Exam 1: Equations and Inequalities425 Questions
Exam 2: Functions and Graphs359 Questions
Exam 3: Polynomial and Rational Functions532 Questions
Exam 4: Exponential and Logarithmic Functions270 Questions
Exam 5: Trigonometric Functions386 Questions
Exam 6: Analytic Trigonometry226 Questions
Exam 7: Additional Topics in Trigonometry264 Questions
Exam 8: Systems of Equations and Inequalities288 Questions
Exam 9: Matrices and Determinants152 Questions
Exam 10: Conic Sections and Analytic Geometry228 Questions
Exam 11: Sequences, Induction, and Probability304 Questions
Exam 12: Prerequisites: Fundamental Concepts of Algebra409 Questions
Select questions type
Solve a System of Linear Equations in Two Variables Using Cramer's Rule
- 2x+3y=24 2x-3y=12
(Multiple Choice)
4.8/5  (35)
(35)
Encode and Decode Messages
-Use the coding matrix and its inverse to decode the cryptogram .
(Multiple Choice)
4.9/5  (33)
(33)
Solve Problems Involving Systems Without Unique Solutions
-The figure below shows the intersection of three one-way streets. To keep traffic moving, the number of cars per minute entering an intersection must equal the number of cars leaving that intersection. Set up a system of equations that keeps traffic moving, and use Gaussian elimination to solve the system. If construction limits z to t cars per minute, how many cars per minute must pass through the other intersections to keep traffic moving? 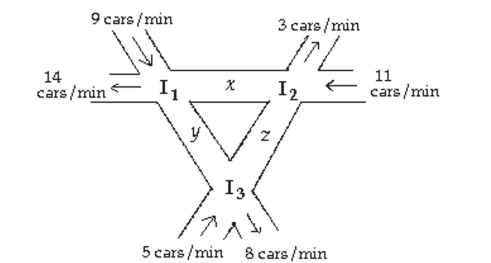
(Multiple Choice)
4.8/5  (37)
(37)
Solve a System of Linear Equations in Two Variables Using Cramer's Rule
-
(Multiple Choice)
4.9/5  (36)
(36)
Apply Gaussian Elimination to Systems with More Variables than Equations
- 5x-y+z=8 7x+y+z=6
(Multiple Choice)
4.8/5  (29)
(29)
Encode and Decode Messages
-Use the coding matrix and its inverse to decode the cryptogram .
(Multiple Choice)
4.9/5  (30)
(30)
Write a system of linear equations in three variables, and then use matrices to solve the system.
-The table below shows the number of birds for three selected years after an endangered species protection program was started. (Number of years after 1980) 1 5 10 (Number of birds) 43 139 349 Use the quadratic function to model the data. Solve the system of linear equations involving , and using matrices. Find the equation that models the data.
(Multiple Choice)
4.9/5  (34)
(34)
Multiplicative Inverses of Matrices and Matrix Equations
1 Find the Multiplicative Inverse of a Square Matrix
-
(Multiple Choice)
4.9/5  (40)
(40)
Multiplicative Inverses of Matrices and Matrix Equations
1 Find the Multiplicative Inverse of a Square Matrix
-
(Multiple Choice)
4.8/5  (39)
(39)
Solve the problem using matrices.
-State University has a College of Arts & Sciences, a College of Business, and a College of Engineering. The percentage of students in each category are given by the following matrix. ![Solve the problem using matrices. -State University has a College of Arts & Sciences, a College of Business, and a College of Engineering. The percentage of students in each category are given by the following matrix. The student population is distributed by class and age as given in the following matrix. \left. \begin{array} { l c c } & \text { Female } & \text { Male } \\ \text { Freshman } & 410 & 720 \\ \text { Sophomore } & 550 & 750 \\ \text { Junior } & 800 & 670 \\ \text { Senior } & 630 & 480 \end{array} \right] How many female students are in the College of Business? How many male students are in the College of Arts & Sciences?](https://storage.examlex.com/TB7044/11eb42be_2084_8fb9_8bfd_671c3575e8b5_TB7044_00.jpg) The student population is distributed by class and age as given in the following matrix. How many female students are in the College of Business? How many male students are in the College of Arts & Sciences?
The student population is distributed by class and age as given in the following matrix. How many female students are in the College of Business? How many male students are in the College of Arts & Sciences?
(Multiple Choice)
4.9/5  (34)
(34)
Simplify Complex Rational Expressions
- 2x+6y+9z=66 7x+6y+8z=77 9x+8y-2z=51
(Multiple Choice)
4.9/5  (35)
(35)
Multiplicative Inverses of Matrices and Matrix Equations
1 Find the Multiplicative Inverse of a Square Matrix
-
(Multiple Choice)
4.8/5  (28)
(28)
Use Inverses to Solve Matrix Equations
- 5x+7z=83 9y+6z=72 4x+7y-2z=12
(Multiple Choice)
4.7/5  (29)
(29)
Showing 101 - 120 of 152
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)