Exam 10: Analytic Geometry in Three Dimensions
Exam 1: Functions and Their Graphs120 Questions
Exam 2: Polynomial and Rational Functions120 Questions
Exam 3: Exponential and Logarithmic Functions120 Questions
Exam 4: Trigonometric Functions120 Questions
Exam 5: Analytic Trigonometry120 Questions
Exam 6: Additional Topics in Trigonometry120 Questions
Exam 7: Linear Systems and Matrices120 Questions
Exam 8: Sequences, Series, and Probability118 Questions
Exam 9: Topics in Analytic Geometry120 Questions
Exam 10: Analytic Geometry in Three Dimensions120 Questions
Exam 11: Limits and an Introduction to Calculus120 Questions
Select questions type
D Determine whether and are parallel, orthogonal, or neither.
(Multiple Choice)
4.7/5  (33)
(33)
Find the lengths of the sides of the right triangle whose vertices are located at the given points. Show that these lengths satisfy the Pythagorean Theorem. Show all of your work.
(Essay)
4.8/5  (32)
(32)
For the points :
a. Verify that the points are vertices of a parallelogram. Show all work.
b. Find the area of the parallelogram. Show all work.
c. Determine whether the parallelogram is a rectangle.
(Essay)
4.9/5  (42)
(42)
Find the lengths of the sides of the right triangle whose vertices are located at the given points. Show that these lengths satisfy the Pythagorean Theorem. Show all of your work.
(Essay)
4.9/5  (32)
(32)
Find the general form of the equation of the plane with the given characteristics. The plane passes through the points and and is perpendicular to the plane .
(Multiple Choice)
4.8/5  (30)
(30)
Find the torque on the crankshaft using the data shown in the figure. Round to the nearest tenth of a foot-pound.
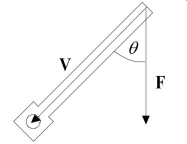 \|\|=1.6 \|\|=20 \theta=6
\|\|=1.6 \|\|=20 \theta=6
(Multiple Choice)
4.9/5  (28)
(28)
Find symmetric equations for the line through the point and parallel to the specified vector. Show all your work.
, parallel to
(Essay)
4.8/5  (32)
(32)
Find the area of the parallelogram that has the vectors as adjacent sides.
(Multiple Choice)
4.9/5  (28)
(28)
Find the area of the parallelogram that has the vectors as adjacent sides.
(Multiple Choice)
4.8/5  (27)
(27)
Determine whether and are parallel, orthogonal, or neither.
(Multiple Choice)
4.7/5  (28)
(28)
Write the component form of the vector described below.
Initial point:
Terminal point:
(Multiple Choice)
5.0/5  (24)
(24)
Find the area of the parallelogram that has the vectors as adjacent sides.
(Multiple Choice)
4.9/5  (35)
(35)
Find the lengths of the sides of the right triangle whose vertices are located at the given points. Show that these lengths satisfy the Pythagorean Theorem.
Show all of your work.
(Essay)
4.8/5  (35)
(35)
Find the angle between the vectors and . Express your answer in degrees and round to the nearest tenth of a degree.
(Multiple Choice)
4.8/5  (43)
(43)
Find the angle of intersection of the planes in degrees. Round to a tenth of a degree.
3x-2y+4z=3 5x-3y+3z=-6
(Multiple Choice)
5.0/5  (38)
(38)
Find the general form of the equation of the plane passing through the three points. [Be sure to reduce the coefficients in your answer to lowest terms by dividing out any common factor.]
(Multiple Choice)
4.9/5  (35)
(35)
Showing 41 - 60 of 120
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)