Exam 9: Estimation and Confidence Intervals
Exam 1: What Is Statistics79 Questions
Exam 2: Describing Data: Frequencydistributions and Graphic Presentation100 Questions
Exam 3: Describing Data: Numerical Measures214 Questions
Exam 4: Describing Data: Displaying and Exploring Pata138 Questions
Exam 5: A Sulvey of Probability Concepts121 Questions
Exam 6: Discrete Probability Distributions145 Questions
Exam 7: Continuous Probabilitydistributions79 Questions
Exam 8: Sampling Methods and the Central Umit Theorem134 Questions
Exam 9: Estimation and Confidence Intervals140 Questions
Exam 10: One-Sample Tests of Hypothesis111 Questions
Exam 11: Two Sample Tests of Hypothesis103 Questions
Exam 12: Analysis of Variance173 Questions
Exam 13: Linear Regression and Correlation132 Questions
Exam 14: Multiple Regression and Correlation Analysis126 Questions
Exam 15: Chi-Square Applications94 Questions
Exam 16: Decision Making and Payoff Tables in Investment Scenarios151 Questions
Select questions type
Test at the 0.01 level the statement that 55% of those families who plan to purchase a vacation
Residence in Florida want a condominium. The null hypothesis is p = 0.55 and the alternate is p ≠
0)55. A random sample of 400 families who planned to buy a vacation residence revealed that 228
Families want a condominium. What decision should be made regarding the null hypothesis?
(Multiple Choice)
4.8/5  (40)
(40)
What is the probability of making a Type II error if the null hypothesis is actually true?
(Multiple Choice)
4.7/5  (29)
(29)
i. An alternate hypothesis is a statement about a population parameter that is accepted when the
Null hypothesis is rejected.
ii. The level of significance is the risk we assume of rejecting the null hypothesis when it is actually
True.
iii. There is only one level of significance that is applied to all studies involving sampling.
(Multiple Choice)
4.9/5  (36)
(36)
A nationwide survey of college students was conducted and found that students spend two hours
Per class hour studying. A professor at your school wants to determine whether the time students
Spend at your school is significantly different from the two hours. A random sample of fifteen
Statistics students is carried out and the findings indicate an average of 1.75 hours with a standard
Deviation of 0.24 hours.
Given the following printout, what can you determine? 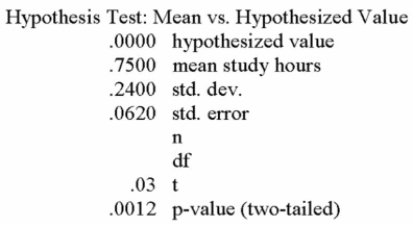
(Multiple Choice)
4.7/5  (41)
(41)
The average cost of tuition, room and board at community colleges is reported to be $8,500 per
Year with a standard deviation of $1,200, but a financial administrator believes that the average cost
Is higher. A study conducted using 150 community colleges showed that the average cost per year
Is $9,000. Let α = 0.05.
Given the z-statistic is 5.1, what is our decision about the average cost?
(Multiple Choice)
4.8/5  (34)
(34)
The printout below refers to the weekly closing stock prices for Air Canada on 20 randomly
Selected weeks in 2000. 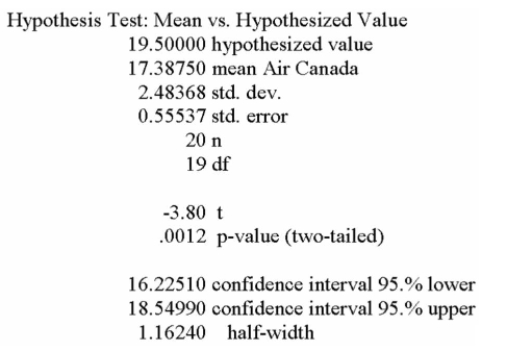 Using a 5% level of significance, can you say that the average Air Canada stock price was different
From $19.50?
Using a 5% level of significance, can you say that the average Air Canada stock price was different
From $19.50?
(Multiple Choice)
4.8/5  (37)
(37)
The printout below refers to the weekly closing stock prices for Air Canada on 20 randomly
Selected weeks in 2000.
Using a 5% level of significance, can you say that the average Air Canada stock price was less than
$19)00? 
(Multiple Choice)
4.9/5  (30)
(30)
A manufacturer claims that less than 1% of all his products do not meet the minimum government
Standards. A survey of 500 products revealed ten did not meet the standard.
(Multiple Choice)
4.7/5  (43)
(43)
It is claimed that in a bushel of peaches less than ten percent are defective. A sample of 400
Peaches is examined and 50 are found to be defective. What is the critical value for α = 0.025?
(Multiple Choice)
4.8/5  (39)
(39)
For a null hypothesis, H0: µ = 4,000, if the 1% level of significance is used and the z-test statistic is
+6)00, what is our decision regarding the null hypothesis?
(Multiple Choice)
4.9/5  (25)
(25)
It is claimed that in a bushel of peaches less than ten percent are defective. A sample of 400
Peaches is examined and 50 are found to be defective.
If α = 0.025, what will be the decision?
(Multiple Choice)
4.9/5  (35)
(35)
The Jamestown Steel Company manufactures and assembles desks and other office equipment at
Several plants. The weekly production of the Model A325 desk follows a normal probability
Distribution, with a mean of 200 and a standard deviation of 16. Recently, due to market expansion,
New production methods have been introduced and new employees hired. The vice president of
Manufacturing would like to investigate whether there has been a change in the weekly production
Of the Model A325 desk. The mean number of desks produced last year (50 weeks, because the
Plant was shut down two weeks for vacation) is 203.5. Is the mean number of desks changed from
200? Test using the .05 significance level. 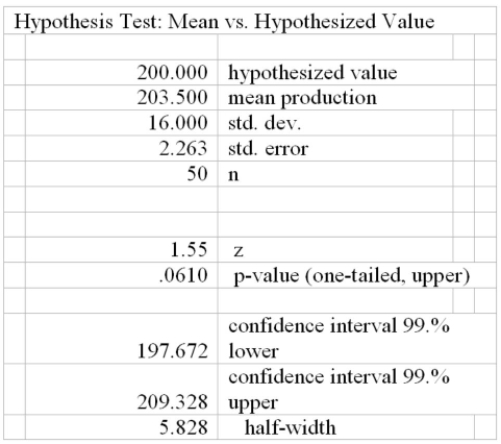
(Multiple Choice)
4.9/5  (43)
(43)
The mean weight of newborn infants at a community hospital is said to be 6.6 pounds. A sample of
Seven infants is randomly selected and their weights at birth are recorded as 9.0, 7.3, 6.0, 8.8, 6.8,
8)4, and 6.6 pounds. What is the sample standard deviation?
(Multiple Choice)
4.8/5  (40)
(40)
The mean gross annual incomes of certified tack welders are normally distributed with the mean of
$50,000 and a standard deviation of $4,000. The ship building association wishes to find out
Whether their tack welders earn more or less than $50,000 annually. The alternate hypothesis is
That the mean is not $50,000.
Which of the following is the alternate hypothesis?
(Multiple Choice)
4.8/5  (37)
(37)
A restaurant that bills its house account monthly is concerned that the average monthly bill exceeds
$200 per account. A random sample of twelve accounts is selected, resulting in a sample mean of
$220 and a standard deviation of $12. The t-test is to be conducted at the 5% level of significance.
The t-value is calculated to be 5.77. At the 0.01 level of significance, what is your decision?
(Multiple Choice)
4.9/5  (35)
(35)
The mean length of a small counter balance bar is 43 millimeters. There is concern that the
Adjustments of the machine producing the bars have changed. Test the claim at the 0.02 level that
There has been no change in the mean length. The alternate hypothesis is that there has been a
Change. Twelve bars (n = 12) were selected at random and their lengths recorded. The lengths are
(in millimeters) 42, 39, 42, 45, 43, 40, 39, 41, 40, 42, 43 and 42. The mean of the sample is 41.5 and
The standard deviation 1.784. Computed t = -2.913. Has there been a statistically significant change
In the mean length of the bars?
(Multiple Choice)
4.9/5  (35)
(35)
i. One assumption in testing a hypothesis about a proportion is that the data collected are the result
Of counting something.
ii. One assumption in testing a hypothesis about a proportion is that an outcome of an experiment
Can be classified into two mutually exclusive categories, namely, a success or a failure.
iii. A proportion is a fraction, ratio or probability that gives the part of the population or sample that
Has a particular trait of interest.
(Multiple Choice)
5.0/5  (40)
(40)
The Jamestown Steel Company manufactures and assembles desks and other office equipment at
Several plants. The weekly production of the Model A325 desk follows a normal probability
Distribution, with a mean of 200 and a standard deviation of 16. Recently, due to market expansion,
New production methods have been introduced and new employees hired. The vice president of
Manufacturing would like to investigate whether there has been a change in the weekly production
Of the Model A325 desk. The mean number of desks produced last year (50 weeks, because the
Plant was shut down two weeks for vacation) is 203.5. Is the mean number of desks produced
Different from 200? Test using the .01 significance level.
(Multiple Choice)
4.9/5  (38)
(38)
It is claimed that in a bushel of peaches less than ten percent are defective. A sample of 400
Peaches is examined and 50 are found to be defective. What is the sample proportion?
(Multiple Choice)
4.9/5  (43)
(43)
Showing 81 - 100 of 140
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)