Exam 16: Time-Series Forecasting

TABLE 16-14 A contractor developed a multiplicative time-series model to forecast the number of contracts in future quarters, using quarterly data on number of contracts during the 3-year period from 2008 to 2010. The following is the resulting regression equation: ln Ŷ = 3.37 + 0.117 X - 0.083 Q₁ + 1.28 Q₂ + 0.617 Q₃ where Ŷ is the estimated number of contracts in a quarter X is the coded quarterly value with X = 0 in the first quarter of 2008. Q₁ is a dummy variable equal to 1 in the first quarter of a year and 0 otherwise. Q₂ is a dummy variable equal to 1 in the second quarter of a year and 0 otherwise. Q₃ is a dummy variable equal to 1 in the third quarter of a year and 0 otherwise. -Referring to Table 16-14, in testing the coefficient for Q₁ in the regression equation (-0.083), the results were a t-statistic of -0.66 and an associated p-value of 0.530. Which of the following is the best interpretation of this result?

TABLE 16-3 The following table contains the number of complaints received in a department store for the first 6 months of last year. 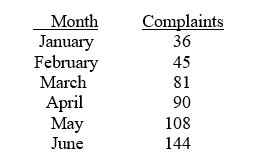-Referring to Table 16-3, if this series is smoothed using exponential smoothing with a smoothing constant of 1/3, what would be the second value?

TABLE 16-12 A local store developed a multiplicative time-series model to forecast its revenues in future quarters, using quarterly data on its revenues during the 4-year period from 2005 to 2009. The following is the resulting regression equation: log₁₀ = 6.102 + 0.012 X - 0.129 Q₁ - 0.054 Q₂ + 0.098 Q₃ where is the estimated number of contracts in a quarter. X is the coded quarterly value with X = 0 in the first quarter of 2005. Q₁ is a dummy variable equal to 1 in the first quarter of a year and 0 otherwise. Q₂ is a dummy variable equal to 1 in the second quarter of a year and 0 otherwise. Q₃ is a dummy variable equal to 1 in the third quarter of a year and 0 otherwise. -Referring to Table 16-12, the best interpretation of the constant 6.102 in the regression equation is

When a time series appears to be increasing at an increasing rate, such that percentage difference from value to value is constant, the appropriate model to fit is the

TABLE 16-8 The manager of a marketing consulting firm has been examining his company's yearly profits. He believes that these profits have been showing a quadratic trend since 1990. He uses Microsoft Excel to obtain the partial output below. The dependent variable is profit (in thousands of dollars), while the independent variables are coded years and squared of coded years, where 1990 is coded as 0, 1991 is coded as 1, etc. SUMMARY OUTPUT Regression Statistics Multiple R 0.998 R Square 0.996 Adjusted R Square 0.996 Standard Error 4.996 Observations 17 Coefficients Intercept 35.5 Coded Year 0.45 Year Squared 1.00 -Referring to Table 16-8, the forecast for profits in 2010 is ________.

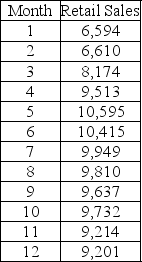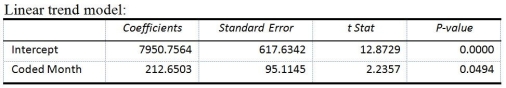
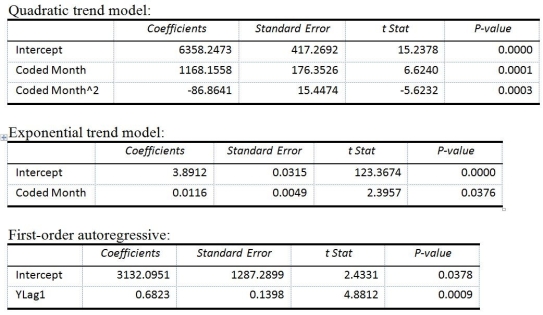
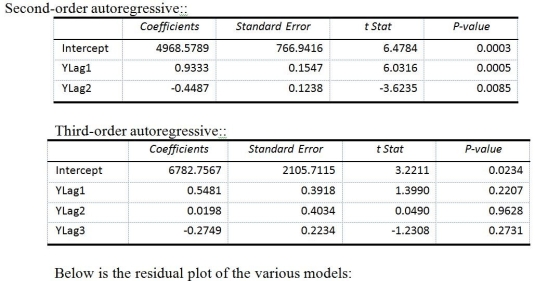
TABLE 16-13 Given below is the monthly time-series data for U.S. retail sales of building materials over a specific year. The results of the linear trend, quadratic trend, exponential trend, first-order autoregressive, second-order autoregressive and third-order autoregressive model are presented below in which the coded month for the first month is 0: -Referring to Table 16-13, the best model based on the residual plots is the quadratic-trend regression model.

TABLE 16-13 Given below is the monthly time-series data for U.S. retail sales of building materials over a specific year. The results of the linear trend, quadratic trend, exponential trend, first-order autoregressive, second-order autoregressive and third-order autoregressive model are presented below in which the coded month for the first month is 0: -Referring to Table 16-13, the best autoregressive model using the 5% level of significance is

TABLE 16-3 The following table contains the number of complaints received in a department store for the first 6 months of last year. -Referring to Table 16-3, suppose the last two smoothed values are 81 and 96 (Note: they are not). What would you forecast as the value of the time series for September?

TABLE 16-11 The manager of a health club has recorded mean attendance in newly introduced step classes over the last 15 months: 32.1, 39.5, 40.3, 46.0, 65.2, 73.1, 83.7, 106.8, 118.0, 133.1, 163.3, 182.8, 205.6, 249.1, and 263.5. She then used Microsoft Excel to obtain the following partial output for both a first- and second-order autoregressive model. 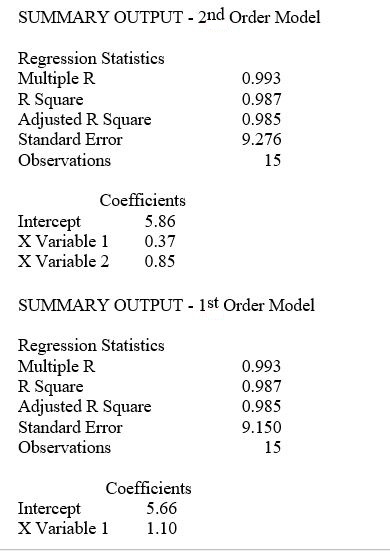-Referring to Table 16-11, based on the parsimony principle, the second-order model is the better model for making forecasts.

To assess the adequacy of a forecasting model, one measure that is often used is

TABLE 16-5 The number of passengers arriving at San Francisco on the Amtrak cross-country express on 6 successive Mondays were: 60, 72, 96, 84, 36, and 48. -Referring to Table 16-5, the number of arrivals will be smoothed with a 3-term moving average. The last smoothed value will be ________.

TABLE 16-4 The number of cases of merlot wine sold by a Paso Robles winery in an 8-year period follows. -Referring to Table 16-4, exponential smoothing with a weight or smoothing constant of 0.4 will be used to forecast wine sales. The forecast for 2011 is ________.

TABLE 16-13 Given below is the monthly time-series data for U.S. retail sales of building materials over a specific year. The results of the linear trend, quadratic trend, exponential trend, first-order autoregressive, second-order autoregressive and third-order autoregressive model are presented below in which the coded month for the first month is 0: -Referring to Table 16-13, if a five-month moving average is used to smooth this series, what would be the first calculated value?

TABLE 16-13 Given below is the monthly time-series data for U.S. retail sales of building materials over a specific year. The results of the linear trend, quadratic trend, exponential trend, first-order autoregressive, second-order autoregressive and third-order autoregressive model are presented below in which the coded month for the first month is 0: -Referring to Table 16-13, the best model based on the residual plots is the linear-trend model.

A second-order autoregressive model for average mortgage rate is: Rateᵢ = - 2.0 + 1.8(Rate)ᵢ₋₁ - 0.5 (Rate)ᵢ₋₂. If the average mortgage rate in 2010 was 7.0, and in 2009 was 6.4, the forecast for 2012 is ________.

TABLE 16-12 A local store developed a multiplicative time-series model to forecast its revenues in future quarters, using quarterly data on its revenues during the 4-year period from 2005 to 2009. The following is the resulting regression equation: log₁₀ = 6.102 + 0.012 X - 0.129 Q₁ - 0.054 Q₂ + 0.098 Q₃ where is the estimated number of contracts in a quarter. X is the coded quarterly value with X = 0 in the first quarter of 2005. Q₁ is a dummy variable equal to 1 in the first quarter of a year and 0 otherwise. Q₂ is a dummy variable equal to 1 in the second quarter of a year and 0 otherwise. Q₃ is a dummy variable equal to 1 in the third quarter of a year and 0 otherwise. -Referring to Table 16-12, using the regression equation, what is the forecast for the revenues in the first quarter of 2012?

TABLE 16-14 A contractor developed a multiplicative time-series model to forecast the number of contracts in future quarters, using quarterly data on number of contracts during the 3-year period from 2008 to 2010. The following is the resulting regression equation: ln Ŷ = 3.37 + 0.117 X - 0.083 Q₁ + 1.28 Q₂ + 0.617 Q₃ where Ŷ is the estimated number of contracts in a quarter X is the coded quarterly value with X = 0 in the first quarter of 2008. Q₁ is a dummy variable equal to 1 in the first quarter of a year and 0 otherwise. Q₂ is a dummy variable equal to 1 in the second quarter of a year and 0 otherwise. Q₃ is a dummy variable equal to 1 in the third quarter of a year and 0 otherwise. -Referring to Table 16-14, to obtain a forecast for the first quarter of 2011 using the model, which of the following sets of values should be used in the regression equation?

TABLE 16-13 Given below is the monthly time-series data for U.S. retail sales of building materials over a specific year. The results of the linear trend, quadratic trend, exponential trend, first-order autoregressive, second-order autoregressive and third-order autoregressive model are presented below in which the coded month for the first month is 0: -Referring to Table 16-13, what is your forecast for the 13ᵗʰ month using the quadratic-trend model?

TABLE 16-10 Business closures in Laramie, Wyoming from 2005 to 2010 were: -Referring to Table 16-10, the residuals for the first-order autoregressive model are ________, ________, ________, ________, and ________.

The annual multiplicative time-series model does not possess ________ component.