Exam 4: Systems of Linear Equations; Matrices
Exam 1: Linear Equations and Graphs59 Questions
Exam 2: Functions and Graphs178 Questions
Exam 3: Mathematics of Finance57 Questions
Exam 4: Systems of Linear Equations; Matrices115 Questions
Exam 5: Linear Inequalities and Linear Programming26 Questions
Exam 6: Linear Programming: The Simplex Method58 Questions
Exam 7: Logic, Sets, and Counting51 Questions
Exam 8: Probability59 Questions
Exam 9: Markov Chains60 Questions
Exam 10: Data Description and Probability Distributions65 Questions
Exam 11: Games and Decisions52 Questions
Exam 12: Appendix A: Basic Algebra Review45 Questions
Exam 13: Appendix B: Special Topics20 Questions
Select questions type
State whether the matrix is in reduced form or not in reduced form:
-
(Multiple Choice)
4.9/5  (37)
(37)
A retail company offers, through two different stores in a city, three models, A, B, and C, of a particular brand of camping stove. The inventory of each model on hand in each store is summarized in matrix M. Wholesale (W) and retail (R) prices of each model are summarized in matrix M. Find the product MN and label its columns and rows appropriately. What is the wholesale value of the inventory in Store 1?
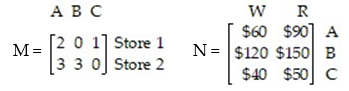
(Short Answer)
4.7/5  (35)
(35)
Labor and material costs for manufacturing each of three types of products M, N, and P are given in the table:ProductMNPLabor$50$40$50Materials$60$40$70The weekly allocation for labor is $50,000 and for materials is $80,000. There are to be 3 times as many units of product M manufactured as units of product P. How many of each type of product would be manufactured each week to use exactly each of the weekly allocations? Set up a system of linear equations, letting 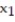 ,
, 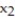 , and
, and 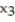 be the number of units of products M, N, and P, respectively, manufactured in one week.
be the number of units of products M, N, and P, respectively, manufactured in one week.
(Short Answer)
4.8/5  (40)
(40)
If $9,000 is to be invested, part at 13% and the rest at 8% simple interest, how much should be invested at each rate so that the total annual return will be the same as $9,000 invested at 9%? Set up a system of linear equations, letting 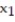 be the amount invested at 13% and
be the amount invested at 13% and 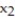 be the amount invested at 8%.
be the amount invested at 8%.
(Short Answer)
4.9/5  (32)
(32)
The system cannot be solved by matrix inverse methods. Find a method that could be used and then solve the system:
-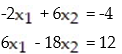
(Multiple Choice)
4.9/5  (31)
(31)
Solve the matrix equation 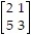
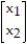 +.
+. 
 = by using the inverse of the coefficient matrix
= by using the inverse of the coefficient matrix
(Short Answer)
4.8/5  (33)
(33)
Find the matrix product mentally, without the use of a calculator or pencil-and-paper calculations:
-
(Multiple Choice)
4.8/5  (34)
(34)
Use a graphing utility and augmented matrix methods to solve the system: 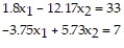 Express your answer accurate to three decimal places.
Express your answer accurate to three decimal places.
(Short Answer)
4.8/5  (36)
(36)
Write the system as a matrix equation of the form AX = B:
-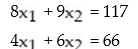
(Multiple Choice)
4.8/5  (32)
(32)
Showing 21 - 40 of 115
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)