Exam 4: Systems of Linear Equations; Matrices
Exam 1: Linear Equations and Graphs59 Questions
Exam 2: Functions and Graphs178 Questions
Exam 3: Mathematics of Finance57 Questions
Exam 4: Systems of Linear Equations; Matrices115 Questions
Exam 5: Linear Inequalities and Linear Programming26 Questions
Exam 6: Linear Programming: The Simplex Method58 Questions
Exam 7: Logic, Sets, and Counting51 Questions
Exam 8: Probability59 Questions
Exam 9: Markov Chains60 Questions
Exam 10: Data Description and Probability Distributions65 Questions
Exam 11: Games and Decisions52 Questions
Exam 12: Appendix A: Basic Algebra Review45 Questions
Exam 13: Appendix B: Special Topics20 Questions
Select questions type
Write the matrix equation as a system of linear equations without matrices:
-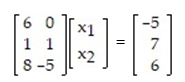
(Multiple Choice)
4.8/5  (27)
(27)
A message has been encoded and the matrix which the receiver gets is shown below. 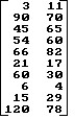 The encoding matrix A which was used to encode the message is:A =
The encoding matrix A which was used to encode the message is:A =  Find the decoding matrix A-1, and use it to decode the message.Assume that the numerical assignment used was a = 1, b = 2, ....., z = 26, space = 30, period = 40, and apostrophe = 60.
Find the decoding matrix A-1, and use it to decode the message.Assume that the numerical assignment used was a = 1, b = 2, ....., z = 26, space = 30, period = 40, and apostrophe = 60.
(Multiple Choice)
4.7/5  (30)
(30)
A company makes three chocolate candies: cherry, almond, and raisin. Matrix A gives the number of units of each ingredient in each type of candy in one batch. Matrix B gives the cost of each ingredient (dollars per unit) from suppliers X and Y. What is the cost of 100 batches from supplier X? 
(Multiple Choice)
4.9/5  (42)
(42)
A paper company produces high, medium, and low grade paper. The number of tons of each grade that is produced from one ton of pulp depends on the source of that pulp. The following table lists three sources and the amount of each grade of paper that can be made for one ton of pulp from each source.
 The paper company has orders for 11 tons of high grade, 15 tons of medium grade, and 14 tons of low grade paper. How many tons of each type of pulp should be used to fill these orders exactly? Set up a system of linear equations, letting x, y, and z be the number of tons of Brazilian pulp, domestic pulp, and recycled pulp, respectively, needed to fill the orders.
The paper company has orders for 11 tons of high grade, 15 tons of medium grade, and 14 tons of low grade paper. How many tons of each type of pulp should be used to fill these orders exactly? Set up a system of linear equations, letting x, y, and z be the number of tons of Brazilian pulp, domestic pulp, and recycled pulp, respectively, needed to fill the orders.
(Short Answer)
4.9/5  (27)
(27)
Find the values of a, b, c, and d that make the matrix equation true.
-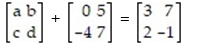
(Multiple Choice)
4.9/5  (34)
(34)
Solve the equation for the indicated variable. Assume that the dimensions are such that matrix multiplication and addition are possible and that inverses exist when needed:
-Solve for Y: XY + ZY = A
(Multiple Choice)
4.8/5  (33)
(33)
Showing 101 - 115 of 115
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)