Exam 12: Modeling Randomness

The volume in a can of soda is normally distributed with mean 358 mililiters (ml) and standard deviation 6 ml. -You buy a can of soda that has 364 ml of soda. How many standard deviations away from the mean volume is this can of soda?

(Multiple Choice)

4.9/5

(34)

Question 61

A lightbulb manufacturing plant's production line has determined that the probability a lightbulb will be defective is 0.04. The quality control team decides to take a random sample of 500 lightbulbs and measure the proportion of those lightbulbs that are defective. -On average, how far away should the quality control team expect the sample proportion of defective lightbulbs to be from 0.04?

(Short Answer)

4.9/5

(21)

Question 62

The volume in a can of soda is normally distributed with mean 358 mililiters (ml) and standard deviation 6 ml. -Use the Normal Probability Calculator applet to calculate the probability that a randomly chosen can of soda is filled with at least 360 ml of soda.

(Short Answer)

4.9/5

(35)

Question 63

Let X be the amount in claims (in dollars) that a randomly chosen policyholder collects from an insurance company this year. The probability distribution function of X is given below. -Calculate the expected value of X. $_________

(Short Answer)

4.9/5

(34)

Question 64

The volume in a can of soda is normally distributed with mean 358 mililiters (ml) and standard deviation 6 ml. -Let be the total volume in a six-pack of soda cans. Assume the six cans are filled independently. Then has a normal distribution with what mean and what standard deviation? Mean:(1) SD: (2)

(Short Answer)

4.9/5

(34)

Question 65

In a large population of college students, 56% live in a campus residence hall, 62% participate in a campus meal program, and 42% do both. Define events R = student lives in a campus residence hall M = student participates in a campus meal program -Find P(R^C).

(Short Answer)

4.8/5

(43)

Question 66

A soft drink company holds a contest in which a prize may be revealed on the inside of the bottle cap. The probability that each bottle cap reveals a prize is 0.39, and winning is independent from one bottle to the next. You buy six bottles. Let X be the number of prizes you win. -What is the expected value of the number of prizes in six bottles?

(Multiple Choice)

4.8/5

(39)

Question 67

In a large population of college students, 56% live in a campus residence hall, 62% participate in a campus meal program, and 42% do both. Define events R = student lives in a campus residence hall M = student participates in a campus meal program -How would you write the event that a college student "lives on campus and does not participate in a campus meal program" in probability notation?

(Multiple Choice)

4.8/5

(30)

Question 68

Suppose we randomly select a healthy adult from a large population and measure X = body temperature in degrees Fahrenheit. An appropriate probability model for the distribution of X is a

(Multiple Choice)

4.9/5

(34)

Question 69

Data on sex and opinion on the death penalty for respondents in the 2008 General Social Survey in which a random sample of 1,902 adults in the U.S. was surveyed are below. 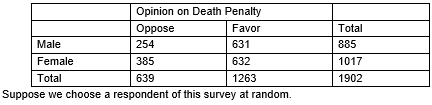-What is the probability that the respondent will oppose the death penalty, given that the respondent is male?

(Multiple Choice)

4.8/5

(28)

Question 70

A soft drink company holds a contest in which a prize may be revealed on the inside of the bottle cap. The probability that each bottle cap reveals a prize is 0.26, and winning is independent from one bottle to the next. You plan to keep buying bottles until you win a prize. Let X be the number of bottles you purchase. -Calculate P(X≥3).

(Short Answer)

4.8/5

(27)

Question 71

In a large population of college students, 56% live in a campus residence hall, 62% participate in a campus meal program, and 42% do both. Define events R = student lives in a campus residence hall M = student participates in a campus meal program -Suppose there are 100 students in this population. Fill in the Venn diagram below to represent the number of students in each category for this scenario. Hint: The four numbers should sum to 100!

(Short Answer)

4.8/5

(44)

Question 72

Let X be the amount in claims (in dollars) that a randomly chosen policyholder collects from an insurance company this year. The probability distribution function of X is given below. -What is the probability that a randomly chosen policy holder claims more than $100?

(Multiple Choice)

4.9/5

(33)

Question 73

A tire manufacturer designed a new tread pattern for its all-weather tires. Repeated tests were conducted on cars of approximately the same weight traveling at 60 miles per hour. The tests showed that the new tread pattern enables the cars to stop completely in an average distance of 125 feet with a standard deviation of 6.5 feet and that the stopping distances are approximately normally distributed. -Use the Normal Probability Calculator applet to find the 70^th percentile of the distribution of stopping distances?

(Short Answer)

4.7/5

(36)

Question 74

Consider a game in which a fair die is thrown. The player pays $5 to play and wins $2 for each dot that appears on the roll. Define X = number on which the die lands, and Y = player's net profit (amount won - amount paid to play). -Based on the expected value, is it worth the $5 to enter?

(Multiple Choice)

4.8/5

(38)

Question 75

A random sample of 25 college statistics textbook prices was collected and the mean price was found to be $91. To determine the probability of finding a sample mean of $91 or more extreme, you would need to refer to

(Multiple Choice)

4.9/5

(27)

Question 76

Suppose you roll a six-sided die once. Define events A = roll an even number B = roll a two C = roll an odd number D = roll a number greater than three -Find P(C∪D).

(Short Answer)

4.7/5

(28)

Question 77

An "instant lottery" is played by buying a ticket and scratching off a coating to reveal whether or not you have won a prize, and if so, how much. Suppose an instant lottery pays $5 with probability 0.05 and $100 with probability 0.006. Otherwise it pays nothing. Define X = amount won for a single ticket. (You can ignore the cost of the ticket.) -Calculate E(X). $_______

(Short Answer)

4.8/5

(38)

Question 78

Suppose you roll a six-sided die once. Define events A = roll an even number B = roll a two C = roll an odd number D = roll a number greater than three -Find P(A∪B).

(Short Answer)

4.9/5

(27)

Question 79

A lightbulb manufacturing plant's production line has determined that the probability a lightbulb will be defective is 0.04. The quality control team decides to take a random sample of 500 lightbulbs and measure the proportion of those lightbulbs that are defective. -Can we apply the normal approximation to the distribution of the sample proportion of defective lightbulbs?

(Multiple Choice)

4.9/5

(34)

Question 80

Showing 61 - 80 of 129

The volume in a can of soda is normally distributed with mean 358 mililiters (ml) and standard deviation 6 ml. -You buy a can of soda that has 364 ml of soda. How many standard deviations away from the mean volume is this can of soda?

The volume in a can of soda is normally distributed with mean 358 mililiters (ml) and standard deviation 6 ml. -Use the Normal Probability Calculator applet to calculate the probability that a randomly chosen can of soda is filled with at least 360 ml of soda.

Let X be the amount in claims (in dollars) that a randomly chosen policyholder collects from an insurance company this year. The probability distribution function of X is given below. -Calculate the expected value of X. $_________

In a large population of college students, 56% live in a campus residence hall, 62% participate in a campus meal program, and 42% do both. Define events R = student lives in a campus residence hall M = student participates in a campus meal program -Find P(R^C).

Suppose we randomly select a healthy adult from a large population and measure X = body temperature in degrees Fahrenheit. An appropriate probability model for the distribution of X is a

Data on sex and opinion on the death penalty for respondents in the 2008 General Social Survey in which a random sample of 1,902 adults in the U.S. was surveyed are below. -What is the probability that the respondent will oppose the death penalty, given that the respondent is male?

Let X be the amount in claims (in dollars) that a randomly chosen policyholder collects from an insurance company this year. The probability distribution function of X is given below. -What is the probability that a randomly chosen policy holder claims more than $100?

Consider a game in which a fair die is thrown. The player pays $5 to play and wins $2 for each dot that appears on the roll. Define X = number on which the die lands, and Y = player's net profit (amount won - amount paid to play). -Based on the expected value, is it worth the $5 to enter?

A random sample of 25 college statistics textbook prices was collected and the mean price was found to be $91. To determine the probability of finding a sample mean of $91 or more extreme, you would need to refer to

Suppose you roll a six-sided die once. Define events A = roll an even number B = roll a two C = roll an odd number D = roll a number greater than three -Find P(C∪D).

Suppose you roll a six-sided die once. Define events A = roll an even number B = roll a two C = roll an odd number D = roll a number greater than three -Find P(A∪B).

Preliminaries: Introduction to Statistical Investigations

Significance: How Strong Is the Evidence

Generalization: How Broadly Do the Results Apply

Estimation: How Large Is the Effect

Causation: Can We Say What Caused the Effect

Comparing Two Proportions

Comparing Two Means

Paired Data: One Quantitative Variable

Comparing More Than Two Proportions

Comparing More Than Two Means

Two Quantitative Variables

Filters

Exam 12: Modeling Randomness

The volume in a can of soda is normally distributed with mean 358 mililiters (ml) and standard deviation 6 ml. -You buy a can of soda that has 364 ml of soda. How many standard deviations away from the mean volume is this can of soda?

The volume in a can of soda is normally distributed with mean 358 mililiters (ml) and standard deviation 6 ml. -Use the Normal Probability Calculator applet to calculate the probability that a randomly chosen can of soda is filled with at least 360 ml of soda.

Let X be the amount in claims (in dollars) that a randomly chosen policyholder collects from an insurance company this year. The probability distribution function of X is given below. -Calculate the expected value of X. $_________

In a large population of college students, 56% live in a campus residence hall, 62% participate in a campus meal program, and 42% do both. Define events R = student lives in a campus residence hall M = student participates in a campus meal program -Find P(RC).

Suppose we randomly select a healthy adult from a large population and measure X = body temperature in degrees Fahrenheit. An appropriate probability model for the distribution of X is a

Data on sex and opinion on the death penalty for respondents in the 2008 General Social Survey in which a random sample of 1,902 adults in the U.S. was surveyed are below. -What is the probability that the respondent will oppose the death penalty, given that the respondent is male?

Let X be the amount in claims (in dollars) that a randomly chosen policyholder collects from an insurance company this year. The probability distribution function of X is given below. -What is the probability that a randomly chosen policy holder claims more than $100?

Consider a game in which a fair die is thrown. The player pays $5 to play and wins $2 for each dot that appears on the roll. Define X = number on which the die lands, and Y = player's net profit (amount won - amount paid to play). -Based on the expected value, is it worth the $5 to enter?

A random sample of 25 college statistics textbook prices was collected and the mean price was found to be $91. To determine the probability of finding a sample mean of $91 or more extreme, you would need to refer to

Suppose you roll a six-sided die once. Define events A = roll an even number B = roll a two C = roll an odd number D = roll a number greater than three -Find P(C∪D).

Suppose you roll a six-sided die once. Define events A = roll an even number B = roll a two C = roll an odd number D = roll a number greater than three -Find P(A∪B).

Preliminaries: Introduction to Statistical Investigations

Significance: How Strong Is the Evidence

Generalization: How Broadly Do the Results Apply

Estimation: How Large Is the Effect

Causation: Can We Say What Caused the Effect

Comparing Two Proportions

Comparing Two Means

Paired Data: One Quantitative Variable

Comparing More Than Two Proportions

Comparing More Than Two Means

Two Quantitative Variables

Filters

In a large population of college students, 56% live in a campus residence hall, 62% participate in a campus meal program, and 42% do both. Define events R = student lives in a campus residence hall M = student participates in a campus meal program -Find P(R^C).