Exam 4: Exponential Functions
Exam 1: Linear Functions and Change148 Questions
Exam 2: Functions138 Questions
Exam 3: Quadratic Functions46 Questions
Exam 4: Exponential Functions94 Questions
Exam 5: Logarithmic Functions87 Questions
Exam 6: Transformations of Functions and Their Graphs85 Questions
Exam 7: Trigonometry and Periodic Functions178 Questions
Exam 8: Triangle Trigonometry and Polar Coordinates43 Questions
Exam 9: Trigonometric Identities, Models, and Complex Numbers106 Questions
Exam 10: Compositions, Inverses, and Combinations of Functions69 Questions
Exam 11: Polynomial and Rational Functions145 Questions
Exam 12: Vectors and Matrices104 Questions
Exam 13: Sequences and Series81 Questions
Exam 14: Parametric Equations and Conic Sections128 Questions
Select questions type
Is the formula for a function representing a quantity which begins at in year and grows at a continuous annual rate of given by
Free
(True/False)
4.9/5  (32)
(32)
Correct Answer:
True
The average life expectancy in a country tends to increase by the same percentage each year. Should a linear or an exponential function be used to model this scenario?
Free
(Short Answer)
4.8/5  (33)
(33)
Correct Answer:
exponential
The price of an item increases due to inflation. Let give the price of the item as a function of time in years, with in 2004. At what continuous annual rate is the price increasing? Round to 2 decimal places.
Free
(Short Answer)
4.9/5  (44)
(44)
Correct Answer:
Write the formula for the price of a gallon of gas in days if the price is on day and the price increases by per day.
(Short Answer)
4.9/5  (30)
(30)
The US population in 2005 was approximately 296.4 million. Assume the population increases at a rate of per year. How many million people would you expect to live in the United States in the year 2020? Round to 1 decimal place.
(Short Answer)
4.8/5  (31)
(31)
Let be the intersection of the graphs of the two exponential functions and , where . If is increased, does increase, decrease, or stay the same?
(Short Answer)
4.8/5  (31)
(31)
Each of the functions in the table below is increasing, but each increases in a different way. One is linear, one is exponential, and one is neither.
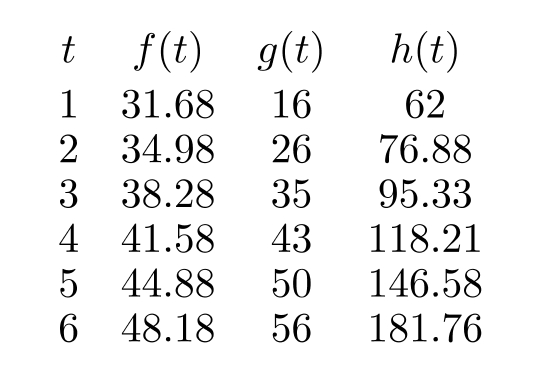 Which one is exponential: , or ?
Which one is exponential: , or ?
(Short Answer)
4.8/5  (31)
(31)
Which of the following quantities are increasing with time ?
(Multiple Choice)
4.8/5  (40)
(40)
A population is 150,000 in year and declines at a continuous rate of per year. By what percentage does the population decrease each year? Round to 2 decimal places.
(Short Answer)
4.8/5  (45)
(45)
Consider the following figure, where Graph I has equation , Graph II has equation , Graph III has equation , and Graph IV has equation .
 Is positive or negative?
Is positive or negative?
(Short Answer)
4.8/5  (30)
(30)
Without a calculator, graph the following on the same set of axes:
A)
B)
C)
(Short Answer)
4.9/5  (35)
(35)
The graph of the exponential function is shown below. The formula for
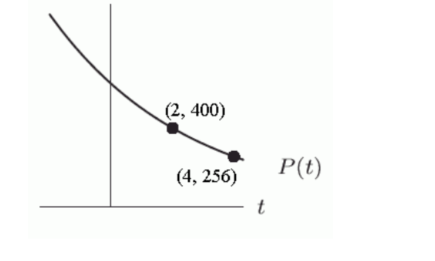
(Short Answer)
4.9/5  (36)
(36)
The populations of 4 species of animals are given by the following equations:
Which species are shrinking in size?
(Multiple Choice)
4.9/5  (43)
(43)
The populations of 4 species of animals are given by the following equations:
What is the annual percent growth rate for the population that is growing the fastest?
(Short Answer)
4.8/5  (39)
(39)
One solution to the equation is . Use your calculator to estimate the other solution to 2 decimal places.
(Short Answer)
4.9/5  (40)
(40)
Showing 1 - 20 of 94
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)