Exam 11: Nonlinear Functions, Conic Sections, and Nonlinear Systems
Exam 1: Review of the Real Number System431 Questions
Exam 2: Linear Equations, Inequalities, and Applications338 Questions
Exam 3: Linear Equations, Graphs, and Functions347 Questions
Exam 4: Systems of Linear Equations166 Questions
Exam 5: Exponents, Polynomials, and Polynomial Functions327 Questions
Exam 6: Factoring180 Questions
Exam 7: Rational Expressions and Functions233 Questions
Exam 8: Roots, Radicals, and Root Functions358 Questions
Exam 9: Quadratic Equations, Inequalities, and Functions339 Questions
Exam 10: Inverse, Exponential, and Logarithmic Functions292 Questions
Exam 11: Nonlinear Functions, Conic Sections, and Nonlinear Systems233 Questions
Exam 12: Further Topics in Algebra190 Questions
Exam 13: Appendices29 Questions
Select questions type
Solve the system by the elimination method or a combination of the elimination and substitution methods.
-
(Multiple Choice)
4.8/5  (33)
(33)
Which method should be used to solve the system? Explain your answer, including a description of the first step.
- -2- =9 3+4 =4
(Essay)
4.8/5  (42)
(42)
The circle or ellipse shown in the calculator-generated graph was created using function mode with a square viewing window. What are the two functions and that were used to obtain the graph whose equation is given?
-
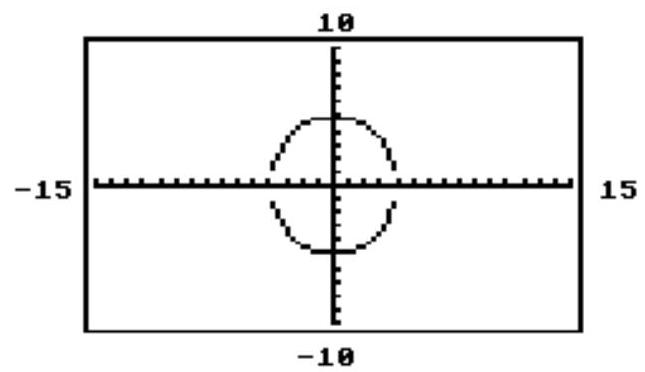
(Multiple Choice)
4.8/5  (33)
(33)
Solve the problem.
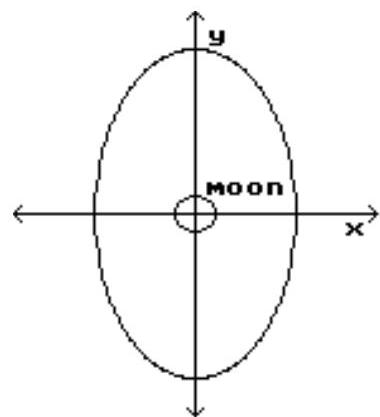
-A satellite is to be put into an elliptical orbit around a moon. The moon is a sphere with radius 589 . Determine an equation for the ellipse, where and are measured in , if the distance of the satellite from the surface of the moon varies from to .
(Multiple Choice)
4.8/5  (35)
(35)
Find the equation of a circle satisfying the given conditions.
-Center: ; radius:
(Multiple Choice)
4.9/5  (35)
(35)
The hyperbola shown in the calculator-generated graph was graphed in function mode with a square viewing window. What are the two functions and that were used to obtain the graph whose equation is given?
-
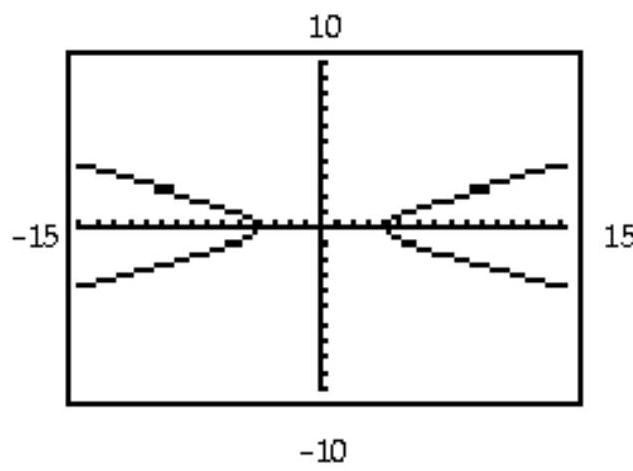
(Multiple Choice)
4.9/5  (35)
(35)
Graph the step function.
-Assume it costs 25 cents to mail a letter weighing one ounce or less, and then 20 cents for each additional ounce or fraction of an ounce. Let be the cost of mailing a letter weighing ounces. Graph . Use the interval .
(Multiple Choice)
4.9/5  (38)
(38)
The circle or ellipse shown in the calculator-generated graph was created using function mode with a square viewing window. What are the two functions and that were used to obtain the graph whose equation is given?
-
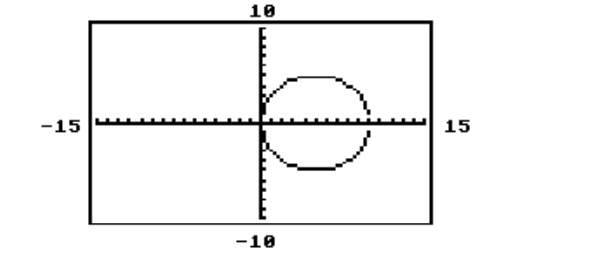
(Multiple Choice)
4.8/5  (37)
(37)
Graph the system of inequalities.
- x\leq0 y\leq0 +\geq9 y+x\geq-5
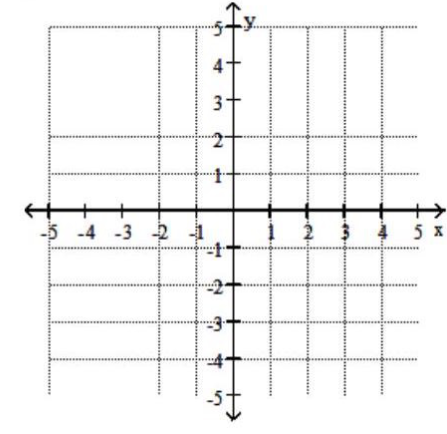
(Multiple Choice)
4.8/5  (33)
(33)
The hyperbola shown in the calculator-generated graph was graphed in function mode with a square viewing window. What are the two functions and that were used to obtain the graph whose equation is given?
-
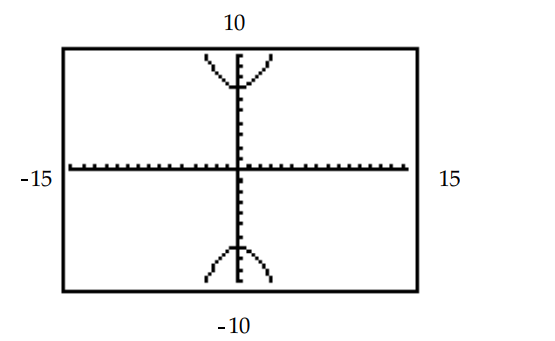
(Multiple Choice)
4.8/5  (33)
(33)
Solve the problem. Round your answer to the nearest tenth.
-The roof of a building is in the shape of the top half of the hyperbola , where and are in meters. Refer to the figure and determine the distance, , between the two outside walls.
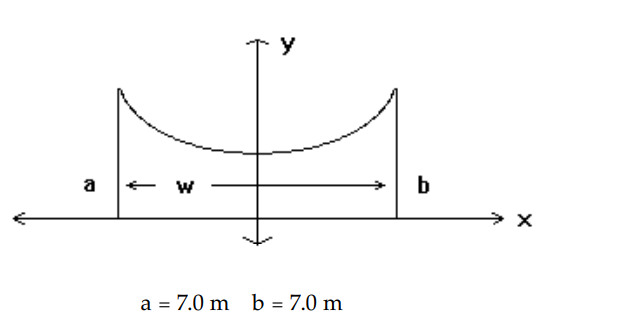
(Multiple Choice)
4.9/5  (37)
(37)
Showing 21 - 40 of 233
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)