Question 1

What assumptions are necessary for a Markov process to have stationary transition probabilities?

Accepted Answer

For a Markov process to have stationary

Question 2

All entries in a matrix of transition probabilities sum to 1.

Accepted Answer

A) True 
 B)False

Question 3

A state i is a transient state if there exists a state j that is reachable from i,but the state i is not reachable from state j.

Accepted Answer

A) True 
 B)False

Question 4

Bark Bits Company is planning an advertising campaign to raise the brand loyalty of its customers to .80.
a.The former transition matrix is $$\left[ \begin{array} { c c } 
.75 & .25 \
.20 & .80
\end{array} \right]$$ What is the new one? 
b.What are the new steady state probabilities?
c.If each point of market share increases profit by $15000, what is the most you would pay for the advertising?

Accepted Answer

a. @#IMG-DLM& b.F1F1F1S1F1F1F10₁ = .5,F1

Question 5

All Markov chains have steady-state probabilities.

Accepted Answer

A) True 
 B)False

Question 6

Analysis of a Markov process

Accepted Answer

A) describes future behavior of the system. 
B) optimizes the system. 
C) leads to higher order decision making. 
D) All of the alternatives are true. 
A) describes future behavior of the system. 
B) optimizes the system. 
C) leads to higher order decision making. 
D) All of the alternatives are true.

Question 7

When absorbing states are present,each row of the transition matrix corresponding to an absorbing state will have a single 1 and all other probabilities will be 0.

Accepted Answer

A) True 
 B)False

Question 8

Henry,a persistent salesman,calls North's Hardware Store once a week hoping to speak with the store's buying agent,Shirley.If Shirley does not accept Henry's call this week,the probability she will do the same next week is .35.On the other hand,if she accepts Henry's call this week,the probability she will not do so next week is .20.
a.Construct the transition matrix for this problem.
b.How many times per year can Henry expect to talk to Shirley?
c.What is the probability Shirley will accept Henry's next two calls if she does not accept his call this week?
d.What is the probability of Shirley accepting exactly one of Henry's next two calls if she accepts his call this week?

Accepted Answer

a.The transition mat

Question 9

On any particular day an individual can take one of two routes to work.Route A has a 25% chance of being congested,whereas route B has a 40% chance of being congested.
The probability of the individual taking a particular route depends on his previous day's experience.If one day he takes route A and it is not congested,he will take route A again the next day with probability .8.If it is congested,he will take route B the next day with probability .7.
On the other hand,if on a day he takes route B and it is not congested,he will take route B again the next day with probability .9.Similarly if route B is congested,he will take route A the next day with probability .6.
a.Construct the transition matrix for this problem. (HINT: There are 4 states corresponding to the route taken and the congestion. The transition probabilities are products of the independent probabilities of congestion and next day choice.)
b.What is the long-run proportion of time that route A is taken?

Accepted Answer

a. @#IMG-DLM& b..36

Question 10

The probability that a system is in a particular state after a large number of periods is

Accepted Answer

A) independent of the beginning state of the system. 
B) dependent on the beginning state of the system. 
C) equal to one half. 
D) the same for every ending system. 
A) independent of the beginning state of the system. 
B) dependent on the beginning state of the system. 
C) equal to one half. 
D) the same for every ending system.

Question 11

Calculate the steady state probabilities for this transition matrix. $$\left[ \begin{array} { c c } 
.60 & .40 \
.30 & .70
\end{array} \right]$$

Accepted Answer

F1F1F1S1F

Question 12

For a situation with weekly dining at either an Italian or Mexican restaurant,

Accepted Answer

A) the weekly visit is the trial and the restaurant is the state. 
B) the weekly visit is the state and the restaurant is the trial. 
C) the weekly visit is the trend and the restaurant is the transition. 
D) the weekly visit is the transition and the restaurant is the trend. 
A) the weekly visit is the trial and the restaurant is the state. 
B) the weekly visit is the state and the restaurant is the trial. 
C) the weekly visit is the trend and the restaurant is the transition. 
D) the weekly visit is the transition and the restaurant is the trend.

Question 13

The vice president of personnel at Jetair Aerospace has noticed that yearly shifts in personnel can be modeled by a Markov process.The transition matrix is: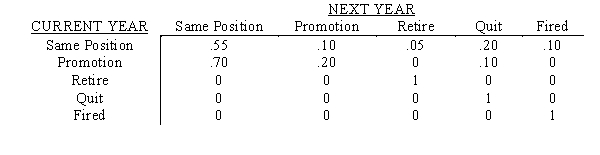   
a. Write the transition matrix in the form of I, O, R, and Q submatrices.
b. Compute the fundamental matrix, N, for this problem.
c. What is the probability of an employee who was just promoted eventually retiring? Quitting? Being fired?

Accepted Answer

a.Because the identity matrix must be in

Question 14

If an absorbing state exists,then the probability that a unit will ultimately move into the absorbing state is given by the steady state probability.

Accepted Answer

A) True 
 B)False

Question 15

The probability of going from state 1 in period 2 to state 4 in period 3 is

Accepted Answer

A) p12 
B) p23 
C) p14 
D) p43 
A) p12 
B) p23 
C) p14 
D) p43

Question 16

The evening television news broadcast that individuals view on one evening is influenced by which broadcast they viewed previously.An executive at the C network has determined the following transition probability matrix describing this phenomenon.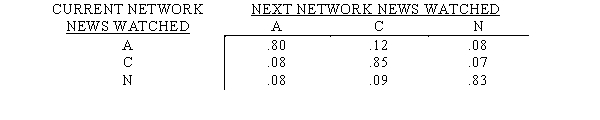   
a. Which network has the most loyal viewers?
b. What are the three networks' long-run market shares?
c. Suppose each of the three networks earns $1,250 in daily profit from advertising revenue for each 1,000,000 viewers it has. If on the average 40,000,000 people watch the evening television news, compute the long run average daily profit each network generates from its evening news broadcast.

Accepted Answer

a.Network C b.(p_A,p_C,p

Question 17

The medical prognosis for a patient with a certain disease is to recover,to die,to exhibit symptom 1,or to exhibit symptom 2.The matrix of transition probabilities is $$\begin{array} { c | c c c c } 
& \text { Recover } & \text { Die } & \mathrm { S } _ { 1 } & \mathrm {~S} _ { 2 } \
\hline \text { Recover } & 1 & 0 & 0 & 0 \
\text { Die } & 0 & 1 & 0 & 0 \
\mathrm {~S} _ { 1 } & 1 / 4 & 1 / 4 & 1 / 3 & 1 / 6 \
\mathrm {~S} _ { 2 } & 1 / 4 & 1 / 8 & 1 / 8 & 1 / 2
\end{array}$$ 
a.What are the absorbing states?
b.What is the probability that a patient with symptom 2 will recover?

Accepted Answer

a.States 1 and 2 (recover and

Question 18

Where is a fundamental matrix,N,used? How is N computed?

Accepted Answer

A fundamental matrix, denoted as N, is u

Question 19

Why is a computer necessary for some Markov analyses?

Accepted Answer

A computer is necessary for some Markov

Question 20

A state,i,is an absorbing state if,when i = j,pij = 1.

Accepted Answer

A) True 
 B)False

What assumptions are necessary for a Markov process to have stationary transition probabilities?

All entries in a matrix of transition probabilities sum to 1.

A state i is a transient state if there exists a state j that is reachable from i,but the state i is not reachable from state j.

All Markov chains have steady-state probabilities.

Analysis of a Markov process

When absorbing states are present,each row of the transition matrix corresponding to an absorbing state will have a single 1 and all other probabilities will be 0.

The probability that a system is in a particular state after a large number of periods is

Calculate the steady state probabilities for this transition matrix. $\left[ \begin{array} { c c } .60 & .40 \\.30 & .70\end{array} \right]$

For a situation with weekly dining at either an Italian or Mexican restaurant,

If an absorbing state exists,then the probability that a unit will ultimately move into the absorbing state is given by the steady state probability.

The probability of going from state 1 in period 2 to state 4 in period 3 is

Where is a fundamental matrix,N,used? How is N computed?

Why is a computer necessary for some Markov analyses?

A state,i,is an absorbing state if,when i = j,p_ij = 1.

Introduction

Introduction to Probability

Probability Distributions

Decision Analysis

Utility and Game Theory

Times Series Analysis and Forecasting

Introduction to Linear Programming

Linear Programming: Sensitivity Analysis and Interpretation of Solution

Linear Programming Applications in Marketing, Finance, and Operations Management

Distribution and Network Models

Integer Linear Programming

Advanced Optimization Applications

Project Scheduling: Pertcpm

Inventory Models

Waiting Line Models

Simulation

Filters

Exam 17: Markov Processes

What assumptions are necessary for a Markov process to have stationary transition probabilities?

All entries in a matrix of transition probabilities sum to 1.

A state i is a transient state if there exists a state j that is reachable from i,but the state i is not reachable from state j.

All Markov chains have steady-state probabilities.

Analysis of a Markov process

When absorbing states are present,each row of the transition matrix corresponding to an absorbing state will have a single 1 and all other probabilities will be 0.

The probability that a system is in a particular state after a large number of periods is

Calculate the steady state probabilities for this transition matrix. [.60.40.30.70]\left[ \begin{array} { c c } .60 & .40 \\.30 & .70\end{array} \right][.60.30​.40.70​]

For a situation with weekly dining at either an Italian or Mexican restaurant,

If an absorbing state exists,then the probability that a unit will ultimately move into the absorbing state is given by the steady state probability.

The probability of going from state 1 in period 2 to state 4 in period 3 is

Where is a fundamental matrix,N,used? How is N computed?

Why is a computer necessary for some Markov analyses?

A state,i,is an absorbing state if,when i = j,pij = 1.

Introduction

Introduction to Probability

Probability Distributions

Decision Analysis

Utility and Game Theory

Times Series Analysis and Forecasting

Introduction to Linear Programming

Linear Programming: Sensitivity Analysis and Interpretation of Solution

Linear Programming Applications in Marketing, Finance, and Operations Management

Distribution and Network Models

Integer Linear Programming

Advanced Optimization Applications

Project Scheduling: Pertcpm

Inventory Models

Waiting Line Models

Simulation

Filters

Calculate the steady state probabilities for this transition matrix. $\left[ \begin{array} { c c } .60 & .40 \\.30 & .70\end{array} \right]$

A state,i,is an absorbing state if,when i = j,p_ij = 1.