Exam 8: Estimating Single Population Parameters
Exam 1: The Where, why, and How of Data Collection167 Questions
Exam 2: Graphs,charts and Tablesdescribing Your Data138 Questions
Exam 3: Describing Data Using Numerical Measures130 Questions
Exam 4: Using Probability and Probability Distributions77 Questions
Exam 5: Discrete Probability Distributions119 Questions
Exam 6: Introduction to Continuous Probability Distributions90 Questions
Exam 7: Introduction to Sampling Distributions104 Questions
Exam 8: Estimating Single Population Parameters145 Questions
Exam 9: Introduction to Hypothesis Testing129 Questions
Exam 10: Estimation and Hypothesis Testing for Two Population Parameters97 Questions
Exam 11: Hypothesis Tests and Estimation for Population Variances71 Questions
Exam 12: Analysis of Variance137 Questions
Exam 13: Goodness-Of-Fit Tests and Contingency Analysis104 Questions
Exam 14: Introduction to Linear Regression and Correlation Analysis136 Questions
Exam 15: Multiple Regression Analysis and Model Building153 Questions
Exam 16: Analyzing and Forecasting Time-Series Data133 Questions
Exam 17: Introduction to Nonparametric Statistics104 Questions
Exam 18: Introduction to Quality and Statistical Process Control110 Questions
Exam 19: Introduction to Decision Analysis116 Questions
Select questions type
The following data represent a random sample of bank balances for a population of checking account customers at a large eastern bank.Based on these data,what is the critical value for a 95 percent confidence interval estimate for the true population mean? 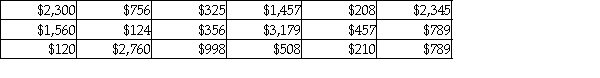
(Multiple Choice)
4.8/5  (39)
(39)
In an effort to estimate the mean length of stay for motel guests at a major national motel chain,the decision makers asked for a 95 percent confidence,and a margin of error of ± 0.25 days.Further,it was known that the population standard deviation is 0.50 days.Given this,the required sample size to estimate the mean length of stay is about 16 customers.
(True/False)
4.7/5  (39)
(39)
In determining the sample size requirement for an application involving the estimation of the proportion of department store customers who pay using the store's credit card,the closer the true proportion is to .5,the larger will be the required sample size for a given margin of error and confidence level.
(True/False)
4.8/5  (34)
(34)
An educational organization in California is interested in estimating the mean number of minutes per day that children between the age of 6 and 18 spend watching television per day.A previous study showed that the population standard deviation was 21.5 minutes.The organization selected a random sample of n = 200 children between the age of 6 and 18 and recorded the number of minutes of TV that each person watched on a particular day.The mean time was 191.3 minutes.If the leaders of the organization wish to develop an interval estimate with 95 percent confidence,what will the margin of error be?
(Multiple Choice)
4.7/5  (39)
(39)
The produce manager for a large retail food chain is interested in estimating the percentage of potatoes that arrive on a shipment with bruises.A random sample of 150 potatoes showed 14 with bruises.Based on this information,what is the margin of error for a 95 percent confidence interval estimate?
(Multiple Choice)
4.7/5  (21)
(21)
A point estimate is equally likely to be higher or lower than the population mean if the sampling is done using a statistical sampling procedure.
(True/False)
4.7/5  (38)
(38)
Which of the following statements is true with respect to the confidence level associated with an estimation application?
(Multiple Choice)
4.8/5  (35)
(35)
A point estimate for the population mean will always fall within the confidence interval estimate.
(True/False)
4.8/5  (32)
(32)
The fact that a point estimate will likely be different than the corresponding population value is due to the fact that point estimates are subject to sampling error.
(True/False)
4.7/5  (39)
(39)
In developing a confidence interval estimate for the population mean,the t-distribution is used to obtain the critical value when:
(Multiple Choice)
4.8/5  (32)
(32)
A popular restaurant takes a random sample n = 25 customers and records how long each occupied a table.The found a sample mean of 1.2 hours and a sample standard deviation of 0.3 hours.Find the 95 percent confidence interval for the mean.
(Multiple Choice)
4.9/5  (32)
(32)
One of the major oil products companies conducted a study recently to estimate the mean gallons of gasoline purchased by customers per visit to a gasoline station.To do this,a random sample of customers was selected with the following data being recorded that show the gallons of gasoline purchased.  Based on these sample data,construct and interpret a 95 percent confidence interval estimate for the population mean.
Based on these sample data,construct and interpret a 95 percent confidence interval estimate for the population mean.
(Essay)
4.9/5  (32)
(32)
When determining sample size for a proportion,using π = 0.5 will produce the smallest possible value for n.
(True/False)
4.8/5  (31)
(31)
In a sample size determination situation,reducing the margin of error by half will double the required sample size.
(True/False)
4.9/5  (40)
(40)
Recently,a report in a financial journal indicated that the 90 percent confidence interval estimate for the proportion of investors who own one or mutual funds is between 0.88 and 0.92.Given this information,the sample size that was used in this study was approximately 609 investors.
(True/False)
4.8/5  (35)
(35)
A university computer lab manager wishes to estimate the mean time that students stay in the lab per visit.She believes that the population standard deviation would be no larger than 10 minutes.Further,she wishes to have a confidence level of 90 percent and a margin of error of ± 2.00 minutes.Given this,the sample size that she uses is approximately 60 students.
(True/False)
4.9/5  (32)
(32)
The t-distribution is used to obtain the critical value in developing a confidence interval when the population distribution is not known and the sample size is small.
(True/False)
4.8/5  (38)
(38)
Confidence intervals constructed with small samples tend to have greater margins of error than those constructed from larger samples,all else being constant.
(True/False)
4.8/5  (36)
(36)
A sample of 250 people resulted in a confidence interval estimate for the proportion of people who believe that the federal government's proposed tax increase is justified is between 0.14 and 0.20.Based on this information,what was the confidence level used in this estimation?
(Multiple Choice)
4.9/5  (32)
(32)
Showing 21 - 40 of 145
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)