Exam 12: Analysis of Variance
Exam 1: The Where, why, and How of Data Collection167 Questions
Exam 2: Graphs,charts and Tablesdescribing Your Data138 Questions
Exam 3: Describing Data Using Numerical Measures130 Questions
Exam 4: Using Probability and Probability Distributions77 Questions
Exam 5: Discrete Probability Distributions119 Questions
Exam 6: Introduction to Continuous Probability Distributions90 Questions
Exam 7: Introduction to Sampling Distributions104 Questions
Exam 8: Estimating Single Population Parameters145 Questions
Exam 9: Introduction to Hypothesis Testing129 Questions
Exam 10: Estimation and Hypothesis Testing for Two Population Parameters97 Questions
Exam 11: Hypothesis Tests and Estimation for Population Variances71 Questions
Exam 12: Analysis of Variance137 Questions
Exam 13: Goodness-Of-Fit Tests and Contingency Analysis104 Questions
Exam 14: Introduction to Linear Regression and Correlation Analysis136 Questions
Exam 15: Multiple Regression Analysis and Model Building153 Questions
Exam 16: Analyzing and Forecasting Time-Series Data133 Questions
Exam 17: Introduction to Nonparametric Statistics104 Questions
Exam 18: Introduction to Quality and Statistical Process Control110 Questions
Exam 19: Introduction to Decision Analysis116 Questions
Select questions type
The Tukey-Kramer method for multiple comparison is used after the analysis of variance F-test has lead us to reject the null hypothesis that all population means are equal.
Free
(True/False)
4.8/5  (23)
(23)
Correct Answer:
True
In a two-factor ANOVA,the minimum number of replications required in any cell is two,but all cells must have the same number of replications.
Free
(True/False)
4.7/5  (35)
(35)
Correct Answer:
True
A large orchard owner in the state of Washington is interested in determining whether the mean number of bushels of peaches per acre is the same or different depending on the type of tree that is used.He also thinks that production may be affected by the type of fertilizer that is used.To test,he has set up a test in which a one-acre plot of peach trees with a combination each of 5 varieties and 3 fertilizer types are studied.The following data reflect the number of bushels of peaches on each acre plot. 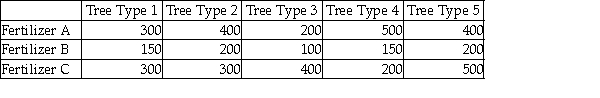 Assuming that the hypothesis tests will be conducted using an alpha equal 0.05 level,what is the value of the Fisher's LSD critical value for doing the multiple comparisons?
Assuming that the hypothesis tests will be conducted using an alpha equal 0.05 level,what is the value of the Fisher's LSD critical value for doing the multiple comparisons?
Free
(Multiple Choice)
4.8/5  (31)
(31)
Correct Answer:
C
Three brands of running shoes are each tested by 10 different runners.The amount of wear on the sole of the shoes is then measured.The objective is to determine if there is any difference among the three brands of shoes based on how long the soles last.The degrees of freedom for testing whether the brands of shoes differ are D1 = 9 and D2 = 2.
(True/False)
4.8/5  (42)
(42)
A study was recently conducted to see whether the mean starting salaries for graduates of engineering,business,healthcare,and computer information systems majors differ.A random sample of 8 graduates was selected from each major.The following shows the results of the ANOVA computations.However,the degrees of freedom column has been omitted.The correct number of degrees of freedom for the within variation is 28.
Anova: Single Factor
SUMMARY  ANOVA
ANOVA 
(True/False)
4.7/5  (37)
(37)
In order to analyze any potential interactions between factors in an analysis of variance study,it is necessary to have at least two measurements at each level of each factor.
(True/False)
4.8/5  (40)
(40)
Under the basic logic of one-way analysis of variance,if the within variation is large relative to the between variation,it is an indication that the population means are likely to be different.
(True/False)
4.9/5  (29)
(29)
The State Transportation Department is thinking of changing its speed limit signs.It is considering two new options in addition to the existing sign design.At question is whether the three sign designs will produce the same mean speed.To test this,the department has conducted a limited test in which a stretch of roadway was selected.With the original signs up,a random sample of 30 cars was selected and the speeds were measured.Then,on different days,the two new designs were installed,30 cars each day were sampled,and their speeds were recorded.Suppose that the following summary statistics were computed based on the data: 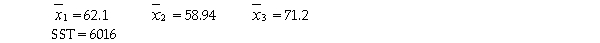 Based on these sample results and a significance level equal to 0.05,assuming that the null hypothesis of equal means has been rejected,the Tukey-Kramer critical range is:
Based on these sample results and a significance level equal to 0.05,assuming that the null hypothesis of equal means has been rejected,the Tukey-Kramer critical range is:
(Multiple Choice)
4.7/5  (31)
(31)
A national car rental company recently conducted a study recently in which cars with automatic and standard transmissions (factor A Sample)were rented to male and female customers (factor B Columns).Three customers in each category were randomly selected and the miles driven per day was recorded as follows:  Based on the design of this study,how many degrees of freedom will be associated with the mean square for factor A?
Based on the design of this study,how many degrees of freedom will be associated with the mean square for factor A?
(Multiple Choice)
4.9/5  (39)
(39)
In a completely randomized analysis of variance design,the observations from each factor are selected in an independent and random fashion.
(True/False)
4.8/5  (37)
(37)
A national car rental agency is interested in determining whether the mean days that customers rent cars is the same between three of its major cities.The following data reflect the number of days people rented a car for a sample of people in each of three cities.Given this information,the correct null and alternative hypotheses are:  :
:  =
= 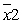 =
= 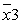
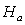 : not all
: not all 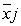 are equal.
are equal. 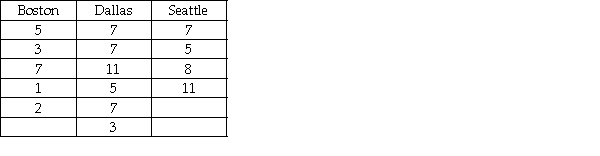
(True/False)
4.9/5  (30)
(30)
A national car rental company recently conducted a study recently in which cars with automatic and standard transmissions (factor A Sample)were rented to male and female customers (factor B Columns).Three customers in each category were randomly selected and the miles driven per day was recorded as follows: 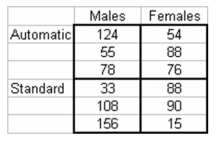 Based on these sample data,and alpha = .05,which of the following statements is true?
Based on these sample data,and alpha = .05,which of the following statements is true?
(Multiple Choice)
4.8/5  (41)
(41)
To test for the stopping distances of the four brake systems,10 of the same make and model of car are selected randomly and then are assigned randomly to each of four brake systems.This is a randomized complete block design.
(True/False)
4.8/5  (33)
(33)
In conducting one-way analysis of variance,the sample size for each group must be equal.
(True/False)
4.7/5  (34)
(34)
A company has established an experiment with its production process in which three temperature settings are used and five elapsed times are used for each setting.The company then produces one product under each and measures the resulting strength of the product.The managers are mainly interested in determining whether the mean strength is the same at all temperature settings,but they know that controlling for process time is important.The following data were observed from the experiment: 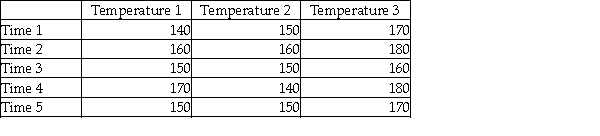 Based on these data and experimental design,for a significance level of 0.05,the managers should conclude that they were justified in blocking on the basis of processing time.
Based on these data and experimental design,for a significance level of 0.05,the managers should conclude that they were justified in blocking on the basis of processing time.
(True/False)
4.7/5  (35)
(35)
Three brands of running shoes are each tested by 10 different runners.The amount of wear on the sole of the shoes is then measured.The objective is to determine if there is any difference among the three brands of shoes based on how long the soles last.The degrees of freedom for testing whether there is any blocking effect D1 = 9 and D2 = 18.
(True/False)
4.8/5  (38)
(38)
In a two-factor ANOVA,the total sum of squares can be partitioned into four parts; the variation due to factor A,the variation due to factor B,the variation due to blocking,and the error variation.
(True/False)
4.9/5  (44)
(44)
As a step in establishing its rates,an automobile insurance company is interested in determining whether there is a difference in the mean highway speeds that drivers of different age groups drive.To help answer this question,it has selected a random sample of drivers in three age categories: under 21,21-50,and over 50.The engineers then recorded the drivers' speeds at a designated point on a highway in the state.The subjects were unaware that their speed was being recorded.The following one-way ANOVA output was generated from the sample data.Based upon this output,if the significance level is 0.05,the engineers should conclude that the mean speeds may all be equal since the p-value is less than alpha.
Anova: Single Factor
SUMMARY  ANOVA
ANOVA 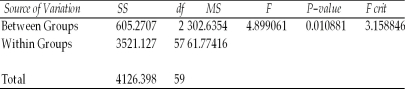
(True/False)
4.9/5  (45)
(45)
Six food critics each visited and rated four different restaurants.Each critic visited each restaurant on three separate occasions and recorded a score for each visit.The critical value for testing whether there is any difference among the four restaurants,using the 0.05 level of significance is approximately F = 2.8.
(True/False)
4.8/5  (31)
(31)
Explain what is meant by interaction between two factors in a two-way analysis of variance study.
(Essay)
4.9/5  (38)
(38)
Showing 1 - 20 of 137
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)