Exam 11: Hypothesis Tests and Estimation for Population Variances
Exam 1: The Where, why, and How of Data Collection167 Questions
Exam 2: Graphs,charts and Tablesdescribing Your Data138 Questions
Exam 3: Describing Data Using Numerical Measures130 Questions
Exam 4: Using Probability and Probability Distributions77 Questions
Exam 5: Discrete Probability Distributions119 Questions
Exam 6: Introduction to Continuous Probability Distributions90 Questions
Exam 7: Introduction to Sampling Distributions104 Questions
Exam 8: Estimating Single Population Parameters145 Questions
Exam 9: Introduction to Hypothesis Testing129 Questions
Exam 10: Estimation and Hypothesis Testing for Two Population Parameters97 Questions
Exam 11: Hypothesis Tests and Estimation for Population Variances71 Questions
Exam 12: Analysis of Variance137 Questions
Exam 13: Goodness-Of-Fit Tests and Contingency Analysis104 Questions
Exam 14: Introduction to Linear Regression and Correlation Analysis136 Questions
Exam 15: Multiple Regression Analysis and Model Building153 Questions
Exam 16: Analyzing and Forecasting Time-Series Data133 Questions
Exam 17: Introduction to Nonparametric Statistics104 Questions
Exam 18: Introduction to Quality and Statistical Process Control110 Questions
Exam 19: Introduction to Decision Analysis116 Questions
Select questions type
One of the major automobile makers has developed two new engines.At question is whether the two engines have the same variability with respect to miles per gallon.To test this,the following information is available:  Based on this situation and the information provided,the appropriate null and alternative hypotheses are:
Based on this situation and the information provided,the appropriate null and alternative hypotheses are: 
Free
(True/False)
4.9/5  (38)
(38)
Correct Answer:
False
The test statistic that is used when testing a null hypothesis for a population variance is the standard normal z-value.
Free
(True/False)
4.8/5  (42)
(42)
Correct Answer:
False
In performing a one-tailed test for the difference between two population variances,which of the following statements is true?
Free
(Multiple Choice)
4.7/5  (36)
(36)
Correct Answer:
B
If we are interested in performing a one-tailed,upper-tail hypothesis test about a population variance where the level of significance is .05 and the sample size is n = 20,the critical chi-square value to be used is 30.1435.
(True/False)
5.0/5  (32)
(32)
For a given significance level,increasing the sample size will tend to increase the chi-square critical value used in testing the null hypothesis about a population variance.
(True/False)
4.8/5  (35)
(35)
There are two major companies that provide SAT test tutoring for high school students.At issue is whether Company 1,which has been in business for the longer time,provides better results than Company 2,the newer company.Specifically of interest is whether the mean increase in SAT scores for students who have already taken the SAT test one time is higher for Company 1 than for Company 2.Two random samples of students are selected.The following data reflect the number of points higher (or lower)that the students scored on the SAT test after taking the tutoring.Prior to conducting the test,which compares the means,we should determine if the assumption of equal variances is supported.
Conduct the appropriate hypothesis test to determine if the assumption of equal variances is supported using a 0.10 level of significance. 
(Essay)
4.8/5  (34)
(34)
One of the major automobile makers has developed two new engines.At question is whether the two engines have the same variability with respect to miles per gallon.To test this using a significance level equal to 0.10,the following information is available:  Based on this situation and the information provided,the critical value is F=4.147 .
Based on this situation and the information provided,the critical value is F=4.147 .
(True/False)
4.8/5  (42)
(42)
A fast food restaurant that sells burritos is concerned about the variability in the amount of filling that different employees place in the burritos.To achieve product consistency it needs this variability to be no more than 1.7 ounces.A sample of n = 18 burritos showed a sample variance of 2.89 ounces.Using a 0.10 level of significance,what can you conclude?
(Multiple Choice)
4.8/5  (31)
(31)
A consulting report that was recently submitted to a company indicated that a hypothesis test for a single population variance was conducted.The report indicated that the test statistic was 34.79,the hypothesized variance was 345 and the sample variance 600.However,the report did not indicate what the sample size was.What was it?
(Multiple Choice)
4.9/5  (42)
(42)
Which distribution is used in testing the hypotheses about the equality of two population variances?
(Multiple Choice)
4.9/5  (39)
(39)
One of the key quality characteristics in many service environments is that the variation in service time be reasonably small.Recently,a major amusement park company initiated a new line system at one of its parks.It then wished to compare this new system with the old system in place at a comparable park in another state.At issue is whether the standard deviation in waiting time is less under the new line system than under the old line system.The following information was collected:  Assuming that it wishes to conduct the test using a 0.05 level of significance,the null hypothesis should be rejected since the test statistic exceeds the F-critical value from the F-distribution table.
Assuming that it wishes to conduct the test using a 0.05 level of significance,the null hypothesis should be rejected since the test statistic exceeds the F-critical value from the F-distribution table.
(True/False)
4.9/5  (36)
(36)
A two-tailed test for two population variances could have a null hypothesis like the following: 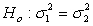 .
.
(True/False)
4.9/5  (28)
(28)
One of the most important aspects of quality improvement is the idea of reducing the variability in a product or service.For instance,a major bank has worked to reduce the variability in the service time at the drive-through.The managers believe that the standard deviation in service time should not exceed 30 seconds.To test whether this goal is being achieved,a random sample of n = 25 cars is selected each week and the service time for each car is measured.Last week,the mean time was 345 seconds with a standard deviation equal to 38 seconds.Given this information,if the significance level is 0.10,the critical value from the chi-square table is about 34.3.
(True/False)
4.9/5  (34)
(34)
Because of the way the F-distribution is formed,all F-tests are one-tailed tests.
(True/False)
4.9/5  (39)
(39)
One of the major automobile makers has developed two new engines.At question is whether the two engines have the same variability with respect to miles per gallon.To test this,the following information is available:  Based on this situation and the information provided,the test statistic is 1.2118.
Based on this situation and the information provided,the test statistic is 1.2118.
(True/False)
4.8/5  (27)
(27)
A potato chip manufacturer has found that in the past the standard deviation of bag weight has been 0.2 ounces.They want to test whether the standard deviation has changed.The null hypothesis is:
(Multiple Choice)
4.9/5  (30)
(30)
In a two-tailed hypothesis test for the difference between two population variances,the test statistic is an F-ratio formed by putting the largest sample variance in numerator.
(True/False)
4.8/5  (32)
(32)
If a one-tailed F-test is employed when testing a null hypothesis about two population variances,the test statistic is an F-value formed by taking the ratio of the two sample variances so that the sample variance predicted to be larger is placed in the numerator.
(True/False)
4.8/5  (32)
(32)
A potato chip manufacturer has two packaging lines and wants to determine if the variances differ between the two lines.They take samples of n1 = 10 bags from line 1 and n2 = 8 bags from line 2.To perform the hypothesis test at the 0.05 level of significance,the critical value is F = 3.68.
(True/False)
4.7/5  (39)
(39)
A frozen food company that makes burritos currently has employees making burritos by hand.It is considering purchasing equipment to automate the process and wants to determine if the automated process would result in lower variability of burrito weights.It takes a random sample from each process as shown below.  To conduct a hypothesis test using a 0.05 level of significance,the critical value is 3.347.
To conduct a hypothesis test using a 0.05 level of significance,the critical value is 3.347.
(True/False)
4.9/5  (35)
(35)
Showing 1 - 20 of 71
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)