Exam 6: Continuous Probability Distributions

The average starting salary for this year's graduates at a large university (LU)is $20,000 with a standard deviation of $8,000.Furthermore,it is known that the starting salaries are normally distributed. a.What is the probability that a randomly selected LU graduate will have a starting salary of at least $30,400? b.Individuals with starting salaries of less than $15,600 receive a low income tax break.What percentage of the graduates will receive the tax break? c.What are the minimum and the maximum starting salaries of the middle 95% of the LU graduates? d.If 189 of the recent graduates have salaries of at least $32,240,how many students graduated this year from this university?

(Essay)

4.8/5

(34)

Question 21

The miles-per-gallon obtained by the 1995 model Z cars is normally distributed with a mean of 22 miles-per-gallon and a standard deviation of 5 miles-per-gallon. a.What is the probability that a car will get between 13.35 and 35.1 miles-per-gallon? b.What is the probability that a car will get more than 29.6 miles-per-gallon? c.What is the probability that a car will get less than 21 miles-per-gallon? d.What is the probability that a car will get exactly 22 miles-per-gallon?

(Essay)

4.9/5

(36)

Question 22

An exponential probability distribution

(Multiple Choice)

4.8/5

(32)

Question 23

Exhibit 6-1 The assembly time for a product is uniformly distributed between 6 to 10 minutes. -Refer to Exhibit 6-1.The standard deviation of assembly time (in minutes)is approximately

(Multiple Choice)

4.9/5

(30)

Question 24

The advertised weight on a can of soup is 10 ounces.The actual weight in the cans follows a uniform distribution and varies between 9.3 and 10.3 ounces. a.Give the mathematical expression for the probability density function. b.What is the probability that a can of soup will have between 9.4 and 10.3 ounces? c.What is the mean weight of a can of soup? d.What is the standard deviation of the weight?

(Essay)

5.0/5

(46)

Question 25

The random variable x is known to be uniformly distributed between 70 and 90.The probability of x having a value between 80 to 95 is

(Multiple Choice)

4.9/5

(36)

Question 26

Z is a standard normal random variable.The P (-1.20 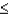Z 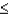1.50)equals

(Multiple Choice)

4.7/5

(29)

Question 27

Given that Z is a standard normal random variable,what is the value of Z if the area to the left of Z is 0.119?

(Multiple Choice)

4.8/5

(33)

Question 28

The Globe Fishery packs shrimp that weigh more than 1.91 ounces each in packages marked" large" and shrimp that weigh less than 0.47 ounces each into packages marked "small";the remainder are packed in "medium" size packages.If a day's catch showed that 19.77 percent of the shrimp were large and 6.06 percent were small,determine the mean and the standard deviation for the shrimp weights.Assume that the shrimps' weights are normally distributed.

(Essay)

4.8/5

(32)

Question 29

The time it takes a worker on an assembly line to complete a task is exponentially distributed with a mean of 8 minutes. a.What is the probability density function for the time it takes to complete the task? b.What is the probability that it will take a worker less than 4 minutes to complete the task? c.What is the probability that it will take a worker between 6 and 10 minutes to complete the task?

(Essay)

4.7/5

(40)

Question 30

Exhibit 6-1 The assembly time for a product is uniformly distributed between 6 to 10 minutes. -Refer to Exhibit 6-1.The probability of assembling the product in less than 6 minutes is

(Multiple Choice)

4.8/5

(40)

Question 31

In grading eggs into small,medium,and large,the Nancy Farms packs the eggs that weigh more than 3.6 ounces in packages marked "large" and the eggs that weigh less than 2.4 ounces into packages marked "small";the remainder are packed in packages marked "medium." If a day's packaging contained 10.2% large and 4.18% small eggs,determine the mean and the standard deviation for the eggs' weights.Assume that the distribution of the weights is normal.

(Essay)

4.7/5

(30)

Question 32

Z is a standard normal random variable.The P(-1.5 < Z < 1.09)equals

(Multiple Choice)

4.8/5

(31)

Question 33

A negative value of Z indicates that

(Multiple Choice)

4.8/5

(34)

Question 34

The length of time patients must wait to see a doctor in a local clinic is uniformly distributed between 15 minutes and 2 1/2 hours. a.What is the probability of a patient waiting exactly 50 minutes? b.What is the probability that a patient would have to wait between 45 minutes and 2 hours? c.Compute the probability that a patient would have to wait over 2 hours. d.Determine the expected waiting time and its standard deviation.

(Essay)

4.9/5

(40)

Question 35

Exhibit 6-9 The average price of personal computers manufactured by MNM Company is $1,200 with a standard deviation of $220.Furthermore,it is known that the computer prices manufactured by MNM are normally distributed. -Refer to Exhibit 6-9.If 513 of the MNM computers were priced at or below $647.80,how many computers were produced by MNM?

(Multiple Choice)

4.7/5

(41)

Question 36

Exhibit 6-1 The assembly time for a product is uniformly distributed between 6 to 10 minutes. -Refer to Exhibit 6-1.The expected assembly time (in minutes)is

(Multiple Choice)

5.0/5

(31)

Question 37

Approximate the following binomial probabilities by the use of normal approximation. a.P(X = 18,n = 50,p = 0.3) b.P(X $\le$ 15,n = 50,p = 0.3) c.P(X $\le$ 12,n = 50,p = 0.3) d.P(12 $\le$ X $\le$ 18,n = 50,p = 0.3)

(Essay)

4.8/5

(35)

Question 38

For a normal distribution,a negative value of z indicates

(Multiple Choice)

4.7/5

(33)

Question 39

A normal probability distribution

(Multiple Choice)

4.8/5

(30)

Question 40

Showing 21 - 40 of 165

An exponential probability distribution

Exhibit 6-1 The assembly time for a product is uniformly distributed between 6 to 10 minutes. -Refer to Exhibit 6-1.The standard deviation of assembly time (in minutes)is approximately

The random variable x is known to be uniformly distributed between 70 and 90.The probability of x having a value between 80 to 95 is

Z is a standard normal random variable.The P (-1.20 Z 1.50)equals

Given that Z is a standard normal random variable,what is the value of Z if the area to the left of Z is 0.119?

Exhibit 6-1 The assembly time for a product is uniformly distributed between 6 to 10 minutes. -Refer to Exhibit 6-1.The probability of assembling the product in less than 6 minutes is

Z is a standard normal random variable.The P(-1.5 < Z < 1.09)equals

A negative value of Z indicates that

Exhibit 6-1 The assembly time for a product is uniformly distributed between 6 to 10 minutes. -Refer to Exhibit 6-1.The expected assembly time (in minutes)is

Approximate the following binomial probabilities by the use of normal approximation. a.P(X = 18,n = 50,p = 0.3) b.P(X $\le$ 15,n = 50,p = 0.3) c.P(X $\le$ 12,n = 50,p = 0.3) d.P(12 $\le$ X $\le$ 18,n = 50,p = 0.3)

For a normal distribution,a negative value of z indicates

A normal probability distribution

Data and Statistics

Descriptive Statistics: Tabular and Graphical Presentations

Descriptive Statistics: Numerical Measures

Introduction to Probability

Discrete Probability Distributions

Sampling and Sampling Distributions

Interval Estimation

Hypothesis Tests

Statistical Inference About Means and Proportions With Two Populations

Inferences About Population Variances

Tests of Goodness of Fit and Independence

Analysis of Variance and Experimental Design

Simple Linear Regression

Multiple Regression

Regression Analysis: Model Building

Index Numbers

Forecasting

Nonparametric Methods

Statistical Methods for Quality Control

Decision Analysis

Sample Survey

Filters

Exam 6: Continuous Probability Distributions

An exponential probability distribution

Exhibit 6-1 The assembly time for a product is uniformly distributed between 6 to 10 minutes. -Refer to Exhibit 6-1.The standard deviation of assembly time (in minutes)is approximately

The random variable x is known to be uniformly distributed between 70 and 90.The probability of x having a value between 80 to 95 is

Z is a standard normal random variable.The P (-1.20 Z 1.50)equals

Given that Z is a standard normal random variable,what is the value of Z if the area to the left of Z is 0.119?

Exhibit 6-1 The assembly time for a product is uniformly distributed between 6 to 10 minutes. -Refer to Exhibit 6-1.The probability of assembling the product in less than 6 minutes is

Z is a standard normal random variable.The P(-1.5 < Z < 1.09)equals

A negative value of Z indicates that

Exhibit 6-1 The assembly time for a product is uniformly distributed between 6 to 10 minutes. -Refer to Exhibit 6-1.The expected assembly time (in minutes)is

Approximate the following binomial probabilities by the use of normal approximation. a.P(X = 18,n = 50,p = 0.3) b.P(X ≤\le≤ 15,n = 50,p = 0.3) c.P(X ≤\le≤ 12,n = 50,p = 0.3) d.P(12 ≤\le≤ X ≤\le≤ 18,n = 50,p = 0.3)

For a normal distribution,a negative value of z indicates

A normal probability distribution

Data and Statistics

Descriptive Statistics: Tabular and Graphical Presentations

Descriptive Statistics: Numerical Measures

Introduction to Probability

Discrete Probability Distributions

Sampling and Sampling Distributions

Interval Estimation

Hypothesis Tests

Statistical Inference About Means and Proportions With Two Populations

Inferences About Population Variances

Tests of Goodness of Fit and Independence

Analysis of Variance and Experimental Design

Simple Linear Regression

Multiple Regression

Regression Analysis: Model Building

Index Numbers

Forecasting

Nonparametric Methods

Statistical Methods for Quality Control

Decision Analysis

Sample Survey

Filters

Approximate the following binomial probabilities by the use of normal approximation. a.P(X = 18,n = 50,p = 0.3) b.P(X $\le$ 15,n = 50,p = 0.3) c.P(X $\le$ 12,n = 50,p = 0.3) d.P(12 $\le$ X $\le$ 18,n = 50,p = 0.3)