Exam 6: Continuous Probability Distributions
Exam 1: Data and Statistics98 Questions
Exam 2: Descriptive Statistics: Tabular and Graphical Presentations64 Questions
Exam 3: Descriptive Statistics: Numerical Measures156 Questions
Exam 4: Introduction to Probability138 Questions
Exam 5: Discrete Probability Distributions122 Questions
Exam 6: Continuous Probability Distributions165 Questions
Exam 7: Sampling and Sampling Distributions131 Questions
Exam 8: Interval Estimation131 Questions
Exam 9: Hypothesis Tests133 Questions
Exam 10: Statistical Inference About Means and Proportions With Two Populations121 Questions
Exam 11: Inferences About Population Variances91 Questions
Exam 12: Tests of Goodness of Fit and Independence80 Questions
Exam 13: Analysis of Variance and Experimental Design113 Questions
Exam 14: Simple Linear Regression140 Questions
Exam 15: Multiple Regression106 Questions
Exam 16: Regression Analysis: Model Building75 Questions
Exam 17: Index Numbers52 Questions
Exam 18: Forecasting67 Questions
Exam 19: Nonparametric Methods81 Questions
Exam 20: Statistical Methods for Quality Control30 Questions
Exam 21: Decision Analysis65 Questions
Exam 22: Sample Survey63 Questions
Select questions type
Given that Z is a standard normal random variable,what is the value of Z if the area to the right of Z is 0.5?
(Multiple Choice)
4.7/5  (31)
(31)
The function that defines the probability distribution of a continuous random variable is a
(Multiple Choice)
4.9/5  (33)
(33)
Given that Z is a standard normal random variable,what is the probability that -2.08 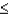 Z
Z 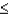 1.46?
1.46?
(Multiple Choice)
4.9/5  (36)
(36)
Given that Z is a standard normal random variable,what is the value of Z if the area between -Z and Z is 0.901?
(Multiple Choice)
4.9/5  (37)
(37)
The time required to assemble a part of a machine follows an exponential probability distribution with a mean of 14 minutes.
a.What is the probability that the part can be assembled in 7 minutes or less?
b.What is the probability that the part can be assembled between 3.5 and 7 minutes?
(Essay)
4.8/5  (35)
(35)
Exhibit 6-2
The weight of football players is normally distributed with a mean of 200 pounds and a standard deviation of 25 pounds.
-Refer to Exhibit 6-2.The probability of a player weighing more than 241.25 pounds is
(Multiple Choice)
4.9/5  (33)
(33)
The life expectancy of computer terminals is normally distributed with a mean of 4 years and a standard deviation of 10 months.
a.What is the probability that a randomly selected terminal will last more than 5 years?
b.What percentage of terminals will last between 5 and 6 years?
c.What percentage of terminals will last less than 4 years?
d.What percentage of terminals will last between 2.5 and 4.5 years?
e.If the manufacturer guarantees the terminals for 3 years (and will replace them if they malfunction),what percentage of terminals will be replaced?
(Essay)
4.8/5  (30)
(30)
A local bank has determined that the daily balances of the checking accounts of its customers are normally distributed with an average of $280 and a standard deviation of $20.
a.What percentage of its customers has daily balances of more than $275?
b.What percentage of its customers has daily balances less than $243?
c.What percentage of its customers' balances is between $241 and $301.60?
(Essay)
4.9/5  (36)
(36)
Exhibit 6-7
The weight of items produced by a machine is normally distributed with a mean of 8 ounces and a standard deviation of 2 ounces.
-Refer to Exhibit 6-7.What percentage of items will weigh at least 11.7 ounces?
(Multiple Choice)
4.9/5  (35)
(35)
Exhibit 6-6
The starting salaries of individuals with an MBA degree are normally distributed with a mean of $40,000 and a standard deviation of $5,000.
-Refer to Exhibit 6-6.What is the probability that a randomly selected individual with an MBA degree will get a starting salary of at least $47,500?
(Multiple Choice)
4.7/5  (43)
(43)
Exhibit 6-5
The travel time for a college student traveling between her home and her college is uniformly distributed between 40 and 90 minutes.
-Refer to Exhibit 6-5.The probability that her trip will take longer than 60 minutes is
(Multiple Choice)
4.9/5  (27)
(27)
The time it takes a mechanic to change the oil in a car is exponentially distributed with a mean of 5 minutes.
a.What is the probability density function for the time it takes to change the oil?
b.What is the probability that it will take a mechanic less than 6 minutes to change the oil?
c.What is the probability that it will take a mechanic between 3 and 5 minutes to change the oil?
d.What is the variance of the time it takes to change the oil?
(Essay)
4.7/5  (38)
(38)
The Mathematics part of the SAT scores of students at UTC are normally distributed with a mean of 500 and a standard deviation of 75.
a.If 2.28 percent of the students who had the highest scores received scholarships,what was the minimum score among those who received scholarships? Do not round your answer.
b.It is known that 6.3 percent of students who applied to UTC were not accepted.What is the highest score of those who were denied acceptance? Do not round your answer.
c.What percentage of students had scores between 575 and 650?
(Essay)
4.8/5  (34)
(34)
Which of the following is not a characteristic of the normal probability distribution?
(Multiple Choice)
5.0/5  (37)
(37)
The ticket sales for events held at the new civic center are believed to be normally distributed with a mean of 12,000 and a standard deviation of 1,000.
a.What is the probability of selling more than 10,000 tickets?
b.What is the probability of selling between 9,500 and 11,000 tickets?
c.What is the probability of selling more than 13,500 tickets?
(Essay)
4.9/5  (28)
(28)
The probability that a continuous random variable takes any specific value
(Multiple Choice)
4.8/5  (33)
(33)
When a continuous probability distribution is used to approximate a discrete probability distribution
(Multiple Choice)
4.8/5  (34)
(34)
Z is a standard normal random variable.The P(1.05 < Z < 2.13)equals
(Multiple Choice)
4.9/5  (35)
(35)
The First National Mortgage Company has noted that 6% of its customers pay their mortgage payments after the due date.
a.What is the probability that in a random sample of 150 customers 7 will be late on their payments?
b.What is the probability that in a random sample of 150 customers at least 10 will be late on their payments?
(Essay)
4.9/5  (35)
(35)
Showing 61 - 80 of 165
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)