Exam 7: Introduction to Probability
Exam 1: Linear Equations and Functions245 Questions
Exam 2: Quadratic and Other Special Functions120 Questions
Exam 3: Matrices230 Questions
Exam 4: Inequalities and Linear Programming119 Questions
Exam 5: Exponential and Logarithmic Functions109 Questions
Exam 6: Mathematics of Finance131 Questions
Exam 7: Introduction to Probability180 Questions
Exam 8: Further Topics in Probability and Data Description114 Questions
Exam 9: Derivatives249 Questions
Exam 10: Derivatives172 Questions
Exam 11: Derivatives Continued139 Questions
Exam 12: Indefinite Integrals120 Questions
Exam 13: Definite Integrals - Techniques370 Questions
Exam 13: A: Definite Integrals - Techniques370 Questions
Exam 14: Functions of Two or More Variables122 Questions
Exam 15: Algebraic Concepts 240 Questions
Exam 15: Algebraic Concepts 374 Questions
Exam 15: Algebraic Concepts 496 Questions
Exam 15: Algebraic Concepts 599 Questions
Select questions type
Six men and four women are semifinalists in a lottery. From this group, three finalists are to be selected by a drawing. What is the probability that all four finalists will be men? Round your answer to three decimal places.
(Multiple Choice)
5.0/5  (45)
(45)
An examination consists of 11 questions. If 6 questions must be answered, find the number of different orders in which a student can answer the questions.
(Multiple Choice)
4.8/5  (35)
(35)
According to a recent medical study, 196 of 200 mammograms turn out to be normal. What is the probability that the mammogram of a woman chosen at random will show an abnormality?
(Multiple Choice)
4.8/5  (35)
(35)
From a deck of 52 playing cards, two cards are drawn, one after the other without replacement. What is the probability that the first will be an ace nd the second will be an ace?
(Multiple Choice)
4.9/5  (42)
(42)
An unprepared student must take a 7-question, multiple-choice test that has 5 possible answers per question. If the student can eliminate two of the possible answers on the first three questions, and if she guesses on every question, what is the probability that she will answer at least one question correctly?
(Multiple Choice)
4.9/5  (44)
(44)
A bag contains 7 white balls and 8 red balls. Construct a probability tree to determine the probability that if 2 balls are drawn,with replacement, the first ball is red R and the second is white W.
(Multiple Choice)
4.9/5  (42)
(42)
If the odds that a particular horse will win a race are 15 : 9, what is the probability that the horse will win the race?
(Multiple Choice)
4.9/5  (38)
(38)
A bag contains 4 red balls and 11 white balls. Two balls are drawn from the bag. What is the probability that the second ball is white W, given that the first ball is red and is replaced before the second is drawn?
(Multiple Choice)
4.7/5  (33)
(33)
A fair die is rolled. Find the probability that the result is a 4, given that the result is even.
(Multiple Choice)
4.8/5  (40)
(40)
The following table gives the percent of employees of the Ace Company in each of three salary brackets, categorized by the sex of the employees. An employee is selected at random. What is the probability that the person selected is female and makes less than $30,000?
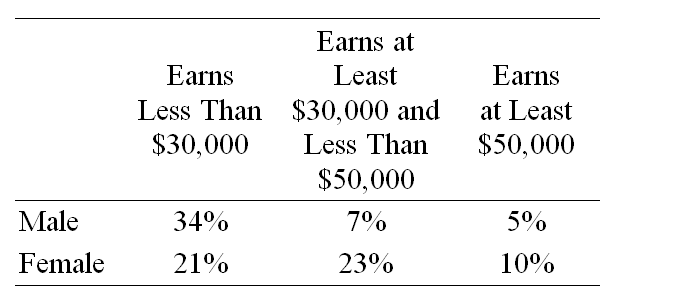
(Multiple Choice)
4.7/5  (32)
(32)
An urn contains 8 red, 9 white, and 2 black balls. One ball is drawn from the urn, it is replaced, and a second ball is drawn. Construct a probability tree to determine the probability that at least one ball drawn is black B.
(Multiple Choice)
4.8/5  (30)
(30)
Two balls are drawn, without replacement, from a bag containing 18 blue balls numbered 1-18 and 4 green balls numbered 19-22. What is the probability that the first ball is blue and even-numbered and the second ball is even-numbered?
(Multiple Choice)
4.9/5  (29)
(29)
A red ball and 17 white balls are in a box. If two balls are drawn, without replacement, what is the probability of getting 2 red R balls?
(Multiple Choice)
4.9/5  (29)
(29)
A company estimates that 50% of the country has seen its commercial and that if a person sees its commercial, there is a 45% probability that the person will buy its product. What is the probability that a person chosen at random in the country will have seen the commercial and bought the product? Round your answer to four decimal places.
(Multiple Choice)
4.8/5  (39)
(39)
Use the transition matrix  and initial probability vector
and initial probability vector  to find the resulting third probability vector. Round all numerical values in your answer to three decimal places.
to find the resulting third probability vector. Round all numerical values in your answer to three decimal places.
(Multiple Choice)
4.9/5  (31)
(31)
To see whether a bank has enough minority construction company loans, a social agency selects 31 loans to construction companies at random and finds that 4 of them are loans to minority companies. If the bank's claim that 10 of every 100 of its loans to construction companies are minority loans is true, what is the probability that 4 loans out of 31 are minority loans? Leave your answer with combination symbols.
(Multiple Choice)
5.0/5  (38)
(38)
An urn contains three red R balls numbered 1, 2 and 3, four white W balls numbered 4, 5, 6 and 7, and three black B balls numbered 8, 9 and 10. A ball is drawn from the urn. What is the probability that it is red?
(Multiple Choice)
4.8/5  (38)
(38)
Thirty-seven percent of the cars owned by a car rental firm have some defect. What is the probability that if 3 cars are selected at random, at least one would have a defect? Round your answer to four decimal places.
(Multiple Choice)
4.7/5  (34)
(34)
A card is drawn from an ordinary deck of 52 playing cards. What is the probability that it will be a jack (J), queen (Q), or king (K)?
(Multiple Choice)
4.8/5  (38)
(38)
A mathematics class consists of 14 engineering majors E, 8 science majors S, and 15 liberal arts majors LA. What is the probability that a student selected at random will be an engineering or science major?
(Multiple Choice)
4.8/5  (37)
(37)
Showing 41 - 60 of 180
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)