Exam 13: Inference for Regression

The following is a plot of the residuals versus fat for 26 menu items at a fast food restaurant. 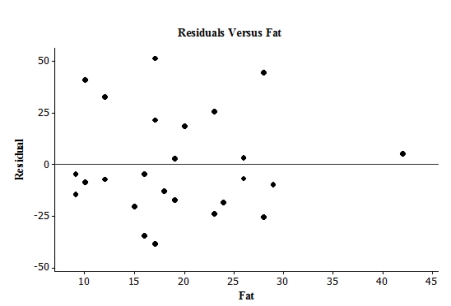Which of the following statements is supported by this plot?

The following is a scatterplot of a company's profits versus their sales (in dollars). Each point on the plot represents profits and sales during one of the months in the sample. 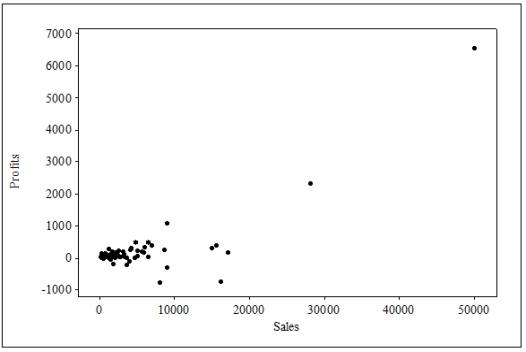Assume that the point in the far upper right was erroneous, resulting from inaccurate data entry. Which of the following statements will be correct once that point is removed from the regression analysis?

A study of obesity risk in children in a head start program used a food score calculated from a 45-question food survey to predict body mass index (BMI) percentile in these children 18 months after the initial survey. The study enrolled 130 children. The researchers used a linear regression model for the prediction of BMI percentile. Which of the following is not a condition for linear regression?

A study of obesity risk in children in a head start program used a food score calculated from a 45-question food survey to predict body mass index (BMI) percentile in these children 18 months after the initial survey. The study enrolled 130 children. The researchers used a linear regression model for the prediction of BMI percentile. The food scores ranged from 45 to 245. Fifteen children had a food score of 170. A boxplot of those 15 children showed BMI percentile to be very skewed. This information is:

You can visit the official website of any large restaurant chain to examine the nutritional data for menu items. For fast-food restaurants, many menu items are high in fat, so most of their calorie content comes from fat (rather than from carbohydrates or protein). Here we investigate the relationship between the amount of fat in a menu item (in grams) and the number of calories. To predict the number of calories in a menu item given its fat content, we use the simple linear regression model calories =  +  (fat), where the deviations are assumed to be independent and Normally distributed, with mean 0 and standard deviation . At one major fast-food restaurant chain, there were 26 items listed under the heading of "Sandwiches" (which includes hamburgers, chicken sandwiches, and other sandwich selections) on the menu. We fit the model to the data using the method of least squares. We treat these 26 menu items (which came from one restaurant) as a sample from the population of all sandwich items at all fast-food restaurants. This assumption is probably dubious. The following results were obtained from software. r² = 0.846 S = 43.5747 Suppose the researchers test the hypotheses H₀:  = 0, H_a:  > 0. The value of the t statistic for this test is:

Frequent food questionnaires (FFQs) are often given to large groups of people to obtain information on their dietary habits. Study participants are asked about the frequency with which they consume certain goods. Another method to obtain information on foods consumed is a food diary. People are asked to record every type of food and amount consumed for a few days. Food diaries are more difficult to obtain and response rates are lower than for FFQs. A study was conducted to see how well frequent food questionnaires predict food consumed based on food diaries. The regression of alcohol consumption FFQ on alcohol consumption food diary had an intercept of a = 2.96 and a slope of b = 0.67. The predicted number of drinks consumed in the food diary, if the FFQ states 5 drinks, is:

Forced expiratory volume (FEV) is the volume of exhaled air and is related to lung size as well as lung function. It is typically lower in persons with impaired lung function due to disease. A regression of height (in inches) on FEV (in liters) showed a nonlinear pattern. Therefore, height was regressed on log(FEV) (natural log) and the residual plot showed a much improved fit. The intercept a and slope b from the regression of height on log(FEV) are a = -2.2 and b = 0.06. The correlation for this regression is:

Suppose we wish to predict the profits (in hundreds of thousands of dollars) for companies that had sales (in hundreds of thousands of dollars) of 500 units. We use statistical software to do the prediction and obtain the following output. 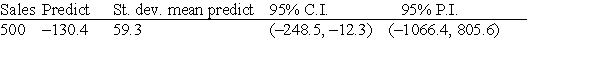A random sample of 19 companies from the Forbes 500 list was selected, and the relationship between sales (in hundreds of thousands of dollars) and profits (in hundreds of thousands of dollars) was investigated by regression. The following simple linear regression model was used: profits =  +  (sales), where the deviations were assumed to be independent and Normally distributed, with mean 0 and standard deviation . This model was fit to the data using the method of least squares. The following results were obtained from statistical software. r² = 0.662 S = 466.2 A 95% confidence interval for the average profit of companies with 500 units of sales is:

A study of obesity risk in children in a head start program used a food score calculated from a 45-question food survey to predict body mass index (BMI) percentile in these children 18 months after the initial survey. The study enrolled 20 children. The researchers used a linear regression model for the prediction of BMI percentile. The food scores ranged from 45 to 245. A regression model has:

A study of obesity risk in children in a head start program used a food score calculated from a 45-question food survey to predict body mass index (BMI) percentile in these children 18 months after the initial survey. The study enrolled 20 children. The researchers used a linear regression model for the prediction of BMI percentile. The food scores ranged from 45 to 245. "Linear" refers to which of the following?

Suppose we wish to predict the profits (in hundreds of thousands of dollars) for companies that had sales (in hundreds of thousands of dollars) of 500 units. We use statistical software to do the prediction and obtain the following output. 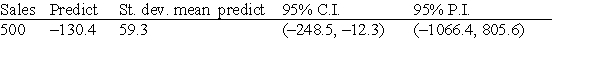A random sample of 19 companies from the Forbes 500 list was selected, and the relationship between sales (in hundreds of thousands of dollars) and profits (in hundreds of thousands of dollars) was investigated by regression. The following simple linear regression model was used: profits =  +  (sales), where the deviations were assumed to be independent and Normally distributed, with mean 0 and standard deviation . This model was fit to the data using the method of least squares. The following results were obtained from statistical software. r² = 0.662 S = 466.2 A 95% interval for this prediction is:

You can visit the official website of any large restaurant chain to examine the nutritional data for menu items. For fast-food restaurants, many menu items are high in fat, so most of their calorie content comes from fat (rather than from carbohydrates or protein). Here we investigate the relationship between the amount of fat in a menu item (in grams) and the number of calories. To predict the number of calories in a menu item given its fat content, we use the simple linear regression model calories =  +  (fat), where the deviations are assumed to be independent and Normally distributed, with mean 0 and standard deviation . At one major fast-food restaurant chain, there were 26 items listed under the heading of "Sandwiches" (which includes hamburgers, chicken sandwiches, and other sandwich selections) on the menu. We fit the model to the data using the method of least squares. We treat these 26 menu items (which came from one restaurant) as a sample from the population of all sandwich items at all fast-food restaurants. This assumption is probably dubious. The following results were obtained from software. r² = 0.846 S = 43.5747 The slope of the least-squares regression line is:

The scatterplot below suggests a linear relationship between the age (in years) of an antique clock and its sale price (in euros) at auction. The data are age and sale price for 11 antique clocks sold at a recent auction. We fit the least-squares regression line to the model price =  +  (age), where the deviations are assumed to be independent and Normally distributed, with mean 0 and standard deviation . A summary of the output is given. R² = 0.848 S = 33.1559 The approximate intercept of the least-squares regression line is:

A study of obesity risk in children in a head start program used a food score calculated from a 45-question food survey to predict body mass index (BMI) percentile in these children 18 months after the initial survey. The study enrolled 20 children. The researchers used a linear regression model for the prediction of BMI percentile with food scores ranging between 45 and 245. The least-squares estimates were slope = 0.29 and intercept = 18.3. If child A has a food score of 145 and child B has a food score of 180, then the difference in BMI percentile between child B and child A is:

A random sample of 19 companies from the Forbes 500 list was selected, and the relationship between sales (in hundreds of thousands of dollars) and profits (in hundreds of thousands of dollars) was investigated by regression. The following simple linear regression model was used: profits =  +  (sales), where the deviations were assumed to be independent and Normally distributed, with mean 0 and standard deviation . This model was fit to the data using the method of least squares. The following results were obtained from statistical software. r² = 0.662 S = 466.2 An approximate 90% confidence interval for the slope  in the simple linear regression model is:

You can visit the official website of any large restaurant chain to examine the nutritional data for menu items. For fast-food restaurants, many menu items are high in fat, so most of their calorie content comes from fat (rather than from carbohydrates or protein). Here we investigate the relationship between the amount of fat in a menu item (in grams) and the number of calories. To predict the number of calories in a menu item given its fat content, we use the simple linear regression model calories =  +  (fat), where the deviations are assumed to be independent and Normally distributed, with mean 0 and standard deviation . The explanatory variable in this study is: