Exam 9: Random Variables and Probability Distributions

It is assumed that the time between failures for an electronic component are exponentially distributed with a mean of 50 hours between consecutive failures. Based on this information, what is the probability that a randomly selected part will fail in less than 10 hours?

A shareholder believes that in one year, there is a 20% chance that his stock will be worth $75, a 50% chance that it will be worth $100, and a 30% chance that it will be worth $140. a. Find the stock's expected value in one year. b. Find the standard deviation of the stock's worth in one year.

The probability model for random variable X is specified as: P (X = x) = 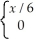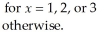The expected value of X is

A company's manufacturing process uses 500 litres of water at a time. A "scrubbing" machine then removes most of a chemical pollutant before pumping the water into a nearby lake. To meet federal regulations the treated water must not contain more than 80 parts per million (ppm) of the chemical. Because there is a fine charged if regulations are not met, the company sets the machine to attain an average of 75 ppm in the treated water. The machine's output can be described by a Normal model with a standard deviation 4.2 ppm. a. What percent of the batches of water discharged exceed the 80 ppm standard? b. The company's lawyers insist that not more than 2% of the treated water should be over the limit. In order to achieve this, to what mean should the company set the scrubbing machine? Assume the standard deviation does not change.

Consider the following to answer the question(s) below: A company believes that there will be a 50% chance of making a profit of $1,000, a 30% chance of making a profit of $1500 and a 20% chance of making a profit of $2,000. -What is the expected profit?

Consider the following to answer the question(s) below: An airline has found that its flights between Toronto and Vancouver can take anywhere from 4.5 to 7.5 hours, with any time between this range being equally likely. -What is the probability that the flight will take more than 7 hours?

Consider the following to answer the question(s) below: A courier service claims that only 5 % of all of its deliveries arrive late. Assuming deliveries are independent, a sample of 10 deliveries is randomly selected. -What is the probability that more than 2 of the sample deliveries arrive late?

The owner of a small convenience store is trying to decide whether to discontinue selling magazines. He suspects that only 5% of the customers buy magazines and thinks that he might be able to sell something more profitable. Before making a final decision, he keeps track of the number of customers who buy magazines on a given day. a. On one day, he had 280 customers. Assuming this day was typical, what would be the mean and standard deviation of the number of customers who buy magazines each day? b. Surprised by the high number of customers who purchased magazines that day, the owner decided that his 5% estimate must have been too low. How many magazine sales would it have taken to convince you? Justify your answer.