Exam 17: Second Order Differential Equations
Exam 1: Preliminaries101 Questions
Exam 2: Limits and Continuity105 Questions
Exam 3: Differentiation116 Questions
Exam 4: Applications of the Derivative118 Questions
Exam 5: Integration129 Questions
Exam 6: Applications of the Definite Integral85 Questions
Exam 7: Exponentials, Logarithms and Other Transcendental Functions66 Questions
Exam 8: Integration Techniques123 Questions
Exam 9: First-Order Differential Equations72 Questions
Exam 10: Infinite Series111 Questions
Exam 11: Parametric Equations and Polar Coordinates129 Questions
Exam 12: Vectors and the Geometry of Space107 Questions
Exam 13: Vector-Valued Functions103 Questions
Exam 14: Functions of Several Variables and Partial Differentiation112 Questions
Exam 15: Multiple Integrals92 Questions
Exam 16: Vector Calculus67 Questions
Exam 17: Second Order Differential Equations38 Questions
Select questions type
Identify the form of a particular solution to the equation 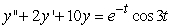 .
.
Free
(Multiple Choice)
4.7/5  (31)
(31)
Correct Answer:
A
Find the general solution of 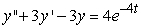 , given the particular solution
, given the particular solution 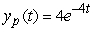 .
.
(Multiple Choice)
4.8/5  (27)
(27)
Identify the pair of graphs that correspond most closely to the solutions of ![Identify the pair of graphs that correspond most closely to the solutions of with , respectively. [The function y(t) is plotted on the vertical axes and t is plotted on the horizontal axes.]](https://storage.examlex.com/TB5869/11eaa88b_9285_6ed3_a696_812bb9a4f027_TB5869_11.jpg) with
with ![Identify the pair of graphs that correspond most closely to the solutions of with , respectively. [The function y(t) is plotted on the vertical axes and t is plotted on the horizontal axes.]](https://storage.examlex.com/TB5869/11eaa88b_9285_95e4_a696_4555f46e304f_TB5869_00.jpg) , respectively. [The function y(t) is plotted on the vertical axes and t is plotted on the horizontal axes.]
, respectively. [The function y(t) is plotted on the vertical axes and t is plotted on the horizontal axes.]
(Multiple Choice)
4.7/5  (27)
(27)
For a pendulum of weight 4 pounds, length 0.60 ft, damping constant ![For a pendulum of weight 4 pounds, length 0.60 ft, damping constant and forcing function find the amplitude and period of the steady-state motion. [The acceleration due to gravity is 32 ft s<sup>-2</sup>.]](https://storage.examlex.com/TB5869/11eaa88b_928b_144c_a696_a3f61fd1345f_TB5869_11.jpg) and forcing function
and forcing function ![For a pendulum of weight 4 pounds, length 0.60 ft, damping constant and forcing function find the amplitude and period of the steady-state motion. [The acceleration due to gravity is 32 ft s<sup>-2</sup>.]](https://storage.examlex.com/TB5869/11eaa88b_928b_3b5d_a696_d503ee1558f8_TB5869_11.jpg) find the amplitude and period of the steady-state motion. [The acceleration due to gravity is 32 ft s-2.]
find the amplitude and period of the steady-state motion. [The acceleration due to gravity is 32 ft s-2.]
(Multiple Choice)
4.8/5  (29)
(29)
A 2.0 kg mass hangs on a spring with a 0.5 newton/meter force constant and its motion is not damped. If the system is subjected to an external variable-frequency vibration described as 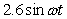 newtons, at what frequency,
newtons, at what frequency, 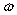 , will the external vibration and the spring system be in resonance?
, will the external vibration and the spring system be in resonance?
(Multiple Choice)
4.9/5  (41)
(41)
For 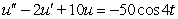 find the steady-state solution and identify its amplitude and phase shift.
find the steady-state solution and identify its amplitude and phase shift.
(Essay)
4.9/5  (35)
(35)
A series circuit has a 0.25 henry inductor, a 500 ohm resistor, and a 0.000004 farad capacitor. There is an initial charge of 0.000001 coulombs, there is no initial current, and there is an applied voltage which is described as 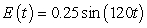 . Identify the solution to the differential equation that describes the charge on the capacitor as a function of time.
. Identify the solution to the differential equation that describes the charge on the capacitor as a function of time.
(Multiple Choice)
4.7/5  (30)
(30)
A series circuit has a 0.1 henry inductor, a 310 ohm resistor, and a 0.000008 farad capacitor. There is an initial charge of 0.000003 coulombs, there is no initial current, and there is an applied voltage which is described as 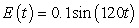 . Identify the solution to the differential equation that describes the charge on the capacitor as a function of time.
. Identify the solution to the differential equation that describes the charge on the capacitor as a function of time.
(Multiple Choice)
4.9/5  (48)
(48)
Identify the general solution of the the differential equation 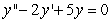 .
.
(Multiple Choice)
4.9/5  (30)
(30)
Identify the radius of convergence of the power series solutions about x = 0 of 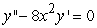 .
.
(Multiple Choice)
4.8/5  (37)
(37)
A pendulum has length 0.22 meter. A bob is released from rest from a starting angle ![A pendulum has length 0.22 meter. A bob is released from rest from a starting angle . Find an equation for the position at any time t and find the amplitude and period of the motion. [The acceleration due to gravity is 9.8 m s<sup>-2</sup>.]](https://storage.examlex.com/TB5869/11eaa88b_928a_c62b_a696_939147aa6696_TB5869_11.jpg) . Find an equation for the position at any time t and find the amplitude and period of the motion. [The acceleration due to gravity is 9.8 m s-2.]
. Find an equation for the position at any time t and find the amplitude and period of the motion. [The acceleration due to gravity is 9.8 m s-2.]
(Multiple Choice)
4.8/5  (41)
(41)
A second order differential equaiton can be arranged to the form 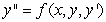 , and one can find the third and higher derivatives of y by simply differentiating this equation. Since a Taylor series expansion of a function y(x) is
, and one can find the third and higher derivatives of y by simply differentiating this equation. Since a Taylor series expansion of a function y(x) is 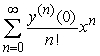 , one can differentiate the rearranged second order differential equation to evaluate coefficients of the Taylor polynomial, if one is either given or can solve for the initial condition y(0) and y'(0). What does the fourth-degree Taylor polynomial look like for the solution to the equation
, one can differentiate the rearranged second order differential equation to evaluate coefficients of the Taylor polynomial, if one is either given or can solve for the initial condition y(0) and y'(0). What does the fourth-degree Taylor polynomial look like for the solution to the equation 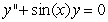 if the initial conditions are
if the initial conditions are 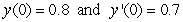 ?
?
(Multiple Choice)
4.7/5  (28)
(28)
Consider solutions to the second order differential equation  in which m, c, and k are positive constants. Which of the following pairs of graphs might correspond to the sets of constants m = 1.00, c = 0.80, and k = 1.20 (blue graph), and m = 1.00, c = 0.08, and k = 1.20 (red graph) ?
in which m, c, and k are positive constants. Which of the following pairs of graphs might correspond to the sets of constants m = 1.00, c = 0.80, and k = 1.20 (blue graph), and m = 1.00, c = 0.08, and k = 1.20 (red graph) ?
(Multiple Choice)
4.7/5  (43)
(43)
A series circuit has a 0.1 henry inductor, a 490 ohm resistor, and a 0.000003 farad capacitor. There is an initial charge of 0.000009 coulombs, there is no initial current, and there is an applied voltage which is described as 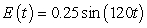 . Identify the steady-state solution to the differential equation.
. Identify the steady-state solution to the differential equation.
(Multiple Choice)
4.7/5  (38)
(38)
Find the general solution of 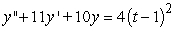 , given the particular solution
, given the particular solution 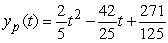 .
.
(Multiple Choice)
4.8/5  (38)
(38)
Showing 1 - 20 of 38
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)