Exam 4: Introduction to Probability
Exam 1: Data and Statistics84 Questions
Exam 2: Descriptive Statistics: Tabular and Graphical Presentations116 Questions
Exam 3: Descriptive Statistics: Numerical Measures130 Questions
Exam 4: Introduction to Probability127 Questions
Exam 5: Discrete Probability Distributions146 Questions
Exam 6: Continuous Probability Distributions138 Questions
Exam 7: Sampling and Sampling Distributions123 Questions
Exam 8: Interval Estimation111 Questions
Exam 9: Hypothesis Tests117 Questions
Exam 10: Comparisons Involving Means, Experimental Design, and Analysis of Variance184 Questions
Exam 11: Comparisons Involving Proportions and a Test of Independence117 Questions
Exam 12: Simple Linear Regression107 Questions
Exam 13: Multiple Regression111 Questions
Exam 14: Statistical Methods for Quality Control72 Questions
Exam 15: Time Series Analysis and Forecastng75 Questions
Select questions type
A committee of 4 is to be selected from a group of 12 people. How many possible committees can be selected?
(Short Answer)
5.0/5  (43)
(43)
A graphical device used for enumerating sample points in a multiple-step experiment is a
(Multiple Choice)
4.8/5  (36)
(36)
Of five letters (A, B, C, D, and E), two letters are to be selected at random. How many possible selections are there?
(Multiple Choice)
4.8/5  (33)
(33)
Since the sun must rise tomorrow, then the probability of the sun rising tomorrow is
(Multiple Choice)
4.9/5  (29)
(29)
A machine is used in a production process. From past data, it is known that 97% of the time the machine is set up correctly. Furthermore, it is known that if the machine is set up correctly, it produces 95% acceptable (non-defective) items. However, when it is set up incorrectly, it produces only 40% acceptable items.
a.An item from the production line is selected. What is the probability that the selected item is non-defective?
b.Given that the selected item is non-defective, what is the probability that the machine is set up correctly?
c.What method of assigning probabilities was used here?
(Essay)
4.7/5  (27)
(27)
A bank has the following data on the gender and marital status of 200 customers.
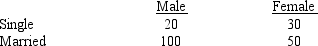 a.What is the probability of finding a single female customer?
b.What is the probability of finding a married male customer?
c.If a customer is female, what is the probability that she is single?
d.What percentage of customers is male?
e.If a customer is male, what is the probability that he is married?
f. Are gender and marital status mutually exclusive?
g. Is marital status independent of gender? Explain using probabilities.
a.What is the probability of finding a single female customer?
b.What is the probability of finding a married male customer?
c.If a customer is female, what is the probability that she is single?
d.What percentage of customers is male?
e.If a customer is male, what is the probability that he is married?
f. Are gender and marital status mutually exclusive?
g. Is marital status independent of gender? Explain using probabilities.
(Essay)
4.9/5  (35)
(35)
The "Top Three" at a racetrack consists of picking the correct order of the first three horses in a race. If there are 10 horses in a particular race, how many "Top Three" outcomes are there?
(Multiple Choice)
4.8/5  (36)
(36)
Revised probabilities of events based on additional information are
(Multiple Choice)
4.9/5  (37)
(37)
If A and B are mutually exclusive events with P(A) 0.3 and P(B) 0.5, then P(A B)
(Multiple Choice)
4.7/5  (34)
(34)
In statistical experiments, each time the experiment is repeated
(Multiple Choice)
4.8/5  (29)
(29)
The following probability model describes the number of snowstorms for Washington, D.C. for a given year:
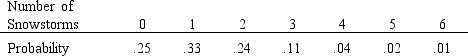 The probability of 7 or more snowstorms in a year is 0.
a. What is the probability of more than 2 but less than 5 snowstorms?
b. Given this a particularly cold year (in which 2 snowstorms have already been observed), what is the conditional probability that 4 or more snowstorms will be observed?
c. If at the beginning of winter there is a snowfall, what is the probability of at least one more snowstorm before winter is over?
The probability of 7 or more snowstorms in a year is 0.
a. What is the probability of more than 2 but less than 5 snowstorms?
b. Given this a particularly cold year (in which 2 snowstorms have already been observed), what is the conditional probability that 4 or more snowstorms will be observed?
c. If at the beginning of winter there is a snowfall, what is the probability of at least one more snowstorm before winter is over?
(Essay)
4.9/5  (32)
(32)
The probability of an intersection of two events is computed using the
(Multiple Choice)
4.7/5  (39)
(39)
If A and B are independent events with P(A) 0.38 and P(B) 0.55, then P(AB)
(Multiple Choice)
4.7/5  (37)
(37)
Two of the cylinders in an eight-cylinder car are defective and need to be replaced. If two cylinders are selected at random, what is the probability that
a.both defective cylinders are selected?
b.no defective cylinder is selected?
c.at least one defective cylinder is selected?
(Essay)
4.9/5  (35)
(35)
A very short quiz has one multiple-choice question with five possible choices (a, b, c, d, e) and one true or false question. Assume you are taking the quiz but do not have any idea what the correct answer is to either question, but you mark an answer anyway.
a.What is the probability that you have given the correct answer to both questions?
b.What is the probability that only one of the two answers is correct?
c.What is the probability that neither answer is correct?
d.What is the probability that only your answer to the multiple-choice question is correct?
e.What is the probability that you have only answered the true or false question correctly?
(Essay)
4.8/5  (33)
(33)
If A and B are mutually exclusive events with P(A) 0.3 and P(B) 0.5, then P(A B)
(Multiple Choice)
4.8/5  (29)
(29)
Showing 101 - 120 of 127
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)