Exam 12: A: linear Regression and Correlation

In a simple linear regression setting, the probabilistic model equation allows for some deviation of the points about the regression line, making it a more practical model.

The value of the sum of squares for regression can never be smaller than 1.

In a simple linear regression , the least-squares line is = -3.75 + 1.25 , and the coefficient of determination is 0.81. The coefficient of correlation must be -0.90.

Given the least-squares regression line 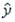= -2.48 + 1.63x, and a coefficient of determination of 0.81, what is the coefficient of correlation?

The value of the sum of squares for error can never be larger than the total sum of squares.

In a simple linear regression model, if the independent and dependent variables are negatively linearly related, then the standard error of the estimate will also be negative.

Regression analysis is a statistical method that seeks to establish an equation that allows the unknown value of one variable to be estimated from the known value of one or more other variables.

A regression line using 25 observations produced SSR = 118.68 and SSE = 56.32. What was the standard error of estimate?

A regression analysis between sales (in $1000) and advertising (in $) resulted in the following least-squares line: 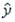= 60 + 5x. This implies that an increase of $1 in advertising is expected to result in an increase of $65 in sales.

In simple linear regression analysis, if the independent variable x and the dependent variable y are highly correlated, this means not only that they are linearly related, but also that a change in x will cause a change in y.

For the values of the coefficient of determination listed below, which one yields the greatest value of sum of squares for regression given that the total sum of squares is 200?