Exam 6: Discrete Random Variables
Exam 1: An Introduction to Business Statistics and Analytics98 Questions
Exam 2: Descriptive Statistics and Analytics: Tabular and Graphical Methods120 Questions
Exam 3: Descriptive Statistics and Analytics: Numerical Methods145 Questions
Exam 4: Probability and Probability Models150 Questions
Exam 5: Predictive Analytics I: Trees, K-Nearest Neighbors, Naive Bayes,101 Questions
Exam 6: Discrete Random Variables150 Questions
Exam 7: Continuous Random Variables150 Questions
Exam 8: Sampling Distributions111 Questions
Exam 9: Confidence Intervals149 Questions
Exam 10: Hypothesis Testing150 Questions
Exam 11: Statistical Inferences Based on Two Samples140 Questions
Exam 12: Experimental Design and Analysis of Variance132 Questions
Exam 13: Chi-Square Tests120 Questions
Exam 14: Simple Linear Regression Analysis147 Questions
Exam 15: Multiple Regression and Model Building85 Questions
Exam 16: Predictive Analytics Ii: Logistic Regression, Discriminate Analysis,101 Questions
Exam 17: Time Series Forecasting and Index Numbers161 Questions
Exam 18: Nonparametric Methods103 Questions
Exam 19: Decision Theory90 Questions
Select questions type
The mean and the variance of a Poisson random variable are equal.
(True/False)
4.9/5  (34)
(34)
The time (in seconds) it takes for an athlete to run 50 meters is an example of a continuous random variable.
(True/False)
4.7/5  (33)
(33)
Consider a Poisson distribution with an average of 3 customers per minute at the local grocery store. If X = the number of arrivals per minute, find the probability of 10 customers or fewer arriving within a minute.
(Multiple Choice)
4.8/5  (35)
(35)
If the probability of a success on a single trial is .2, what is the probability of obtaining 3 successes in 10 trials if the number of successes is binomial?
(Multiple Choice)
4.9/5  (28)
(28)
Using the following probability distribution table of the random variable x, what is the probability of x = 3? 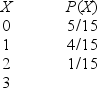
(Multiple Choice)
4.8/5  (35)
(35)
If in a hypergeometric distribution r = 300, N = 600, and n = 30, estimate the binomial probability of success.
(Multiple Choice)
4.9/5  (31)
(31)
The probability distribution of a random variable that is defined to be the number of successes obtained in a random sample selected without replacement from a finite population of N elements that contains r successes and N − r failures is
(Multiple Choice)
5.0/5  (35)
(35)
For a binomial process, the probability of success is 40 percent and the number of trials is 5. Find P(X ≤ 1).
(Multiple Choice)
4.7/5  (29)
(29)
The standard deviation of a discrete random variable measures the spread of the population of all possible values of x.
(True/False)
4.8/5  (30)
(30)
The hypergeometric probability distribution can be approximated by the Poisson distribution.
(True/False)
4.9/5  (42)
(42)
A fair die is rolled 10 times. What is the probability that an odd number (1, 3, or 5) will occur fewer than 3 times?
(Multiple Choice)
4.9/5  (33)
(33)
In a study conducted for the state Department of Education, 30 percent of the teachers who left teaching did so because they were laid off. Assume that we randomly select 10 teachers who have recently left their profession. Find the probability that exactly 4 of them were laid off.
(Multiple Choice)
4.7/5  (39)
(39)
One die is thrown. What is the expected value of the number of dots on the top face of the die?
(Multiple Choice)
4.9/5  (39)
(39)
Suppose you randomly select 3 DVDs from a production run of 10. Of the 10 DVDs, 9 are expected to last a minimum of 3 years. What is the probability that all 3 of your DVDs will last at least three years?
(Short Answer)
4.9/5  (30)
(30)
The number of ways to arrange x successes among n trials is equal to
(Multiple Choice)
4.7/5  (24)
(24)
A vaccine is 95 percent effective. What is the probability that it is not effective for more than 1 out of 20 individuals?
(Multiple Choice)
4.8/5  (32)
(32)
An important part of the customer service responsibilities of a cable company is the speed with which service troubles can be repaired. Historically, the data show that the likelihood is .75 that troubles in a residential service can be repaired on the same day. For the first five troubles reported on a given day, what is the probability that at least three troubles will be repaired on the same day?
(Multiple Choice)
4.8/5  (27)
(27)
The Securities and Exchange Commission has determined that the number of companies listed on the NYSE declaring bankruptcy is approximately a Poisson distribution with a mean of 2.6 per month. Find the probability that more than 1 bankruptcy occurs next month.
(Multiple Choice)
4.9/5  (31)
(31)
Suppose that x has a hypergeometric distribution with N = 10, r = 5, and n = 3. Calculate the mean of the distribution.
(Multiple Choice)
4.7/5  (34)
(34)
Showing 121 - 140 of 150
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)