Question 1

What is the probability of rolling a six with a fair die five times in a row?

Accepted Answer

A)  1/6 
B)  1/46,656 
C)  1/7,776 
D)  5/7,776 
A)  1/6 
B)  1/46,656 
C)  1/7,776 
D)  5/7,776

Question 2

Every current applicant for a position in the marketing department of Company A is given a 10-question test on interpretation of findings from statistical analyses. Individuals are rated on three levels based on their scores: Excellent (9-10 correct), Average (5-8 correct), and Poor (fewer than 5 correct). Historically, the probability of an individual scoring Excellent = .38, Average = .52, and Poor = .10. Also, the company knows that 90 percent of applicants who score Excellent are offered a position, 75 percent of applicants who score Average are offered a position, and 35 percent of the applicants who score Poor are offered a position. What is the probability that an individual who is offered a position has an Excellent score?

Accepted Answer

.446
P(E) = .38
P(A) = .52
P(P

Question 3

Bayes' Theorem is always based on two states of nature and three experimental outcomes.

Accepted Answer

A) True 
 B)False

Question 4

Employees of a local university have been classified according to gender and job type. 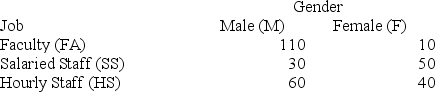  Are gender and type of job mutually exclusive?

Accepted Answer

A)  Yes 
B)  No 
A)  Yes 
B)  No

Question 5

It is very common for a television series to draw a large audience for special events or for cliff-hanging story lines. Suppose that on one of these occasions, the special show drew viewers from 38.2 percent of all US TV households. Suppose that three TV households are randomly selected. What is the probability that exactly one of the three households viewed the special show?

Accepted Answer

A)  0.146 
B)  0.084 
C)  0.438 
D)  0.382 
E)  0.056 
A)  0.146 
B)  0.084 
C)  0.438 
D)  0.382 
E)  0.056

Question 6

New car owners were asked to evaluate their experiences in buying a new car during the past 12 months. In the survey, the owners indicated they were most satisfied with their experiences at the following three dealers (in no particular order): Subaru, Honda, and Buick. When ranking the dealers, how many outcomes are possible?

Accepted Answer

A)  6 
B)  9 
C)  8 
D)  10 
E)  12 
A)  6 
B)  9 
C)  8 
D)  10 
E)  12

Question 7

New car owners were asked to evaluate their experiences in buying a new car during the past 12 months. In the survey, the owners indicated they were most satisfied with their experiences at the following three dealers (in no particular order): Subaru, Honda, and Buick. Assuming that each set of rankings is equally likely, what is the probability that owners ranked Subaru first and Honda second?

Accepted Answer

A)  1/3 
B)  1/6 
C)  1/2 
D)  5/6 
E)  6/6 
A)  1/3 
B)  1/6 
C)  1/2 
D)  5/6 
E)  6/6

Question 8

Suppose that A1, A2, and B are events where A1 and A2 are mutually exclusive events and P(A1) = .7, P(A2) = .3, P(B│A1) = .2, P(B│A2) = .4. Find P(A2│B).

Accepted Answer

A)  0.12 
B)  0.26 
C)  0.21 
D)  0.14 
E)  0.46 
A)  0.12 
B)  0.26 
C)  0.21 
D)  0.14 
E)  0.46

Question 9

At a college, 70 percent of the students are women, and 50 percent of the students receive a grade of C. 25 percent of the students are neither female nor C students. Construct a contingency table.

Accepted Answer

The answer of At a college, 70 percent of the...

Question 10

Probabilities must be assigned to sample space outcomes so that the probability assigned to each sample space outcome must be between ________, inclusive.

Accepted Answer

A)  0 and 100 
B)  −100 and 100 
C)  0 and 1 
D)  −1 and 1 
A)  0 and 100 
B)  −100 and 100 
C)  0 and 1 
D)  −1 and 1

Question 11

The ________ of event X consists of all sample space outcomes that do not correspond to the occurrence of event X.

Accepted Answer

A)  independence 
B)  complement 
C)  conditional probability 
D)  dependence 
A)  independence 
B)  complement 
C)  conditional probability 
D)  dependence

Question 12

The ________ of two events X and Y is another event that consists of the sample space outcomes belonging to either event X or event Y or both events X and Y.

Accepted Answer

A)  complement 
B)  union 
C)  intersection 
D)  conditional probability 
A)  complement 
B)  union 
C)  intersection 
D)  conditional probability

Question 13

Four employees who work as drive-through attendants at a local fast-food restaurant are being evaluated. As part of a quality improvement initiative and employee evaluation, these workers were observed over three days. One of the statistics collected was the proportion of time the employee forgot to include a napkin in the bag. Also recorded was the proportion of all dinners packed by each employee. Related information is given in the table.   You just purchased a dinner and found that there is no napkin in your bag. What is the probability that Jan prepared your order?

Accepted Answer

A)  0.200 
B)  0.004 
C)  0.018 
D)  0.075 
E)  0.100 
A)  0.200 
B)  0.004 
C)  0.018 
D)  0.075 
E)  0.100

Question 14

A probability model is a mathematic representation of a random phenomenon.

Accepted Answer

A) True 
 B)False

Question 15

Employees of a local university have been classified according to gender and job type. 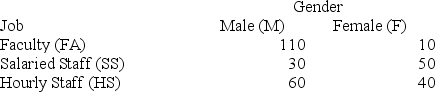  If an employee is selected at random, what is the probability that the employee is female or works as an hourly staff member?

Accepted Answer

A)  0.133 
B)  0.533 
C)  0.667 
D)  0.400 
E)  0.333 
A)  0.133 
B)  0.533 
C)  0.667 
D)  0.400 
E)  0.333

Question 16

Employees of a local university have been classified according to gender and job type. 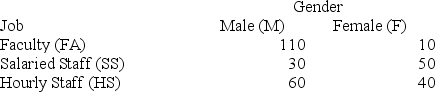  If an employee is selected at random, what is the probability that the employee is female, given that the employee is a salaried member of the staff?

Accepted Answer

A)  .167 
B)  .500 
C)  .625 
D)  267 
E)  .375 
A)  .167 
B)  .500 
C)  .625 
D)  267 
E)  .375

Question 17

If two events are independent, we can ________ their probabilities to determine the intersection probability.

Accepted Answer

A)  divide 
B)  add 
C)  multiply 
D)  subtract 
A)  divide 
B)  add 
C)  multiply 
D)  subtract

Question 18

At a college, 70 percent of the students are women, and 50 percent of the students receive a grade of C. 25 percent of the students are neither female nor C students. Use this contingency table. 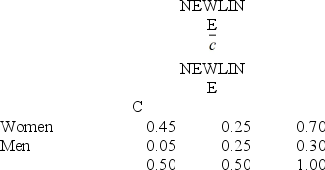  What is the probability that a student is female and a C student?

Accepted Answer

A)  .45 
B)  .50 
C)  .70 
D)  .25 
E)  .05 
A)  .45 
B)  .50 
C)  .70 
D)  .25 
E)  .05

Question 19

In a major midwestern university, 55 percent of all undergraduates are female, 25 percent of all undergraduates belong to a Greek organization (fraternity or sorority), and 40 percent of all males belong to a Greek organization. Are the events "female " and "belongs to a Greek organization" independent?

Accepted Answer

A)  Yes, independent. 
B)  No, not independent. 
A)  Yes, independent. 
B)  No, not independent.

Question 20

At a college, 70 percent of the students are women and 50 percent of the students receive a grade of C. 25 percent of the students are neither female nor C students. Use this contingency table.   If a student has received a grade of C, what is the probability that the student is female?

Accepted Answer

A)  0.45 
B)  0.90 
C)  0.70 
D)  0.64 
E)  0.50 
A)  0.45 
B)  0.90 
C)  0.70 
D)  0.64 
E)  0.50

What is the probability of rolling a six with a fair die five times in a row?

Bayes' Theorem is always based on two states of nature and three experimental outcomes.

Employees of a local university have been classified according to gender and job type. Are gender and type of job mutually exclusive?

Suppose that A₁, A₂, and B are events where A₁ and A₂ are mutually exclusive events and P(A₁) = .7, P(A₂) = .3, P(B│A₁) = .2, P(B│A₂) = .4. Find P(A₂│B).

At a college, 70 percent of the students are women, and 50 percent of the students receive a grade of C. 25 percent of the students are neither female nor C students. Construct a contingency table.

Probabilities must be assigned to sample space outcomes so that the probability assigned to each sample space outcome must be between ________, inclusive.

The ________ of event X consists of all sample space outcomes that do not correspond to the occurrence of event X.

The ________ of two events X and Y is another event that consists of the sample space outcomes belonging to either event X or event Y or both events X and Y.

A probability model is a mathematic representation of a random phenomenon.

Employees of a local university have been classified according to gender and job type. If an employee is selected at random, what is the probability that the employee is female or works as an hourly staff member?

Employees of a local university have been classified according to gender and job type. If an employee is selected at random, what is the probability that the employee is female, given that the employee is a salaried member of the staff?

If two events are independent, we can ________ their probabilities to determine the intersection probability.

At a college, 70 percent of the students are women, and 50 percent of the students receive a grade of C. 25 percent of the students are neither female nor C students. Use this contingency table. What is the probability that a student is female and a C student?

At a college, 70 percent of the students are women and 50 percent of the students receive a grade of C. 25 percent of the students are neither female nor C students. Use this contingency table. If a student has received a grade of C, what is the probability that the student is female?

An Introduction to Business Statistics and Analytics

Descriptive Statistics and Analytics: Tabular and Graphical Methods

Descriptive Statistics and Analytics: Numerical Methods

Predictive Analytics I: Trees, K-Nearest Neighbors, Naive Bayes,

Discrete Random Variables

Continuous Random Variables

Sampling Distributions

Confidence Intervals

Hypothesis Testing

Statistical Inferences Based on Two Samples

Experimental Design and Analysis of Variance

Chi-Square Tests

Simple Linear Regression Analysis

Multiple Regression and Model Building

Predictive Analytics Ii: Logistic Regression, Discriminate Analysis,

Time Series Forecasting and Index Numbers

Nonparametric Methods

Decision Theory

Filters

Exam 4: Probability and Probability Models

What is the probability of rolling a six with a fair die five times in a row?

Bayes' Theorem is always based on two states of nature and three experimental outcomes.

Employees of a local university have been classified according to gender and job type. Are gender and type of job mutually exclusive?

Suppose that A1, A2, and B are events where A1 and A2 are mutually exclusive events and P(A1) = .7, P(A2) = .3, P(B│A1) = .2, P(B│A2) = .4. Find P(A2│B).

At a college, 70 percent of the students are women, and 50 percent of the students receive a grade of C. 25 percent of the students are neither female nor C students. Construct a contingency table.

Probabilities must be assigned to sample space outcomes so that the probability assigned to each sample space outcome must be between ________, inclusive.

The ________ of event X consists of all sample space outcomes that do not correspond to the occurrence of event X.

The ________ of two events X and Y is another event that consists of the sample space outcomes belonging to either event X or event Y or both events X and Y.

A probability model is a mathematic representation of a random phenomenon.

Employees of a local university have been classified according to gender and job type. If an employee is selected at random, what is the probability that the employee is female or works as an hourly staff member?

Employees of a local university have been classified according to gender and job type. If an employee is selected at random, what is the probability that the employee is female, given that the employee is a salaried member of the staff?

If two events are independent, we can ________ their probabilities to determine the intersection probability.

At a college, 70 percent of the students are women, and 50 percent of the students receive a grade of C. 25 percent of the students are neither female nor C students. Use this contingency table. What is the probability that a student is female and a C student?

At a college, 70 percent of the students are women and 50 percent of the students receive a grade of C. 25 percent of the students are neither female nor C students. Use this contingency table. If a student has received a grade of C, what is the probability that the student is female?

An Introduction to Business Statistics and Analytics

Descriptive Statistics and Analytics: Tabular and Graphical Methods

Descriptive Statistics and Analytics: Numerical Methods

Predictive Analytics I: Trees, K-Nearest Neighbors, Naive Bayes,

Discrete Random Variables

Continuous Random Variables

Sampling Distributions

Confidence Intervals

Hypothesis Testing

Statistical Inferences Based on Two Samples

Experimental Design and Analysis of Variance

Chi-Square Tests

Simple Linear Regression Analysis

Multiple Regression and Model Building

Predictive Analytics Ii: Logistic Regression, Discriminate Analysis,

Time Series Forecasting and Index Numbers

Nonparametric Methods

Decision Theory

Filters

Suppose that A₁, A₂, and B are events where A₁ and A₂ are mutually exclusive events and P(A₁) = .7, P(A₂) = .3, P(B│A₁) = .2, P(B│A₂) = .4. Find P(A₂│B).