Exam 40: One-Dimensional Quantum Mechanics
Exam 1: Concepts of Motion52 Questions
Exam 2: Kinematics in One Dimension59 Questions
Exam 3: Vectors and Coordinate Systems33 Questions
Exam 4: Kinematics in Two Dimensions50 Questions
Exam 5: Force and Motion30 Questions
Exam 6: Dynamics I: Motion Along a Line46 Questions
Exam 7: Newtons Third Law43 Questions
Exam 8: Dynamics Ii: Motion in a Plane20 Questions
Exam 9: Work and Kinetic Energy66 Questions
Exam 10: Interactions and Potential Energy55 Questions
Exam 11: Impulse and Momentum43 Questions
Exam 12: Rotation of a Rigid Body116 Questions
Exam 13: Newtons Theory of Gravity50 Questions
Exam 14: Fluids and Elasticity72 Questions
Exam 15: Oscillations49 Questions
Exam 16: Traveling Waves51 Questions
Exam 17: Superposition51 Questions
Exam 18: A Macroscopic Description of Matter46 Questions
Exam 19: Work, Heat, and the First Law of Thermodynamics96 Questions
Exam 20: The Micromacro Connection41 Questions
Exam 21: Heat Engines and Refrigerators44 Questions
Exam 22: Electric Charges and Forces26 Questions
Exam 23: The Electric Field32 Questions
Exam 24: Gausss Law41 Questions
Exam 25: The Electric Potential40 Questions
Exam 26: Potential and Field57 Questions
Exam 27: Current and Resistance32 Questions
Exam 28: Fundamentals of Circuits68 Questions
Exam 29: The Magnetic Field83 Questions
Exam 30: Electromagnetic Induction66 Questions
Exam 31: Electromagnetic Fields and Waves52 Questions
Exam 32: Ac Circuits44 Questions
Exam 33: Wave Optics51 Questions
Exam 34: Ray Optics60 Questions
Exam 35: Optical Instruments52 Questions
Exam 36: Relativity49 Questions
Exam 37: The Foundations of Modern Physics7 Questions
Exam 38: Quantization45 Questions
Exam 39: Wave Functions and Uncertainty18 Questions
Exam 40: One-Dimensional Quantum Mechanics32 Questions
Exam 41: Atomic Physics41 Questions
Exam 42: Nuclear Physics65 Questions
Select questions type
An electron is bound in an infinite well (a box) of width 0.10 nm. If the electron is initially in the n = 8 state and falls to the n = 7 state, find the wavelength of the emitted photon.
(c = 3.00 × 108 m/s, h = 6.626 × 10-34 J ∙ s, mel = 9.11 × 10-31 kg)
Free
(Short Answer)
4.8/5  (43)
(43)
Correct Answer:
2.2 nm
The energy of a proton is 1.0 MeV below the top of a 6.8-fm-wide energy barrier. What is the probability that the proton will tunnel through the barrier? (1 eV = 1.60 × 10-19 J, mproton = 1.67 × 10-27 kg, h = 1.055 × 10-34 J ∙ s, h = 6.626 × 10-34 J ∙ s)
Free
(Multiple Choice)
4.8/5  (30)
(30)
Correct Answer:
A
An electron is in the ground state of an infinite well (a box) where its energy is 5.00 eV. In the next higher level, what would its energy be? (1 eV = 1.60 × 10-19 J)
Free
(Multiple Choice)
4.8/5  (38)
(38)
Correct Answer:
D
An electron is in an infinite square well (a box) that is 2.0 nm wide. The electron makes a transition from the  to the
to the 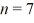 state, what is the wavelength of the emitted photon? (h = 6.626 × 10-34 J ∙ s, mel = 9.11 × 10-31 kg, 1 eV = 1.60 × 10-19)
state, what is the wavelength of the emitted photon? (h = 6.626 × 10-34 J ∙ s, mel = 9.11 × 10-31 kg, 1 eV = 1.60 × 10-19)
(Multiple Choice)
4.9/5  (33)
(33)
A 3.10-eV electron is incident on a 0.40-nm barrier that is 5.67 eV high. What is the probability that this electron will tunnel through the barrier? (1 eV = 1.60 × 10-19 J, mel = 9.11 × 10-31 kg, h = 1.055 × 10-34 J ∙ s, h = 6.626 × 10-34 J ∙ s)
(Multiple Choice)
4.8/5  (35)
(35)
You want to confine an electron in a box (an infinite well) so that its ground state energy is 5.0 × 10-18 J. What should be the length of the box? (h = 6.626 × 10-34 J ∙ s, mel = 9.11 × 10-31 kg)
(Multiple Choice)
4.8/5  (30)
(30)
The lowest energy level of a certain quantum harmonic oscillator is 5.00 eV. What is the energy of the next higher level?
(Multiple Choice)
4.9/5  (42)
(42)
A particle is confined to a one-dimensional box (an infinite well) on the x-axis between x = 0 and x = L. The potential height of the walls of the box is infinite. The normalized wave function of the particle, which is in the ground state, is given by ψ(x) = 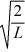 sin
sin 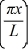 , with 0 ≤ x ≤ L. What is the probability of finding the particle between x = 0 and x = L/3?
, with 0 ≤ x ≤ L. What is the probability of finding the particle between x = 0 and x = L/3?
(Multiple Choice)
4.8/5  (38)
(38)
A particle is confined to a one-dimensional box (an infinite well) on the x-axis between x = 0 and x = L. The potential height of the walls of the box is infinite. The normalized wave function of the particle, which is in the ground state, is given by ψ(x) = 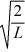 sin
sin 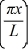 , with 0 ≤ x ≤ L. What is the maximum probability per unit length of finding the particle?
, with 0 ≤ x ≤ L. What is the maximum probability per unit length of finding the particle?
(Multiple Choice)
4.7/5  (30)
(30)
An electron is bound in an infinite square-well potential (a box) on the x-axis. The width of the well is L and the well extends from x = 0.00 nm to 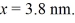 In its present state, the normalized wave function of the electron is given by: ψ(x) =
In its present state, the normalized wave function of the electron is given by: ψ(x) = 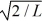 sin (2πx/L). What is the energy of the electron in this state? (h = 6.626 × 10-34 J ∙ s, mel = 9.11 × 10-31 kg,
1 eV = 1.60 × 10-19)
sin (2πx/L). What is the energy of the electron in this state? (h = 6.626 × 10-34 J ∙ s, mel = 9.11 × 10-31 kg,
1 eV = 1.60 × 10-19)
(Multiple Choice)
4.8/5  (33)
(33)
The wave function of an electron in a rigid box (infinite well) is shown in the figure. If the electron energy 98.0 eV, what is the energy of the electron's ground state? (mel = 9.11 × 10-31 kg) 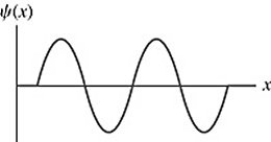
(Multiple Choice)
4.8/5  (34)
(34)
The lowest energy level of a particle confined to a one-dimensional region of space (a box, or infinite well) with fixed length L is E0. If an identical particle is confined to a similar region with fixed length L/6, what is the energy of the lowest energy level that the particles have in common? Express your answer in terms of E0.
(Short Answer)
4.9/5  (29)
(29)
An 80-eV electron impinges upon a potential barrier 100 eV high and 0.20 nm thick. What is the probability the electron will tunnel through the barrier? (1 eV = 1.60 × 10-19 J, mproton = 1.67 × 10-27 kg, h = 1.055 × 10-34 J ∙ s, h = 6.626 × 10-34 J ∙ s)
(Multiple Choice)
4.8/5  (38)
(38)
Calculate the ground state energy of a harmonic oscillator with a classical frequency of 3.68 × 1015 Hz. (1 eV = 1.60 × 10-19 J, h = 1.055 × 10-34 J ∙ s, h = 6.626 × 10-34 J ∙ s)
(Multiple Choice)
4.9/5  (35)
(35)
Find the wavelength of the photon emitted during the transition from the second EXCITED state to the ground state in a harmonic oscillator with a classical frequency of 3.72 × 1013 Hz. (c = 3.00 × 108 m/s, 1 eV = 1.60 × 10-19 J, h = 1.055 × 10-34 J ∙ s, h = 6.626 × 10-34 J ∙ s)
(Multiple Choice)
4.9/5  (38)
(38)
The energy of a particle in the second EXCITED state of a harmonic oscillator potential is 5.45 eV. What is the classical angular frequency of oscillation of this particle? (1 eV = 1.60 × 10-19 J, h = 1.055 × 10-34 J ∙ s, h = 6.626 × 10-34 J ∙ s)
(Multiple Choice)
4.8/5  (37)
(37)
An electron is confined in a one-dimensional box (an infinite well). Two adjacent allowed energies of the electron are 1.068 × 10-18 J and 1.352 × 10-18 J. What is the length of the box? (h = 6.626 × 10-34 J ∙ s, mel = 9.11 × 10-31 kg)
(Multiple Choice)
4.8/5  (25)
(25)
A lithium atom, mass 1.17 × 10-26 kg, vibrates with simple harmonic motion in a crystal lattice, where the effective force constant of the forces on the atom is k = 49.0 N/m.
(c = 3.00 × 108 m/s, h = 6.626 × 10-34 J ∙ s, h = 1.055 × 10-34 J ∙ s, 1 eV = 1.60 × 10-19 J)
(a) What is the ground state energy of this system, in eV?
(b) What is the wavelength of the photon that could excite this system from the ground state to the first excited state?
(Essay)
4.8/5  (33)
(33)
The smallest kinetic energy that an electron in a box (an infinite well) can have is zero.
(Multiple Choice)
4.8/5  (34)
(34)
An electron is in an infinite square well that is 2.6 nm wide. What is the smallest value of the state quantum number n for which the energy level exceeds 100 eV? (h = 6.626 × 10-34 J ∙ s, mel = 9.11 × 10-31 kg, 1 eV = 1.60 × 10-19)
(Multiple Choice)
4.9/5  (46)
(46)
Showing 1 - 20 of 32
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)