Exam 13: Inference About Comparing Two Populations

Undergraduates' Test Scores 35 undergraduate students who completed two years of college were asked to take a basic mathematics test.The mean and standard deviation of their scores were 75.1 and 12.8, respectively.In a random sample of 50 students who only completed high school, the mean and standard deviation of the test scores were 72.1 and 14.6, respectively. -{Undergraduates' Test Scores Narrative} Explain how to use the interval estimate to test the hypotheses.

Aptitude Test Scores Two random samples of 40 students were drawn independently from two populations of students.Assume their aptitude tests are normally distributed (total points = 100).The following statistics regarding their scores in an aptitude test were obtained: 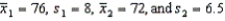. -{Aptitude Test Scores Narrative} Estimate with 95% confidence the difference between the two population means.

The statistical distribution used for testing the difference between two population variances is the

Two independent samples are drawn from two normal populations, where the population variances are assumed to be equal.The sampling distribution of the ratio of the two sample variances is:

Clothing Expenditures A marketing consultant was in the process of studying the perceptions of married couples concerning their monthly clothing expenditures.He believed that the husband's perception would be higher than the wife's.To judge his belief, he takes a random sample of ten married couples and asks each spouse to estimate the family clothing expenditure (in dollars) during the previous month.The data are shown below. 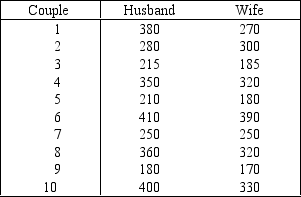-{Clothing Expenditures Narrative} Briefly describe what the interval estimate tells you.

A councilwoman regularly polls her constituency to gauge her level of support among voters.This month, 652 out of 1158 voters support her.Five months ago, 412 out of 982 voters supported her.With a 5% significance level, can she infer that support has increased by at least 10 percentage points?

Headache Medicine A researcher wants to see if/how men and women differ in their reaction to a headache medicine with respect to drowsiness.In testing the hypothese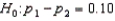s vs. 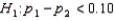, the following statistics were obtained: n₁ = 400, x₁ = 208, n₂ = 250, and x₂ = 115, where x₁ and x₂ represent the number of patients in the two samples (men vs.women) who reported to have drowsiness as a result of taking headache medicine. -{Headache Medicine Narrative} Estimate with 90% confidence the difference between the two population proportions.

In testing for a mean difference the null hypothesis is:

The pooled proportion estimate is used when:

Antioxidants A food processor wants to compare two antioxidants for their effects on retarding spoilage.Suppose 16 cuts of fresh meat are treated with antioxidant A and 16 are treated with antioxidant B, and the number of hours until spoilage begins is recorded for each of the 32 cuts of meat.The results are summarized in the table below 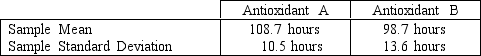-{Antioxidants Narrative} Determine the rejection region for testing the equality of the two population variances at $\alpha$ = .05.

The quantity is called the pooled variance estimate of the common variance of two unknown but equal population variances.It is the weighted average of the two sample variances, where the weights represent the:

In constructing a confidence interval estimate for the difference between two population proportions, we:

In testing for differences between the means of two independent populations the null hypothesis is:

TV Sex A survey of 1,500 Canadians reveals that 945 believe that there is too much sex on television.In a survey of 1,500 Americans, 810 believe that there is too much television sex. -{TV Sex Narrative} Estimate with 99% confidence the difference in the proportion of Canadians and Americans who believe that there is too much sex on television.

Additives A food processor wants to compare two additives for their effects on retarding spoilage.Suppose 16 cuts of fresh meat are treated with additive A and 16 are treated with additive B, and the number of hours until spoilage begins is recorded for each of the 32 cuts of meat.The results are summarized in the table below 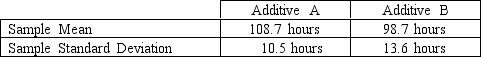-{Additives Narrative} Determine the rejection region at $\alpha$ = .05 and write the proper conclusion.

If the F-test statistic is large, that means the variance of Population 1 is ____________________ than/to the variance of Population 2.

If we are testing for the difference between the means of two independent populations with equal variances, samples of n₁ = 15 and n₂ = 15 are taken, then the number of degrees of freedom is equal to

The expected value of is .

The pooled proportion estimate is used when the proportion of successes from sample 1 equals the proportion of successes from sample 2.

In a matched pairs experiment the parameter of interest is the ____________________ of the population of ____________________.