Exam 10: Introduction to the Derivative
Exam 1: Functions and Applications159 Questions
Exam 2: Nonlinear Functions and Models92 Questions
Exam 3: The Mathematics of Finance137 Questions
Exam 4: Systems of Linear Equations and Matrices111 Questions
Exam 5: Matrix Algebra and Applications174 Questions
Exam 6: Linear Programming161 Questions
Exam 7: Sets and Counting154 Questions
Exam 8: Probability257 Questions
Exam 9: Random Variables and Statistics178 Questions
Exam 10: Introduction to the Derivative139 Questions
Exam 11: Techniques of Differentiation104 Questions
Exam 12: Applications of the Derivative88 Questions
Exam 13: The Integral66 Questions
Exam 14: Further Integration Techniques and Applications of the Integral118 Questions
Exam 15: Functions of Several Variables137 Questions
Exam 16: Trigonometric Models67 Questions
Select questions type
Estimate the slope of the tangent line to the graph of the following function at the point .
Please round the answer to the nearest whole number.
(Short Answer)
4.8/5  (30)
(30)
Determine what, if any, value to assign to to make f continuous at .
;
(Short Answer)
4.9/5  (27)
(27)
Use the graph to compute the following quantities.
and 
Please enter your answer as two numbers, separated by a comma.
Please enter your answer as two numbers, separated by a comma.
(Short Answer)
4.8/5  (40)
(40)
The graph of a function f is given. Determine whether f is continuous on its domain. 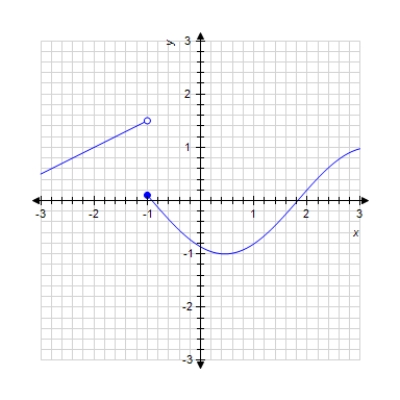
(Multiple Choice)
4.9/5  (33)
(33)
Use a graph to determine whether the function is continuous on its domain. If it is not continuous on its domain, list the points of discontinuity.
(Short Answer)
4.8/5  (33)
(33)
Calculate the average rate of change of the given function over the interval .
(Multiple Choice)
4.8/5  (31)
(31)
Determine what, if any, value to assign to to make f continuous at . ;
(Multiple Choice)
4.9/5  (38)
(38)
Estimate of the function .
?
Please round the answer to the nearest whole number.
(Essay)
4.8/5  (31)
(31)
Draw the graph of a function that is continuous on its domain but not continuous at any integer.
(Multiple Choice)
4.9/5  (29)
(29)
Showing 21 - 40 of 139
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)