Exam 19: Multiple Regression
Exam 1: What Is Statistics16 Questions
Exam 2: Types of Data, Data Collection and Sampling17 Questions
Exam 3: Graphical Descriptive Methods Nominal Data20 Questions
Exam 4: Graphical Descriptive Techniques Numerical Data64 Questions
Exam 5: Numerical Descriptive Measures150 Questions
Exam 6: Probability112 Questions
Exam 7: Random Variables and Discrete Probability Distributions55 Questions
Exam 8: Continuous Probability Distributions118 Questions
Exam 9: Statistical Inference: Introduction8 Questions
Exam 10: Sampling Distributions68 Questions
Exam 11: Estimation: Describing a Single Population132 Questions
Exam 12: Estimation: Comparing Two Populations23 Questions
Exam 13: Hypothesis Testing: Describing a Single Population130 Questions
Exam 14: Hypothesis Testing: Comparing Two Populations81 Questions
Exam 15: Inference About Population Variances47 Questions
Exam 16: Analysis of Variance125 Questions
Exam 17: Additional Tests for Nominal Data: Chi-Squared Tests116 Questions
Exam 18: Simple Linear Regression and Correlation219 Questions
Exam 19: Multiple Regression121 Questions
Exam 20: Model Building100 Questions
Exam 21: Nonparametric Techniques136 Questions
Exam 22: Statistical Inference: Conclusion106 Questions
Exam 23: Time-Series Analysis and Forecasting146 Questions
Exam 24: Index Numbers27 Questions
Exam 25: Decision Analysis51 Questions
Select questions type
For a multiple regression model, the following statistics are given: Total variation in y = SSY = 250, SSE = 50, k = 4, n = 20.
The coefficient of determination adjusted for degrees of freedom is:
(Multiple Choice)
4.9/5  (31)
(31)
For the multiple regression model 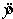 , if were to increase by 5 units, holding and constant, the value of would decrease by 50 units, on average.
, if were to increase by 5 units, holding and constant, the value of would decrease by 50 units, on average.
(True/False)
4.8/5  (32)
(32)
Multicollinearity is a situation in which the independent variables are highly correlated with the dependent variable.
(True/False)
5.0/5  (35)
(35)
In a multiple regression model, the probability distribution of the error variable is assumed to be:
(Multiple Choice)
4.7/5  (33)
(33)
In order to test the significance of a multiple regression model involving 4 independent variables and 25 observations, the number of degrees of freedom for the numerator and denominator, respectively, for the critical value of F are 4 and 20, respectively.
(True/False)
4.8/5  (39)
(39)
An actuary wanted to develop a model to predict how long individuals will live. After consulting a number of physicians, she collected the age at death (y), the average number of hours of exercise per week ( ), the cholesterol level ( ), and the number of points by which the individual's blood pressure exceeded the recommended value ( ). A random sample of 40 individuals was selected. The computer output of the multiple regression model is shown below:
THE REGRESSION EQUATION IS Predictor Coef StDev Constant 55.8 11.8 4.729 1.79 0.44 4.068 -0.021 0.011 -1.909 -0.016 0.014 -1.143 S = 9.47 R-Sq = 22.5%. ANALYSIS OF VARIANCE Source of Variation df SS MS F Regression 3 936 312 3.477 Error 36 3230 89.722 Total 39 4166 What is the coefficient of determination? What does this statistic tell you?
(Essay)
5.0/5  (36)
(36)
In multiple regression, the descriptor 'multiple' refers to more than one independent variable.
(True/False)
4.8/5  (30)
(30)
Pop-up coffee vendors have been popular in the city of Adelaide in 2013. A vendor is interested in knowing how temperature (in degrees Celsius) and number of different pastries and biscuits offered to customers impacts daily hot coffee sales revenue (in $00's).
A random sample of 6 days was taken, with the daily hot coffee sales revenue and the corresponding temperature and number of different pastries and biscuits offered on that day, noted.
Excel output for a multiple linear regression is given below: Coffee sales revenue Temperature Pastries/biscuits 6.5 25 7 10 17 13 5.5 30 5 4.5 35 6 3.5 40 3 28 9 15 SUMMARV OUTPUT Regression Stotistics Multiple R 0.87 R Square 0.75 Adjusted R Square 0.59 Standard Error 5.95 Otservations 6.00 ANOVA Significonce df SS MS F F Regression 2.00 322.14 161.07 4.55 0.12 Residual 3.00 106.20 35.40 Total 5.00 428.33 Coefficients Standard Error tStot Pvolue Lower 95\% Upper 95\% Intercept 18.68 37.8 0.49 0.66 -101.88 139.24 Temperature -0.50 0.83 -0.60 0.59 -3.15 2.15 Patries/bisouits 0.49 2.02 0.24 0.82 -5.94 6.92 Comment on the difference between the coefficient of determination and the Adjusted coefficient of determination.
(Essay)
4.9/5  (33)
(33)
In a multiple regression model, the following statistics are given: SSE = 100, , k = 5, n = 15.
The multiple coefficient of determination adjusted for degrees of freedom is:
(Multiple Choice)
4.9/5  (43)
(43)
The adjusted multiple coefficient of determination is adjusted for the number of independent variables and the sample size.
(True/False)
4.8/5  (45)
(45)
In multiple regression, when the response surface (the graphical depiction of the regression equation) hits every single point, the sum of squares for error SSE = 0, the standard error of estimate = 0, and the coefficient of determination = 1.
(True/False)
4.8/5  (24)
(24)
In a multiple regression analysis, when there is no linear relationship between each of the independent variables and the dependent variable, then:
(Multiple Choice)
4.8/5  (32)
(32)
A multiple regression model has the form ŷ = b0 + b1x1 + b2x2. Which of the following best describes b2?
(Multiple Choice)
4.8/5  (36)
(36)
In regression analysis, we judge the magnitude of the standard error of estimate relative to the values of the dependent variable, and particularly to the mean of y.
(True/False)
4.8/5  (36)
(36)
An actuary wanted to develop a model to predict how long individuals will live. After consulting a number of physicians, she collected the age at death (y), the average number of hours of exercise per week ( ), the cholesterol level ( ), and the number of points by which the individual's blood pressure exceeded the recommended value ( ). A random sample of 40 individuals was selected. The computer output of the multiple regression model is shown below:
THE REGRESSION EQUATION IS Predictor Coef StDev Constant 55.8 11.8 4.729 1.79 0.44 4.068 -0.021 0.011 -1.909 -0.016 0.014 -1.143 S = 9.47 R-Sq = 22.5%. ANALYSIS OF VARIANCE Source of Variation df SS MS F Regression 3 936 312 3.477 Error 36 3230 89.722 Total 39 4166 Is there enough evidence at the 10% significance level to infer that the model is useful in predicting length of life?
(Essay)
4.7/5  (34)
(34)
In order to test the validity of a multiple regression model involving 4 independent variables and 35 observations, the numbers of degrees of freedom for the numerator and denominator, respectively, for the critical value of F are:
(Multiple Choice)
4.9/5  (35)
(35)
An actuary wanted to develop a model to predict how long individuals will live. After consulting a number of physicians, she collected the age at death (y), the average number of hours of exercise per week ( ), the cholesterol level ( ), and the number of points by which the individual's blood pressure exceeded the recommended value ( ). A random sample of 40 individuals was selected. The computer output of the multiple regression model is shown below:
THE REGRESSION EQUATION IS Predictor Coef StDev Constant 55.8 11.8 4.729 1.79 0.44 4.068 -0.021 0.011 -1.909 -0.016 0.014 -1.143 S = 9.47 R-Sq = 22.5%. ANALYSIS OF VARIANCE Source of Variation df SS MS F Regression 3 936 312 3.477 Error 36 3230 89.722 Total 39 4166 Is there enough evidence at the 5% significance level to infer that the number of points by which the individual's blood pressure exceeded the recommended value and the age at death are negatively linearly related?
(Essay)
4.8/5  (43)
(43)
A statistician wanted to determine whether the demographic variables of age, education and income influence the number of hours of television watched per week. A random sample of 25 adults was selected to estimate the multiple regression model .
Where:
y = number of hours of television watched last week. = age. = number of years of education. = income (in $1000s).
The computer output is shown below.
THE REGRESSION EQUATION IS Predictor Coef StDev Constant 22.3 10.7 2.084 0.41 0.19 2.158 -0.29 0.13 -2.231 -0.12 0.03 -4.00 S = 4.51 R-Sq = 34.8%. ANALYSIS OF VARIANCE Source of Variation df SS MS F Regression 3 227 75.667 3.730 Error 21 426 20.286 Total 24 653 Interpret the coefficient .
(Essay)
4.9/5  (37)
(37)
A multiple regression equation includes 5 independent variables, and the coefficient of determination is 0.64. The percentage of the variation in y that is explained by the regression equation is:
(Multiple Choice)
4.8/5  (30)
(30)
Showing 21 - 40 of 121
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)