Exam 14: Multicriteria Decisions
Exam 1: Introduction63 Questions
Exam 2: An Introduction to Linear Programming66 Questions
Exam 3: Linear Programming: Sensitivity Analysis and Interpretation of Solution56 Questions
Exam 4: Linear Programming Applications in Marketing, Finance, and Operations Management63 Questions
Exam 5: Advanced Linear Programming Applications46 Questions
Exam 6: Distribution and Network Models70 Questions
Exam 7: Integer Linear Programming61 Questions
Exam 8: Nonlinear Optimization Models51 Questions
Exam 9: Project Scheduling: Pertcpm59 Questions
Exam 10: Inventory Models65 Questions
Exam 11: Waiting Line Models68 Questions
Exam 12: Simulation62 Questions
Exam 13: Decision Analysis97 Questions
Exam 14: Multicriteria Decisions50 Questions
Exam 15: Time Series Analysis and Forecasting63 Questions
Exam 16: Markov Processes49 Questions
Exam 17: Linear Programming: Simplex Method51 Questions
Exam 18: Simplex-Based Sensitivity Analysis and Duality35 Questions
Exam 19: Solution Procedures for Transportation and Assignment Problems44 Questions
Exam 20: Minimal Spanning Tree19 Questions
Exam 21: Dynamic Programming38 Questions
Select questions type
One limitation of a scoring model is that it uses arbitrary weights that do not necessarily reflect the preferences of the individual decision maker.
Free
(True/False)
4.8/5  (31)
(31)
Correct Answer:
False
The goal programming problem below was solved with the Management Scientist.
Min
P1(d1−) + P2(d2+) + P3(d3−)
s.t.
72x1 + 38x2 + 23x3 ≤ 20,000
.72x1 − .76x2 − .23x3 + d1− − d1+ = 0
x3 + d2− − d2+ = 150
38x2 + d3− − d3+ = 2000
x1, x2, x3, d1−, d 1+, d2−, d2+, d3−, d3+ ≥ 0
Partial output from three successive linear programming problems is given. For each problem, give the original objective function expression and its value, and list any constraints needed beyond those that were in the original problem. 


Free
(Essay)
4.8/5  (28)
(28)
Correct Answer:
a.Min d1minus, Z = 0
B.Min d2plus, Z = 0, the constraint d1minus = 0 was includeD.C.Min d3minus, Z = 0, the constraint d2plus = 0 was included.
An ATM is to be located in a campus union building so that it minimizes the distance from the food court, the gift shop, and the theater. They are located at coordinates (2,2), (0,6) and (8,0). Develop a goal programming model to use to locate the best place for the ATM.
Free
(Essay)
4.9/5  (36)
(36)
Correct Answer:
Let
x1 = the first coordinate of the ATM
x2 = the second coordinate of the ATM
Min
Σ (di+ + di− )s.t.
x1 + d1− − d1+ = 2
x1 + d2− − d2+ = 2
x1 + d3− − d3+ = 0
x2 + d4− − d4+ = 6
x2 + d5− − d5+ = 8
x2 + d6− − d6+ = 0
Explain the structure of a hierarchy diagram used in the analytic hierarchy process as a graphical representation of
the problem.
(Essay)
4.9/5  (40)
(40)
AHP allows a decision maker to express personal preferences about the various aspects of a multicriteria problem.
(True/False)
4.8/5  (31)
(31)
Goal equations consist of a function that defines goal achievement and deviation variables that measure the distance from the target.
(True/False)
4.9/5  (34)
(34)
The steps of the scoring model include all of the following EXCEPT:
(Multiple Choice)
4.7/5  (35)
(35)
Like many high school seniors, Anne has several universities to consider when making her final college choice. To assist in her decision, she has decided to use AHP to develop a ranking for school R, school P, and school M. The schools will be evaluated on five criteria, and Anne's pair-wise comparison matrix for the criteria is shown below. 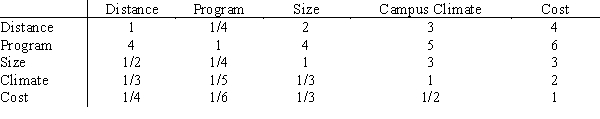
The universities' pair-wise comparisons on the criteria are shown below.
The universities' pair-wise comparisons on the criteria are shown below. 




a.What is the overall ranking of the five criteria?
b.What is the overall ranking of the three universities?
a.What is the overall ranking of the five criteria?
b.What is the overall ranking of the three universities?
(Essay)
4.7/5  (37)
(37)
The goal programming approach can be used when an analyst is confronted with an infeasible solution to an ordinary linear program.
(True/False)
4.7/5  (34)
(34)
The priority matrix shows the priority for each item on each criterion.
(True/False)
4.9/5  (30)
(30)
The constraint 5x1 + 3x2 ≤ 150 is modified to become a goal equation, and priority one is to avoid overutilization. Which of the following is appropriate?
(Multiple Choice)
4.9/5  (37)
(37)
The Lofton Company has developed the following linear programming problem
Max
x1 + x2
s.t.
2x1 + x2 ≤ 10
2x1 + 3x2 ≤ 24
3x1 + 4x2 ≥ 36
but finds it is infeasible. In revision, Lofton drops the original objective and establishes the three goals
Goal 1:
Don't exceed 10 in constraint 1.
Goal 2:
Don't fall short of 36 in constraint 3.
Goal 3:
Don't exceed 24 in constraint 2.
Give the goal programming model and solve it graphically.
(Essay)
4.7/5  (35)
(35)
Why are multicriteria problems of special interest to quantitative analysts?
(Short Answer)
4.8/5  (34)
(34)
Calculating the priority of each criterion in terms of its contribution to the overall goal is known as developing the hierarchy.
(True/False)
4.8/5  (37)
(37)
A problem involving only one priority level is not considered a goal programming problem.
(True/False)
4.8/5  (30)
(30)
The goal programming problem with the objective function min P1(d1+) +P2(d2−) is initially solved by the computer and the objective function value is 0. What constraint should be added for the second problem?
(Multiple Choice)
4.9/5  (25)
(25)
Should the decision maker always accept the alternatives with the highest AHP rating? Explain.
(Short Answer)
4.9/5  (33)
(33)
Showing 1 - 20 of 50
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)