Exam 7: Arithmetic Sequence: Common Difference and First n Terms
Exam 1: Review of Basic Concepts637 Questions
Exam 2: Equations and Inequalities498 Questions
Exam 3: Graphs and Functions531 Questions
Exam 4: Polynomials and Rational Functions517 Questions
Exam 5: Inverse, Exponential, and Logarithmic Functions472 Questions
Exam 6: Systems and Matrices505 Questions
Exam 7: Arithmetic Sequence: Common Difference and First n Terms570 Questions
Select questions type
Use mathematical induction to prove that the statement is true for every positive integer n.
-
(Essay)
4.9/5  (30)
(30)
Decide whether the given sequence is finite or infinite.
-
(Multiple Choice)
4.8/5  (31)
(31)
Use a graphing calculator to evaluate the sum. Round to the nearest thousandth.
-
(Multiple Choice)
4.8/5  (29)
(29)
An ordinary die is tossed. What are the odds in favor of the die showing a number less than 3?
(Multiple Choice)
4.9/5  (38)
(38)
Match the equation of the ellipse with the appropriate description.
-
(Multiple Choice)
4.8/5  (31)
(31)
Use the summation properties to evaluate the series. The following rules may be needed:
-
(Multiple Choice)
4.8/5  (35)
(35)
Determine the two equations necessary to graph the horizontal parabola using a graphing calculator.
-
(Multiple Choice)
5.0/5  (27)
(27)
Use the summation properties to evaluate the series. The following rules may be needed:
-
(Multiple Choice)
4.8/5  (32)
(32)
Provide an appropriate response.
-Explain why the vertex of a parabola must lie on its axis.
(Essay)
4.7/5  (44)
(44)
Identify the equation as a parabola, circle, ellipse, or hyperbola.
-
(Multiple Choice)
5.0/5  (38)
(38)
What are the odds in favor of spinning an A on this spinner? 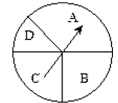
(Multiple Choice)
4.9/5  (29)
(29)
Showing 481 - 500 of 570
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)