Exam 7: Arithmetic Sequence: Common Difference and First n Terms
Exam 1: Review of Basic Concepts637 Questions
Exam 2: Equations and Inequalities498 Questions
Exam 3: Graphs and Functions531 Questions
Exam 4: Polynomials and Rational Functions517 Questions
Exam 5: Inverse, Exponential, and Logarithmic Functions472 Questions
Exam 6: Systems and Matrices505 Questions
Exam 7: Arithmetic Sequence: Common Difference and First n Terms570 Questions
Select questions type
Find for the following arithmetic sequence.
-2, 6, 10, 14, 18, . . .
(Multiple Choice)
4.9/5  (32)
(32)
Find the first term and the common difference for the arithmetic sequence. Round approximations to the nearest
hundredth.
-
(Multiple Choice)
4.8/5  (36)
(36)
Find the eccentricity of the conic section shown in the graph.
-

(Multiple Choice)
4.8/5  (41)
(41)
Three noncollinear points determine a triangle. How many triangles can be formed with 8 noncollinear points?
(Multiple Choice)
4.9/5  (29)
(29)
The series of sketches below starts with an equilateral triangle having sides of length 1 (one). In the following
steps, equilateral triangles are constructed by joining the midpoints of the sides of the preceding triangle.
Develop a formula for the area of the nth new triangle. Use math induction to prove your answer. 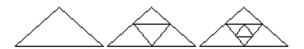
(Essay)
5.0/5  (33)
(33)
It can be shown that a hyperbola with center at the origin, foci at and , and equation has equation . Use this result to find an equation of a hyperbola with center at the origin, foci at and , and absolute value of the distances from any point of the hyperbola to the two foci equal to 8 .
(Multiple Choice)
4.8/5  (35)
(35)
Evaluate the sum using the given information.
- =-5,=4,=5,=3 , and \Deltax=0.1;f(x)= f \Deltax
(Multiple Choice)
4.8/5  (33)
(33)
There are some fundamental differences between graphing a hyperbola centered at the origin and graphing an
ellipse centered at the origin. What are these differences?
(Essay)
5.0/5  (39)
(39)
Evaluate the sum. Round to two decimal places, if necessary.
-
(Multiple Choice)
4.9/5  (23)
(23)
Find the sum of the first n terms of the following arithmetic sequence.
-
(Multiple Choice)
4.9/5  (44)
(44)
Use the summation properties to evaluate the series. The following rules may be needed:
-
(Multiple Choice)
5.0/5  (27)
(27)
Find the first term and the common difference for the arithmetic sequence. Round approximations to the nearest
hundredth.
-
(Multiple Choice)
4.9/5  (40)
(40)
A railroad bridge is built over a V-shaped canyon. If the supports are 8 feet long at each end, the center support is 560 feet long, and the supports increase in length by 4 feet with each support (from 8 feet to 560 feet),
What is the total length of all the supports?
(Multiple Choice)
4.8/5  (30)
(30)
Showing 81 - 100 of 570
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)