Exam 3: Exponential, Logistic, and Logarithmic Functions
Exam 1: Functions and Graphs362 Questions
Exam 2: Polynomial, Power, and Rational Functions494 Questions
Exam 3: Exponential, Logistic, and Logarithmic Functions350 Questions
Exam 4: Trigonometric Functions522 Questions
Exam 5: Analytic Trigonometry313 Questions
Exam 6: Applications of Trigonometry333 Questions
Exam 7: Systems and Matrices354 Questions
Exam 8: Analytic Geometry in Two and Three Dimensions167 Questions
Exam 9: Discrete Mathematics154 Questions
Exam 10: Statistics and Probability147 Questions
Exam 11: An Introduction to Calculus: Limits, Derivatives, and Integrals167 Questions
Exam 12: Prerequisites382 Questions
Select questions type
Use a calculator to find an approximate solution to the equation.
-
(Multiple Choice)
4.9/5  (32)
(32)
Find the amount accumulated after investing a principal P for t years at an interest rate r.
-P = $480, t = 6, r = 10%, compounded quarterly (k = 4)
(Multiple Choice)
4.7/5  (38)
(38)
Compute the exact value of the function for the given x-value without using a calculator.
-
(Multiple Choice)
4.8/5  (35)
(35)
Graph the function and analyze it for domain, range, continuity, increasing or decreasing behavior, symmetry,
boundedness, extrema, asymptotes, and end behavior.
-f(x) = ln (3 - x)
(Essay)
4.8/5  (37)
(37)
Graph the function. Describe its position relative to the graph of the indicated basic function.
-

(Multiple Choice)
4.7/5  (40)
(40)
Decide if the function is an exponential function. If it is, state the initial value and the base.
-
(Multiple Choice)
4.8/5  (31)
(31)
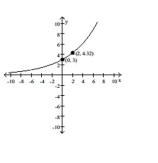
Find the logistic function that satisfies the given conditions.
-
(Multiple Choice)
4.9/5  (37)
(37)
Decide if the function is an exponential function. If it is, state the initial value and the base.
-
(Multiple Choice)
4.8/5  (34)
(34)
Solve the problem.
-The relationship between intensity I of light (in lumens) at a depth of feet in Lake is given by . What is the intensity at a depth of 50 feet?(Round to the nearest ten-thousandth.)
(Multiple Choice)
4.9/5  (38)
(38)
Describe how to transform the graph of g(x) = ln x into the graph of the given function. Graph the function.
-

(Multiple Choice)
4.8/5  (38)
(38)
Showing 321 - 340 of 350
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)