Exam 1: Functions and Models
Exam 1: Functions and Models112 Questions
Exam 2: Limits and Derivatives76 Questions
Exam 3: Differentiation Rules75 Questions
Exam 4: Applications of Differentiation77 Questions
Exam 5: Integrals60 Questions
Exam 6: Applications of Integration78 Questions
Exam 7: Techniques of Integration79 Questions
Exam 8: Further Applications of Integration59 Questions
Exam 9: Differential Equations60 Questions
Exam 10: Parametric Equations and Polar Coordinates60 Questions
Exam 11: Infinite Sequences and Series60 Questions
Exam 12: Vectors and the Geometry of Space54 Questions
Exam 13: Vector Functions58 Questions
Exam 14: Partial Derivatives39 Questions
Exam 15: Multiple Integrals60 Questions
Exam 16: Vector Calculus59 Questions
Exam 17: Second-Order Differential Equations60 Questions
Select questions type
Determine whether is even, odd, or neither.
Free
(Short Answer)
5.0/5  (34)
(34)
Correct Answer:
even
Graph the function by hand, not by plotting points, but by starting with the graph of one of the
standard functions and then applying the appropriate transformations.
Free
(Essay)
4.9/5  (37)
(37)
Correct Answer:
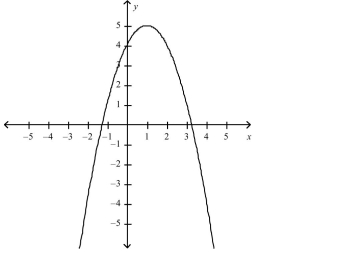
Consider the following function.
Determine the values of for which exists.
(Short Answer)
4.9/5  (43)
(43)
Use the table to evaluate the expression .
x 1 2 3 4 5 6 f(x) 3 2 1 0 1 2 g(x) 6 5 2 3 4 6
(Multiple Choice)
4.9/5  (29)
(29)
The graph of the function has been stretched horizontally by a factor of 2 . Find the function for the transformed graph.
(Multiple Choice)
4.8/5  (39)
(39)
You are given and a tolerance . Find a number such that whenever .
(Short Answer)
4.8/5  (35)
(35)
Estimate the value of the following limit by graphing the function . Round your answer correct to two decimal places.
(Short Answer)
4.8/5  (37)
(37)
Showing 1 - 20 of 112
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)