Exam 6: The Normal Distributions and Other Continuous Distributions

SCENARIO 6-3 Suppose the time interval between two consecutive defective light bulbs from a production line has a uniform distribution over an interval from 0 to 90 minutes. -Referring to Scenario 6-3, what is the probability that the time interval between two consecutive defective light bulbs will be less than 10 minutes?

Suppose Z has a standard normal distribution with a mean of 0 and standard deviation of 1. The probability that Z is more than 0.77 is __________.

SCENARIO 6-3 Suppose the time interval between two consecutive defective light bulbs from a production line has a uniform distribution over an interval from 0 to 90 minutes. -Referring to Scenario 6-3, what is the mean of the time interval?

SCENARIO 6-7 Ball bearings are manufactured with a mean diameter of 6 millimeters (mm).Because of the inherent manufacturing process variability, the lots of bearings are approximately normally distributed with a standard deviation of 0.03 mm. -Using Scenario 6-7, what proportion of ball bearings has a diameter of greater than 6 mm?

Suppose Z has a standard normal distribution with a mean of 0 and standard deviation of 1. The probability that Z is between -0.88 and 2.29 is __________.

The owner of a fish market determined that the mean weight for a catfish is 3.2 pounds.He also knew that the probability of a randomly selected catfish that would weigh more than 3.8 pounds is 20% and the probability that a randomly selected catfish that would weigh less than 2.8 pounds is 30%.The middle 40% of the catfish will weigh between ______ pounds and ______ pounds.

SCENARIO 6-4 The interval between consecutive hits at a web site is assumed to follow an exponential distribution with a mean of 40 hits per minute. -Referring to Scenario 6-4, what is the probability that the next hit at the web site will occur within 10 seconds after just being hit by a visitor?

SCENARIO 6-5 A company producing orange juice buys all its oranges from a large orange orchard.The amount of juice that can be squeezed from each of these oranges is approximately normally distributed with a mean of 4.7 ounces and some unknown standard deviation.The company's production manager knows that the probability is 30.85% that a randomly selected orange will contain less than 4.5 ounces of juice.Also, the probability is 10.56% that a randomly selected orange will contain more than 5.2 ounces of juice.Answer the following questions without the help of a calculator, statistical software or statistical table. -Referring to Scenario 6-5, what is the probability that a randomly selected orange will contain no more than 4.2 ounces of juices?

Suppose Z has a standard normal distribution with a mean of 0 and standard deviation of 1. So, 96% of the possible Z values are between __________ and __________ (symmetrically distributed about the mean).

You were told that the amount of time elapsed between consecutive trades on a foreign stock exchange market followed a normal distribution with a mean of 15 seconds.You were also told that the probability that the time elapsed between two consecutive trades to fall between 16 to 17 seconds was 13%.The probability that the time elapsed between two consecutive trades would fall below 13 seconds was 7%.The probability is 80% that the time elapsed will be longer than how many seconds?

The probability that a standard normal variable, Z, falls between -2.00 and - 0.44 is 0.6472.

The interval between patients arriving at an outpatient clinic follows an exponential distribution at a rate of 15 patients per hour.What is the probability that a randomly chosen arrival to be less than 15 minutes?

SCENARIO 6-1 The number of column inches of classified advertisements appearing on Mondays in a certain daily newspaper is normally distributed with population mean of 320 and population standard deviation of 20 inches. -Referring to Scenario 6-1, for a randomly chosen Monday, what is the probability there will be less than 340 column inches of classified advertisement?

The true length of boards cut at a mill with a listed length of 10 feet is normally distributed with a mean of 123 inches and a standard deviation of 1 inch.What proportion of the boards will be over 125 inches in length?

The amount of time between successive TV watching by first graders follows an exponential distribution with a mean of 10 hours.The probability that a given first grader spends less than 20 hours between successive TV watching is ______.

The amount of tea leaves in a can from a production line is normally distributed with 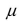= 110 grams and 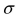= 25 grams.What is the probability that a randomly selected can will contain at least 100 grams of tea leaves?

The interval between patients arriving at an outpatient clinic follows an exponential distribution at a rate of 15 patients per hour.What is the probability that a randomly chosen arrival to be between 5 minutes and 15 minutes?

The amount of time necessary for assembly line workers to complete a product is a normal variable with a mean of 15 minutes and a standard deviation of 2 minutes.The probability is __________ that a product is assembled in less than 20 minutes.

The amount of tea leaves in a can from a production line is normally distributed with 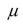= 110 grams and 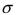= 25 grams.What is the probability that a randomly selected can will contain between 82 and 100 grams of tea leaves?

SCENARIO 6-5 A company producing orange juice buys all its oranges from a large orange orchard.The amount of juice that can be squeezed from each of these oranges is approximately normally distributed with a mean of 4.7 ounces and some unknown standard deviation.The company's production manager knows that the probability is 30.85% that a randomly selected orange will contain less than 4.5 ounces of juice.Also, the probability is 10.56% that a randomly selected orange will contain more than 5.2 ounces of juice.Answer the following questions without the help of a calculator, statistical software or statistical table. -Referring to Scenario 6-5, what is the probability that a randomly selected orange will contain no more than 4.9 ounces of juices?