Exam 9: Understanding Sampling Distributions: Statistics As Random Variables

Use the following information for questions: Contradicting some previous research, a new study finds that frequent use of painkillers does not substantially increase a healthy man's risk of developing hypertension. -What would have been the null value in this scenario for the difference in proportions of men who developed hypertension (frequently use painkiller - not frequently use painkiller)?

Use the following information for questions: Suppose that the weight of apples eaten by individual Americans each year can be described by a normal distribution with mean $\mu$ = 15 pounds and standard deviation $\sigma$ = 5 pounds per year. -For a random sample of n = 16 people, what is the standard deviation of the sampling distribution of the sample mean $\overline{x}$ ?

Which one of the following statements is false?

Use the following information for questions: High school students can be categorized into two groups by the amount of activities they are involved in. Let group 1 consist of all high school students who are very involved in sports and other activities and group 2 consist of all high school students who aren't. The distributions of GPAs in both groups are approximately normal. The mean and standard deviation for group 1 are 2.9 and 0.4, respectively. The mean and standard deviation for group 2 are 2.7 and 0.5, respectively. Independent random samples of 50 high school students are to be selected from both groups (for a total of 100 students). -What is the probability that the average GPA in the sample of students who are not so involved is higher than the average GPA in the sample of students who are very involved?

Use the following information for questions: Many adults complain they do not get enough sleep at night. What happens when these adults have children? Independent random samples of 150 adults who do not have children in the house and 130 adults who do have children in the house are selected. The average amount of sleep the adults without children had was 7.1 hours with a standard deviation of 0.7 hours. The average amount of sleep the adults with children had was 6.5 hours with a standard deviation of 0.8 hours. -What do we call the estimate in question 124?

In a pond with a large population of turtles, the proportion of turtles that are snapping turtles is 20%. In a random sample of n = 80 turtles from this pond, 20 are snapping turtles. What is the z-score associated with the observed sample proportion of snapping turtles?

Use the following information for questions: In a random sample of 250 full time students, 80% preferred to have three one-hour lectures per week rather than two 90-minute lectures. In a random sample of 500 part-time students, 50% said they preferred the three one-hour lectures schedule. The difference between the two sample proportions (full-time - part-time) is to be calculated. -What is the standard error for the difference between the two sample proportions?

For which of the following situations would the Rule for Sample Proportions not apply?

Use the following information for questions: Suppose that the weight of apples eaten by individual Americans each year can be described by a normal distribution with mean $\mu$ = 15 pounds and standard deviation $\sigma$ = 5 pounds per year. -For a random sample of n = 9 people, what is the z-score for a sample mean $\overline{x}$ = 10 pounds?

Which of the following describes the relationship between the Central Limit Theorem and the Rule for Sample Proportions?

Use the following information for questions: Based on the current rate of savings, the average American household will live on 59 percent of pre-retirement income once they retire, a recent report says. The report also says that Americans are behind in funding their retirement years. The report recommended saving enough to be able to live on 85 percent of income in retirement. However, the typical household has put aside only $18,750 for retirement. Suppose we can assume that the true average amount a typical household has put aside for retirement is $18,750. Also assume that these amounts vary with a standard deviation of $12,500. The distribution of these amounts is known to be right-skewed. -Suppose one household is to be randomly selected. Is the probability that this household has set aside more than $30,000 for retirement equal to 0.1841?

Use the following information for questions: Scores on an English spelling test are determined for a sample of n = 25 fifth graders both before and after they learn some new vocabulary "tricks of the trade". In the population of all differences in scores it is known that students improve with an average of 3 points, with a standard deviation of 4 points, and that the distribution of the differences is approximately normal. -What is the z-score if the mean difference in the sample was $\overline{d}$ = 2?

Use the following information for questions: In each situation, indicate whether the value given in bold print is . -Of 10 students sampled from a class of 200, 8 (80%) said they would like the school library to have longer hours.

Use the following information for questions: Assume the cholesterol level in a certain population has mean $\mu$ = 200 and standard deviation $\sigma$ = 24. The cholesterol levels for a random sample of n = 9 individuals are measured and the sample mean $\overline{x}$ is determined. -What is the z-score for a sample mean $\overline{x}$ = 212?

The sample mean number of accidents is to be calculated for both a sample of male and a sample of female drivers and we wish to determine the difference in sample means. Which statistic is being studied?

Which one of the following ways of collecting data would not result in paired data?

Use the following information for questions: Data collected over several years shows that of all patients that come to the doctor's office with a sore throat, 6% of them have a contagious virus. The next 188 patients with a sore throat are tested for this virus. -What is the probability that more than 11% of these 188 patients have the virus?

Use the following information for questions: In a pond with a large population of turtles, the percent of three different types of turtles are presented in the table below. 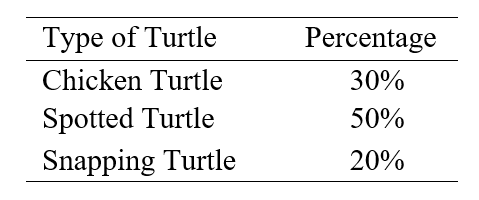A random sample of 100 turtles is taken from the pond. -What is the approximate probability that the sample proportion of spotted turtles falls between 45% and 55%?

Use the following information for questions: The level of nitrogen oxides (NOX) in the exhaust of a particular car model can be modeled by the normal distribution with mean 0.9 grams per mile and standard deviation of 0.16 grams per mile. A random sample of 4 of these NOX levels is to be selected. -What is the probability that the average NOX level of the 4 selected cars is greater than 1 gram per mile?

Use the following information for questions: A new study finds that frequent use of painkillers does not substantially increase a healthy man's risk of developing hypertension (high blood pressure). In other words, the proportion of healthy men with hypertension is the same for those who use painkillers frequently and those who don't. According to the American Heart Association, 1 in 3 American adults have hypertension. -Which of the following choices correctly denotes the difference between the proportions of men with hypertension (frequent use of painkillers - not frequent use of painkillers)?