Exam 6: Sampling and Sampling Distributions
Exam 1: Describing Data: Graphical247 Questions
Exam 2: Describing Data: Numerical326 Questions
Exam 3: Probability345 Questions
Exam 4: Discrete Random Variables and Probability Distributions257 Questions
Exam 5: Continuous Random Variables and Probability Distributions239 Questions
Exam 6: Sampling and Sampling Distributions147 Questions
Exam 7: Estimation: Single Population151 Questions
Exam 8: Estimation: Additional Topics109 Questions
Exam 9: Hypothesis Testing: Single Population164 Questions
Exam 10: Hypothesis Testing: Additional Topics103 Questions
Exam 11: Simple Regression217 Questions
Exam 12: Multiple Regression252 Questions
Exam 13: Additional Topics in Regression Analysis168 Questions
Exam 14: Analysis of Categorical Data241 Questions
Exam 15: Analysis of Variance192 Questions
Exam 16: Time-Series Analysis and Forecasting138 Questions
Exam 17: Additional Topics in Sampling110 Questions
Select questions type
A golfer practices 60 twenty-foot putts a day and historically makes 25 percent of them.Calculate the standard error of the sample proportion.
(Multiple Choice)
4.8/5  (41)
(41)
THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION:
Times spent studying by students in the week before final exams follow a normal distribution with standard deviation 9 hours.A random sample of 5 students was taken in order to estimate the mean study time for the population of all students.
-What is the probability that the sample mean is more than 3.2 hours below the population mean?
(Essay)
4.8/5  (40)
(40)
THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION:
It is estimated that 1.3% of all items produced on an assembly line have some form of defect.You randomly select 60 items for inspection.
-The probability is 86% that the proportion of items with a defect is less than what number?
(Essay)
4.9/5  (25)
(25)
THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION:
Let the random variable X follow a normal distribution with a mean of μ and a standard deviation of σ.Let  1 be the mean of a sample of 16 observations randomly chosen from this population,and
1 be the mean of a sample of 16 observations randomly chosen from this population,and  2 be the mean of a sample of 25 observations randomly chosen from the same population.
-Evaluate the statement P(
2 be the mean of a sample of 25 observations randomly chosen from the same population.
-Evaluate the statement P(  1 < μ +
1 < μ + 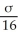 )< P(
)< P(  2 < μ +
2 < μ +  )as to whether it is true or false.Please state if you are unable to determine whether the statement is true or false.Support your answer.
)as to whether it is true or false.Please state if you are unable to determine whether the statement is true or false.Support your answer.
(Essay)
4.8/5  (32)
(32)
THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION:
Monthly rates of return on the shares of a particular common stock are independent of one another and normally distributed with a standard deviation of 1.8.A sample of 15 months is taken.
-Find the probability that the sample standard deviation is more than 1.2.
(Essay)
4.8/5  (33)
(33)
THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION:
It has been found that 62.1% of all unsolicited third class mail delivered to households goes unread.Over the course of a month,a household receives 100 pieces of unsolicited mail.
-What is the standard error of the sample proportion?
(Essay)
4.9/5  (32)
(32)
THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION:
A random sample of 10 stock market mutual funds was taken.Suppose that rates of returns on the population of stock market mutual funds follow a normal distribution.
-Suppose that a sample of 20 mutual funds had been taken.Without doing the calculations,indicate how this would change the 95% interval for the population variance when a random sample of 10 stock market mutual funds was taken.
(Essay)
4.9/5  (39)
(39)
If all possible samples of size n are drawn from an infinite population with a mean of 20 and a standard deviation of 5,then the standard error of the sampling distribution of sample means is equal to 1.0 only for samples of size:
(Multiple Choice)
4.7/5  (39)
(39)
The variance of the sampling distribution of the sample proportion is equal to P(1 - P)/ n.
(True/False)
4.7/5  (45)
(45)
As a general rule,the normal distribution is used to approximate the sampling distribution of the sample proportion only if:
(Multiple Choice)
5.0/5  (31)
(31)
THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION:
Candidates for employment at a city fire department are required to take a written aptitude test.Scores on this test are normally distributed with mean 260 and standard deviation 51.A random sample of nine test scores was taken.
-What are the mean and standard error of the sampling distribution for the mean score?
(Essay)
4.8/5  (36)
(36)
THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION:
Times spent studying by students in the week before final exams follow a normal distribution with standard deviation 9 hours.A random sample of 5 students was taken in order to estimate the mean study time for the population of all students.
-What is the standard error of the sampling distribution for the mean score?
(Essay)
4.8/5  (37)
(37)
THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION:
Candidates for employment at a city fire department are required to take a written aptitude test.Scores on this test are normally distributed with mean 260 and standard deviation 51.A random sample of nine test scores was taken.
-What is the probability that the sample mean score is less than 250?
(Essay)
4.7/5  (38)
(38)
The central limit theorem states that the sampling distribution of sample means will closely resemble the normal distribution regardless of the sample size.
(True/False)
4.9/5  (33)
(33)
THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION:
A sample of 25 bottles is taken from the production line at a local bottling plant.Assume that the fill amounts follow a normal distribution.
-What is the probability that the sample standard deviation is more than 70% of the population standard deviation?
(Essay)
4.8/5  (37)
(37)
THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION:
Each member of a random sample of 20 business economists was asked to predict the rate of inflation for the coming year.Assume that the predictions for the whole population of business economists follow a normal distribution with standard deviation 2%.
-Why does the sample size play such an important role in reducing the standard error of the mean? What are the implications of increasing the sample size?
(Essay)
4.7/5  (37)
(37)
THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION:
You have recently joined a Weight Watchers club.Suppose that the number of times you expect to visit the club in a month is represented by a normally distributed random variable with a mean of 12 and a standard deviation of 2.50.
-Over the course of the next year,what is the probability that you average more than 13 visits to the club?
(Multiple Choice)
4.7/5  (37)
(37)
THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION:
Given a population with mean μ=120 and variance σ2 = 81,the central limit applies when the sample size is large-enough.A random sample of size n = 36 is obtained.
-What are the mean and standard deviation of the sampling distribution for the sample means?
(Essay)
4.9/5  (33)
(33)
Random samples of size 36 are taken from an infinite population whose mean is 80 and standard deviation is 18.The standard error of the sample mean is:
(Multiple Choice)
4.8/5  (38)
(38)
THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION:
The length of time it takes to fill an order at a local sandwich shop is normally distributed with a mean of 4.1 minutes and a standard deviation of 1.3 minutes.
-The probability is 95% that the average waiting time for a random sample of ten customers is greater than how many minutes?
(Essay)
4.8/5  (30)
(30)
Showing 81 - 100 of 147
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)