Exam 6: Sampling and Sampling Distributions
Exam 1: Describing Data: Graphical247 Questions
Exam 2: Describing Data: Numerical326 Questions
Exam 3: Probability345 Questions
Exam 4: Discrete Random Variables and Probability Distributions257 Questions
Exam 5: Continuous Random Variables and Probability Distributions239 Questions
Exam 6: Sampling and Sampling Distributions147 Questions
Exam 7: Estimation: Single Population151 Questions
Exam 8: Estimation: Additional Topics109 Questions
Exam 9: Hypothesis Testing: Single Population164 Questions
Exam 10: Hypothesis Testing: Additional Topics103 Questions
Exam 11: Simple Regression217 Questions
Exam 12: Multiple Regression252 Questions
Exam 13: Additional Topics in Regression Analysis168 Questions
Exam 14: Analysis of Categorical Data241 Questions
Exam 15: Analysis of Variance192 Questions
Exam 16: Time-Series Analysis and Forecasting138 Questions
Exam 17: Additional Topics in Sampling110 Questions
Select questions type
THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION:
A random sample of size n = 25 is obtained from a normally distributed population with population mean μ = 200 and variance σ2 = 100.
-What is the value of the sample variance such that 5% of the sample variances would be greater than this value?
(Essay)
5.0/5  (40)
(40)
If the standard error of the sampling distribution of sample proportions is 0.0245 for samples of size 400,then the population proportion must be 0.40.
(True/False)
4.8/5  (34)
(34)
What is the name of the parameter that determines the shape of the chi-square distribution?
(Multiple Choice)
4.8/5  (32)
(32)
THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION:
A random sample of size n = 25 is obtained from a normally distributed population with population mean μ = 200 and variance σ2 = 100.
-What are the mean and standard deviation of the sampling distribution for the sample means?
(Essay)
4.7/5  (32)
(32)
THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION:
Suppose that 20% of all invoices are for amounts greater than $800.A random sample of 50 invoices is taken.
-What is the probability that more than 22.7% of these 50 invoices are for more than $800?
(Multiple Choice)
4.9/5  (37)
(37)
THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION:
In taking a sample of n observations from a population of N members,the variance of the sampling distribution of the sample means is 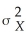 =
= 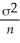
 .The quantity
.The quantity  is called the finite population correction factor.
-To get some feeling for possible magnitudes of the finite population correction factor,calculate it for samples of n = 15 observations from populations of N = 15,30,60,100,500,1,000,5,000,and 10,000 members.
is called the finite population correction factor.
-To get some feeling for possible magnitudes of the finite population correction factor,calculate it for samples of n = 15 observations from populations of N = 15,30,60,100,500,1,000,5,000,and 10,000 members.
(Essay)
4.9/5  (28)
(28)
THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION:
Suppose you flip a coin four times.For every head,you receive one point,and for every tail,you lose one point.
-Develop the sampling distribution for the mean number of points you receive from flipping four coins.
(Essay)
4.7/5  (36)
(36)
THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION:
Let the random variable X follow a normal distribution with a mean of μ and a standard deviation of σ.Let  1 be the mean of a sample of 16 observations randomly chosen from this population,and
1 be the mean of a sample of 16 observations randomly chosen from this population,and  2 be the mean of a sample of 25 observations randomly chosen from the same population.
-Suppose that 15% of all invoices are for amounts greater than $1,000.A random sample of 60 invoices is taken.What is the mean and standard error of the sample proportion of invoices with amounts in excess of $1,000? What is the probability that the proportion of invoices in the sample is greater than 18%?
2 be the mean of a sample of 25 observations randomly chosen from the same population.
-Suppose that 15% of all invoices are for amounts greater than $1,000.A random sample of 60 invoices is taken.What is the mean and standard error of the sample proportion of invoices with amounts in excess of $1,000? What is the probability that the proportion of invoices in the sample is greater than 18%?
(Essay)
5.0/5  (34)
(34)
THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION:
A random sample of 10 stock market mutual funds was taken.Suppose that rates of returns on the population of stock market mutual funds follow a normal distribution.
-The probability is 0.10 that sample variance is bigger than what percentage of the population variance?
(Essay)
4.7/5  (33)
(33)
The variance of the sampling distribution of sample mean  decreases as the sample size,n,increases.
decreases as the sample size,n,increases.
(True/False)
4.8/5  (34)
(34)
The central limit theorem can be applied to both discrete and continuous random variables.
(True/False)
4.8/5  (45)
(45)
THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION:
According to the Internal Revenue Service,80% of all tax returns lead to a refund.A random sample of 100 tax returns is taken.
-What is the probability that the sample proportion exceeds 0.85?
(Essay)
4.7/5  (32)
(32)
Without doing the calculations,state in which of the following ranges the sample mean selling price is most likely to lie:
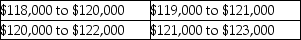
(Essay)
4.8/5  (29)
(29)
If the sample size,n,equals the population size,N,then the variance of the sample mean, 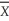 ,is zero.
,is zero.
(True/False)
4.7/5  (32)
(32)
THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION:
A sample of 25 bottles is taken from the production line at a local bottling plant.Assume that the fill amounts follow a normal distribution.
-The probability is 90% that the sample variance is less than what percent of the population variance?
(Essay)
4.7/5  (37)
(37)
All possible random samples of 200 middle managers are selected from a population for a study concerning their mean annual income.The population standard deviation is computed to be $2,248.5.What is the standard deviation of the sampling distribution of the means?
(Multiple Choice)
4.8/5  (29)
(29)
THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION:
Monthly rates of return on the shares of a particular common stock are independent of one another and normally distributed with a standard deviation of 1.8.A sample of 15 months is taken.
-Find the probability that the sample standard deviation is less than 2.6.
(Essay)
4.9/5  (37)
(37)
THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION:
The mean selling price of new homes in a city over a year was $120,000.The population standard deviation was $28,000.A random sample of 100 new home sales from this city was taken.
-What is the probability that the sample mean selling price was between $119,000 and $121,000?
(Essay)
4.9/5  (35)
(35)
The length of time it takes for a stock analyst to complete an evaluation of a company's earnings forecast is normally distributed with a standard deviation of 1.7 hours.From looking at the time spent by an analyst evaluating 15 randomly-selected stocks you find that the sample mean was 6.4 hours.If you know that the probability of getting a sample mean this large or larger is 0.36,what is the mean of the population?
(Essay)
4.8/5  (36)
(36)
Showing 101 - 120 of 147
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)