Exam 8: Continuous Probability Distributions
Exam 1: What Is Statistics46 Questions
Exam 2: Graphical Descriptive Techniques 194 Questions
Exam 3: Graphical Descriptive Techniques 2156 Questions
Exam 4: Numerical Descriptive Techniques275 Questions
Exam 5: Data Collection and Sampling84 Questions
Exam 6: Probability240 Questions
Exam 7: Random Variables and Discrete Probability Distributions283 Questions
Exam 8: Continuous Probability Distributions224 Questions
Exam 9: Sampling Distributions156 Questions
Exam 10: Introduction to Estimation154 Questions
Exam 11: Introduction to Hypothesis Testing189 Questions
Exam 12: Inference About a Population153 Questions
Exam 13: Inference About Comparing Two Populations170 Questions
Exam 14: Analysis of Variance157 Questions
Exam 15: Chi-Squared Tests179 Questions
Exam 16: Simple Linear Regression and Correlation304 Questions
Exam 17: Multiple Regression160 Questions
Exam 18: Model Building148 Questions
Exam 19: Nonparametric Statistics175 Questions
Exam 20: Time-Series Analytics and Forecasting225 Questions
Exam 21: Statistical Process Control140 Questions
Exam 22: Decision Analysis123 Questions
Exam 23: Conclusion47 Questions
Select questions type
Diet
Researchers studying the effects of a new diet found that the weight loss over a one-month period by those on the diet was normally distributed with a mean of 10 pounds and a standard deviation of 5 pounds.
-{Diet Narrative} If a dieter is selected at random,what is the probability that the dieter lost more than 7.5 pounds?
Free
(Short Answer)
4.9/5  (27)
(27)
Correct Answer:
0.6915
Which of the following has a mean and variance that depend on degrees of freedom?
Free
(Multiple Choice)
4.9/5  (23)
(23)
Correct Answer:
D
Let X be an exponential random variable with λ = 1.50.Find the following:
a.P(X ≥ 2)
b.P(X ≤ 4)
c.P(1 ≤ X ≤ 3)
d.P(X = 1)
Free
(Essay)
4.8/5  (35)
(35)
Correct Answer:
a. 0.0498 (note that f(x)= 1.50e−1.50x for x ≥ 0)
b. 0.9975
c. 0.2120
d. 0
Checking Accounts
A bank has determined that the monthly balances of the checking accounts of its customers are normally distributed with an average balance of $1,200 and a standard deviation of $250.
-{Checking Accounts Narrative} What proportion of customers have monthly balances more than $1,125?
(Short Answer)
4.7/5  (35)
(35)
You can use a continuous random variable to ____________________ a discrete random variable that takes on a countable,but very large,number of possible values.
(Short Answer)
4.9/5  (34)
(34)
The probability density function f(x)for a uniform random variable X defined over the interval [2,10] is
(Multiple Choice)
4.9/5  (35)
(35)
If we standardize the normal curve,we express the original X values in terms of their number of standard deviations away from the mean.
(True/False)
4.8/5  (36)
(36)
IT Graduates Salary
The recent average starting salary for new college graduates in IT systems is $47,500.Assume salaries are normally distributed with a standard deviation of $4,500.
-{IT Graduates Salary Narrative} What is the cutoff for the top 3% of the salaries?
(Short Answer)
5.0/5  (33)
(33)
We define 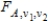 as the value of the F with v1 and v2 degrees of freedom such that the area to its right under the F curve is A,while
as the value of the F with v1 and v2 degrees of freedom such that the area to its right under the F curve is A,while 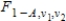 is defined as the value such that the area to its left is A.
is defined as the value such that the area to its left is A.
(True/False)
4.9/5  (29)
(29)
The value of an F distribution with v1 = 5 and v2 = 10 degrees of freedom such that the area to its left is 0.95 is 3.33.
(True/False)
4.9/5  (39)
(39)
Waiting Time
The length of time patients must wait to see a doctor at an emergency room in a large hospital has a uniform distribution between 40 minutes and 3 hours.
-{Waiting Time Narrative} What is the probability that a patient would have to wait no more than one hour?
(Short Answer)
4.8/5  (38)
(38)
Like that of the Student t distribution,the shape of the chi-squared distribution depends on its number of degrees of freedom.
(True/False)
4.8/5  (42)
(42)
Since there is an infinite number of values a continuous random variable can assume,the probability of each individual value is virtually 0.
(True/False)
4.9/5  (37)
(37)
Which of the following distributions can take on negative values?
(Multiple Choice)
4.9/5  (34)
(34)
Battery Life
A certain brand of batteries has a lifetime that has a normal distribution with a mean of 3,750 hours and a standard deviation of 300 hours.
-{Battery Life Narrative} What proportion of these batteries will last between 3,800 and 4,100 hours?
(Short Answer)
4.7/5  (41)
(41)
Repair Time
The time it takes a technician to fix a telephone problem is exponentially distributed with a mean of 15 minutes.
-{Repair Time Narrative} What is the probability that it will take a technician between 10 to 15 minutes to fix a telephone problem?
(Short Answer)
4.8/5  (39)
(39)
Showing 1 - 20 of 224
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)