Exam 18: Model Building
Exam 1: What Is Statistics46 Questions
Exam 2: Graphical Descriptive Techniques 194 Questions
Exam 3: Graphical Descriptive Techniques 2156 Questions
Exam 4: Numerical Descriptive Techniques275 Questions
Exam 5: Data Collection and Sampling84 Questions
Exam 6: Probability240 Questions
Exam 7: Random Variables and Discrete Probability Distributions283 Questions
Exam 8: Continuous Probability Distributions224 Questions
Exam 9: Sampling Distributions156 Questions
Exam 10: Introduction to Estimation154 Questions
Exam 11: Introduction to Hypothesis Testing189 Questions
Exam 12: Inference About a Population153 Questions
Exam 13: Inference About Comparing Two Populations170 Questions
Exam 14: Analysis of Variance157 Questions
Exam 15: Chi-Squared Tests179 Questions
Exam 16: Simple Linear Regression and Correlation304 Questions
Exam 17: Multiple Regression160 Questions
Exam 18: Model Building148 Questions
Exam 19: Nonparametric Statistics175 Questions
Exam 20: Time-Series Analytics and Forecasting225 Questions
Exam 21: Statistical Process Control140 Questions
Exam 22: Decision Analysis123 Questions
Exam 23: Conclusion47 Questions
Select questions type
For the following regression equation 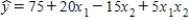 ,a unit increase in x2,while holding x1 constant at 1,changes the value of y on average by:
,a unit increase in x2,while holding x1 constant at 1,changes the value of y on average by:
Free
(Multiple Choice)
4.8/5  (34)
(34)
Correct Answer:
D
Motorcycle Fatalities
A traffic consultant has analyzed the factors that affect the number of motorcycle fatalities.She has come to the conclusion that two important variables are the number of motorcycle and the number of cars.She proposed the model 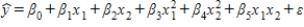 (the second-order model with interaction),where y = number of annual fatalities per county,x1 = number of motorcycles registered in the county (in 10,000),and x2 = number of cars registered in the county (in 1000).The computer output (based on a random sample of 35 counties)is shown below: THE REGRESSION EQUATION IS
(the second-order model with interaction),where y = number of annual fatalities per county,x1 = number of motorcycles registered in the county (in 10,000),and x2 = number of cars registered in the county (in 1000).The computer output (based on a random sample of 35 counties)is shown below: THE REGRESSION EQUATION IS 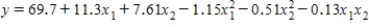
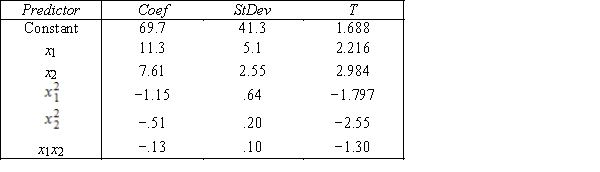 S = 15.2 R−Sq = 47.2% ANALYSIS OF VARIANCE
S = 15.2 R−Sq = 47.2% ANALYSIS OF VARIANCE 
-{Motorcycle Fatalities Narrative} Is there enough evidence at the 5% significance level to conclude that the model is useful in predicting the number of fatalities?
-{Motorcycle Fatalities Narrative} Is there enough evidence at the 5% significance level to conclude that the model is useful in predicting the number of fatalities?
Free
(Essay)
4.7/5  (29)
(29)
Correct Answer:
H0: β1 = β2 = β3 = β4 = β5 = 0 H0: At least one βi is not equal to zero Rejection region: F > F.05,5,29 = 2.55 Test statistic: F = 5.181 Conclusion: Reject the null hypothesis.Yes,the model is useful in predicting the number of fatalities.
A dummy variable is used as an independent variable in a regression model when:
Free
(Multiple Choice)
4.9/5  (29)
(29)
Correct Answer:
B
Motorcycle Fatalities
A traffic consultant has analyzed the factors that affect the number of motorcycle fatalities.She has come to the conclusion that two important variables are the number of motorcycle and the number of cars.She proposed the model 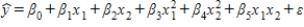 (the second-order model with interaction),where y = number of annual fatalities per county,x1 = number of motorcycles registered in the county (in 10,000),and x2 = number of cars registered in the county (in 1000).The computer output (based on a random sample of 35 counties)is shown below: THE REGRESSION EQUATION IS
(the second-order model with interaction),where y = number of annual fatalities per county,x1 = number of motorcycles registered in the county (in 10,000),and x2 = number of cars registered in the county (in 1000).The computer output (based on a random sample of 35 counties)is shown below: THE REGRESSION EQUATION IS 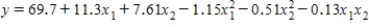
 S = 15.2 R−Sq = 47.2% ANALYSIS OF VARIANCE
S = 15.2 R−Sq = 47.2% ANALYSIS OF VARIANCE 
-{Motorcycle Fatalities Narrative} Test at the 1% significance level to determine if the x1 term should be retained in the model.
-{Motorcycle Fatalities Narrative} Test at the 1% significance level to determine if the x1 term should be retained in the model.
(Essay)
4.9/5  (32)
(32)
Incomes of Physicians
An economist is analyzing the incomes of physicians (general practitioners,surgeons,and psychiatrists).He realizes that an important factor is the number of years of experience.However,he wants to know if there are differences among the three professional groups.He takes a random sample of 125 physicians and estimates the multiple regression model y = β0 + β1x1 + β2x2 + β3x3 + ε,where y = annual income (in $1,000),x1 = years of experience,x2 = 1 if physician and 0 if not,and x3 = 1 if surgeons and 0 if not.The computer output is shown below. THE REGRESSION EQUATION IS y = 71.65 + 2.07x1 + 10.16x2− 7.44x3 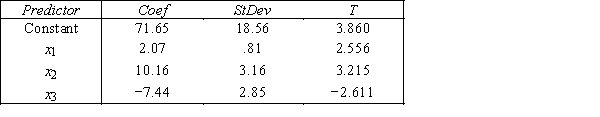 S = 42.6 R−Sq = 30.9% ANALYSIS OF VARIANCE
S = 42.6 R−Sq = 30.9% ANALYSIS OF VARIANCE 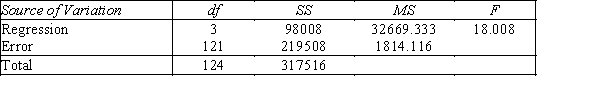
-{Incomes of Physicians Narrative} Estimate the annual income for a psychiatrist with 15 years of experience.
-{Incomes of Physicians Narrative} Estimate the annual income for a psychiatrist with 15 years of experience.
(Essay)
4.8/5  (29)
(29)
Because indicator variables represent different groups,t-tests on the indicator variables allow us to draw inferences about the ____________________ in y between the groups.
(Essay)
4.9/5  (35)
(35)
A first-order polynomial model with one predictor variable is the familiar simple linear regression model.
(True/False)
4.8/5  (35)
(35)
Suppose that the sample regression equation of a model is  .If we examine the relationship between y and x2 for x1 = 1,2,and 3,we observe that the three equations produced not only differ in the intercept term,but the coefficient of x2 also varies.
.If we examine the relationship between y and x2 for x1 = 1,2,and 3,we observe that the three equations produced not only differ in the intercept term,but the coefficient of x2 also varies.
(True/False)
4.8/5  (44)
(44)
In a first-order model with two predictors x1 and x2,an interaction term may be used when the:
(Multiple Choice)
4.8/5  (26)
(26)
In explaining the income earned by college graduates,which of the following independent variables is best represented by a dummy variable?
(Multiple Choice)
4.7/5  (44)
(44)
Hockey Teams
An avid hockey fan was in the process of examining the factors that determine the success or failure of hockey teams.He noticed that teams with many rookies and teams with many veterans seem to do quite poorly.To further analyze his beliefs he took a random sample of 20 teams and proposed a second-order model with one independent variable,average years of professional experience.The selected model is y = β0 + β1x + β2x2 + ε,where y = winning team's percentage,and x = average years of professional experience.The computer output is shown below. THE REGRESSION EQUATION IS y = 32.6 + 5.96x− .48x2  S = 16.1 R−Sq = 43.9% ANALYSIS OF VARIANCE
S = 16.1 R−Sq = 43.9% ANALYSIS OF VARIANCE 
-{Hockey Teams Narrative} Do these results allow us to conclude at the 5% significance level that the model is useful in predicting the team's winning percentage?
-{Hockey Teams Narrative} Do these results allow us to conclude at the 5% significance level that the model is useful in predicting the team's winning percentage?
(Essay)
4.9/5  (35)
(35)
It is not possible to incorporate nominal variables into a regression model.
(True/False)
5.0/5  (32)
(32)
The model 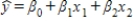 is used whenever the statistician believes that,on average,y is linearly related to x1 and x2 and the predictor variables do not interact.
is used whenever the statistician believes that,on average,y is linearly related to x1 and x2 and the predictor variables do not interact.
(True/False)
5.0/5  (47)
(47)
Which of the following statements about dummy variablesis false?
(Multiple Choice)
4.8/5  (29)
(29)
Suppose that the sample regression line of the first-order model is 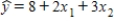 .If we examine the relationship between y and x1 for four different values of x2,we observe that the:
.If we examine the relationship between y and x1 for four different values of x2,we observe that the:
(Multiple Choice)
4.8/5  (35)
(35)
Another term for a first-order polynomial model is a regression ____________________.
(Short Answer)
4.7/5  (28)
(28)
To include a nominal variable in the regression model we need to transform it into:
(Multiple Choice)
4.8/5  (38)
(38)
____________________ regression is an iterative procedure that adds and deletes one independent variable at a time to/from the regression model.
(Short Answer)
4.9/5  (34)
(34)
The model y = β0 + β1x + β2x2 +.........+ βpxp + ε is referred to as a polynomial model with:
(Multiple Choice)
4.7/5  (27)
(27)
The model y = β0 + β1x1 + β2x2 + ε is a(n)____________________-order polynomial model with ____________________ predictor variable(s).
(Short Answer)
4.8/5  (36)
(36)
Showing 1 - 20 of 148
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)