Exam 14: Analysis of Variance
Exam 1: What Is Statistics46 Questions
Exam 2: Graphical Descriptive Techniques 194 Questions
Exam 3: Graphical Descriptive Techniques 2156 Questions
Exam 4: Numerical Descriptive Techniques275 Questions
Exam 5: Data Collection and Sampling84 Questions
Exam 6: Probability240 Questions
Exam 7: Random Variables and Discrete Probability Distributions283 Questions
Exam 8: Continuous Probability Distributions224 Questions
Exam 9: Sampling Distributions156 Questions
Exam 10: Introduction to Estimation154 Questions
Exam 11: Introduction to Hypothesis Testing189 Questions
Exam 12: Inference About a Population153 Questions
Exam 13: Inference About Comparing Two Populations170 Questions
Exam 14: Analysis of Variance157 Questions
Exam 15: Chi-Squared Tests179 Questions
Exam 16: Simple Linear Regression and Correlation304 Questions
Exam 17: Multiple Regression160 Questions
Exam 18: Model Building148 Questions
Exam 19: Nonparametric Statistics175 Questions
Exam 20: Time-Series Analytics and Forecasting225 Questions
Exam 21: Statistical Process Control140 Questions
Exam 22: Decision Analysis123 Questions
Exam 23: Conclusion47 Questions
Select questions type
Number of observation for particular combination of treatments is called a(n)________________.
Free
(Short Answer)
4.9/5  (27)
(27)
Correct Answer:
replicate
In a two-factor ANOVA,there are 4 levels for factor A,5 levels for factor B,and 3 observations for each combination of factor A and factor B levels.The total number of observations in this experiment equals:
Free
(Multiple Choice)
4.8/5  (31)
(31)
Correct Answer:
A
Motorcycle Repair Cost
Motorcycle insurance appraisers examine motorcycles that have been involved in accidental collisions and estimate the cost of repairs.An insurance executive claims that there are significant differences in the estimates from different appraisers.To support his claim he takes a random sample of six motorcycles that have recently been damaged in accidents.Three appraisers then estimate the repair costs of all six motorcycles.The data are shown below. 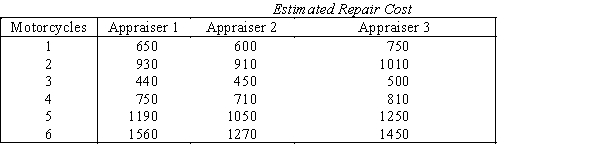
-{Motorcycle Repair Cost Narrative} Can we infer at the 5% significance level that the executive's claim is true?
-{Motorcycle Repair Cost Narrative} Can we infer at the 5% significance level that the executive's claim is true?
Free
(Essay)
4.7/5  (31)
(31)
Correct Answer:
H0: μ1 = μ2 = μ3 vs.H1: At least two means differ Conclusion: Reject the null hypothesis.Yes,the insurance executive's claim is true,according to this data.
We can use the F-test to determine whether μ1 is greater than μ2.
(True/False)
4.9/5  (28)
(28)
The sum of squares for treatments,SST,achieves its smallest value (zero)when all the sample means are equal.
(True/False)
4.9/5  (40)
(40)
In one-way ANOVA,the test statistic is defined as the ratio of the mean square for error (MSE)and the mean square for treatments (MST),namely,F = MSE / MST.
(True/False)
4.7/5  (39)
(39)
A randomized block design with 4 treatments and 5 blocks produced the following sum of squares values: SS(Total)= 2000,SST = 400,SSE = 200.The value of MSB must be 350.
(True/False)
4.9/5  (40)
(40)
In the two-factor ANOVA where a is the number of factor A levels,b is the number of factor B levels,and r is the number of replicates,the number of degrees of freedom for interaction is:
(Multiple Choice)
4.7/5  (30)
(30)
GMAT Scores
A recent college graduate is in the process of deciding which one of three graduate schools he should apply to.He decides to judge the quality of the schools on the basis of the Graduate Management Admission Test (GMAT)scores of those who are accepted into the school.A random sample of six students in each school produced the following GMAT scores.Assume that the data are normally distributed with equal variances for each school. 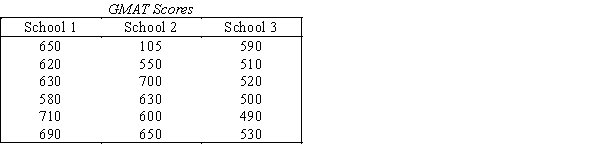
-{GMAT Scores Narrative} Can he infer at the 10% significance level that the GMAT scores differ among the three schools?
-{GMAT Scores Narrative} Can he infer at the 10% significance level that the GMAT scores differ among the three schools?
(Essay)
4.8/5  (37)
(37)
In a one-way ANOVA,error variability is computed as the sum of the squared errors,SSE,for all values of the response variable.This variability is the:
(Multiple Choice)
4.9/5  (42)
(42)
Number of observation for a particular combination of treatments is called a replicate.
(True/False)
4.9/5  (38)
(38)
The distribution of the test statistic for analysis of variance is the:
(Multiple Choice)
4.8/5  (40)
(40)
LSAT Scores
A recent college graduate is in the process of deciding which one of three Law schools he should apply to.He decides to judge the quality of the schools on the basis of the Law School Admission Test (LSAT)scores of those who are accepted into the school.A random sample of six students in each school produced the following LSAT scores.An F-test using ANOVA concluded that average LSAT scores differ for at least two of the schools. 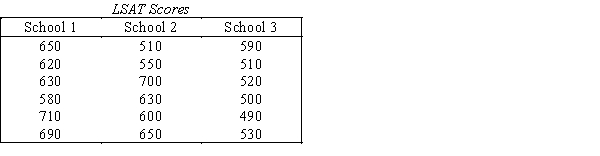
-{LSAT Scores Narrative} Use Tukey's method with α = 0.05 to determine which population means differ.
-{LSAT Scores Narrative} Use Tukey's method with α = 0.05 to determine which population means differ.
(Essay)
4.8/5  (35)
(35)
Which of the following components in an ANOVA table is not additive?
(Multiple Choice)
4.8/5  (36)
(36)
The alternative hypothesis of ANOVA is that ____________________ population means are different.
(Short Answer)
4.8/5  (29)
(29)
In one-way ANOVA,the amount of total variation that is unexplained is measured by the:
(Multiple Choice)
4.7/5  (40)
(40)
The test statistic in the analysis of variance (ANOVA)technique follows a normal distribution.
(True/False)
4.8/5  (39)
(39)
In ANOVA the populations are classified according to one or more criterion,called ____________________.
(Short Answer)
4.9/5  (30)
(30)
In a two-factor ANOVA,always test for ____________________ first.
(Short Answer)
4.8/5  (32)
(32)
TV News Viewing Habits
A statistician employed by a television rating service wanted to determine if there were differences in television viewing habits among three different cities in New York.She took a random sample of five adults in each of the cities and asked each to report the number of hours spent watching television in the previous week.The results are shown below. (Assume normal distributions with equal variances.) 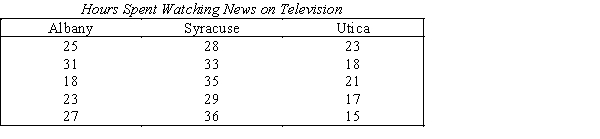
-{TV News Viewing Habits Narrative} Set up the ANOVA Table.Use α = 0.05 to determine the critical value.
-{TV News Viewing Habits Narrative} Set up the ANOVA Table.Use α = 0.05 to determine the critical value.
(Essay)
4.8/5  (43)
(43)
Showing 1 - 20 of 157
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)